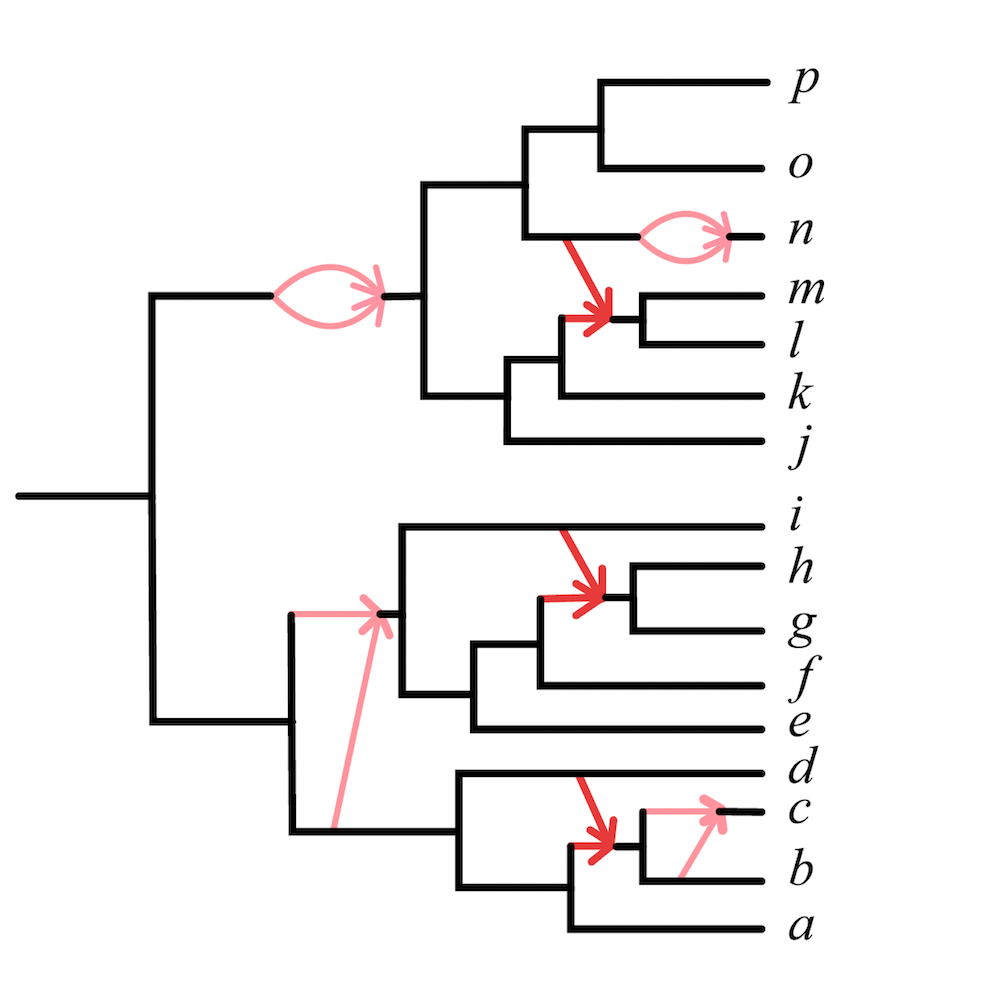
Practical Inference Algorithms for Species Networks
Phylogenetics is a field of evolutionary biology dedicated to understanding the evolutionary relationships among species. Inferring these relationships is essential for diverse applications, including conservation efforts, tracking infectious disease dynamics, and improving agricultural practices.
Understanding Moneyless Markets
Economists are using mathematics to come to a deeper understanding of how to allocate indivisible goods in markets that do not use currency.
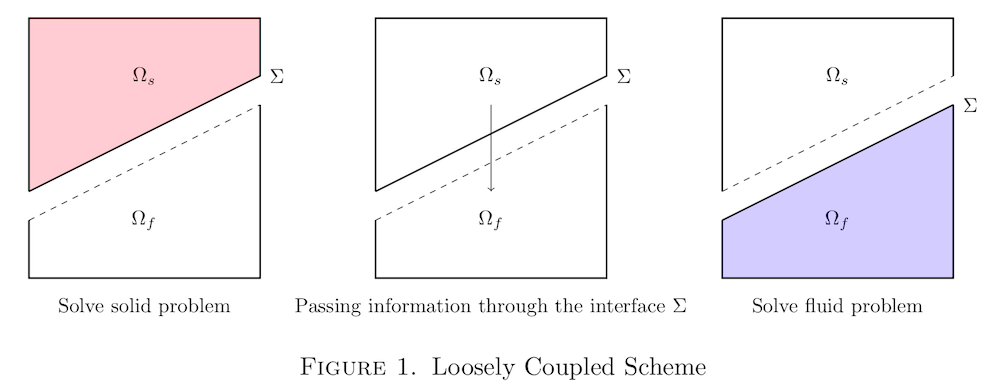
A Second-Order Correction Method For A Parabolic-Parabolic Interface Problem
Fluid-structure interaction (FSI) problems involve the study of how fluids and solid structures behave when they come into contact with each other. These problems are important in many engineering and scientific applications, such as aeronautics, aeroelasticity, aerodynamics, biomechanics, civil engineering, and mechanical engineering. Sijing Liu introduces a second-order correction method for a parabolic-parabolic interface problem, a simplified version of FSI.
Grothendieck Shenanigans: Permutons From Pipe Dreams via Integrable Probability
If Alice and Bob each take a walk, a random one, what is the chance they will meet? If n people walk randomly in Manhattan from the Upper East Side going south or west, how often will two of them meet until they reach the Hudson? If they do not want to see each other more than once, in what relative order will they most likely arrive at the Hudson shore? If we make a rhombus like an Aztec diamond from 2 by 1 dominoes, what would it most likely look like? If water molecules arrange themselves on a square grid, what angles between the hydrogen atoms will be formed near the boundaries? And if ribosomes are transcribing the mRNA, how do they hop between the sites (codons)?
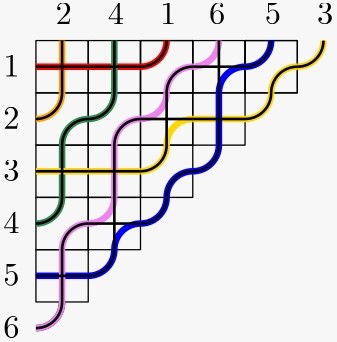
Algorithms, Fairness, and Equity
Political districting is an important and mathematically challenging problem. In fall 2023, the Simons Laufer Mathematical Sciences Institute (SLMath) hosted a semester program on algorithms, fairness, and equity, focusing on the intersection of computational tools and the many notions of fairness that arise in different mathematical and societal contexts, such as political districting.
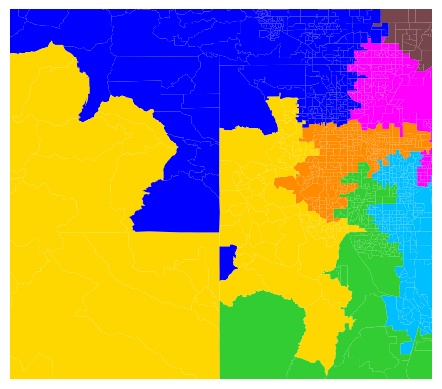
Exploiting Combinatorial Regularity for Topological and Morse Measures of ReLU Neural Networks
Identifying the capabilities of a machine learning model before applying it is a key goal in neural architecture search. Too small of a model, and the network will never successfully perform its task. Too large of a model, and computational energy is wasted and the cost of model training may become unbearably high. While universal approximation theorems and even dynamics are available for various limiting forms of ReLU neural networks, there are still many questions about the limitations of what small neural networks of this form can accomplish. Marissa Masden seeks to understand ReLU neural networks through a combinatorial, discrete structure that enables capturing topological invariants of structures like the decision boundary.
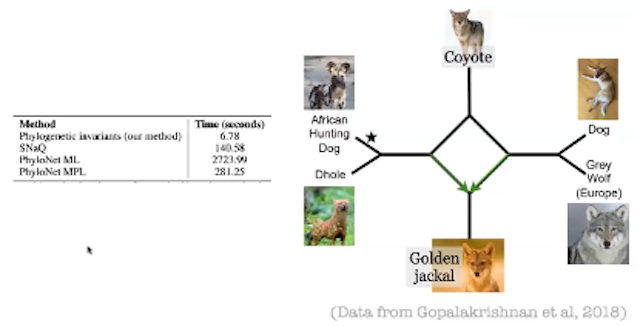
Our Changing World: Algebraic Statistics for Evolutionary Biology and Ecology
Understanding the evolutionary history of a collection of species, through fields such as phylogenomics and comparative phylogenetics, is crucial as we consider the future effects of climate change. Algebraic statistics provides algebraic and geometric tools to study the models commonly used in evolutionary biology. The Institute for Mathematical and Statistical Innovation (IMSI), hosted a workshop, “Algebraic Statistics for Ecological and Biological Systems,” as part of a Long Program on “Algebraic Statistics and Our Changing World,” which highlighted these connections.
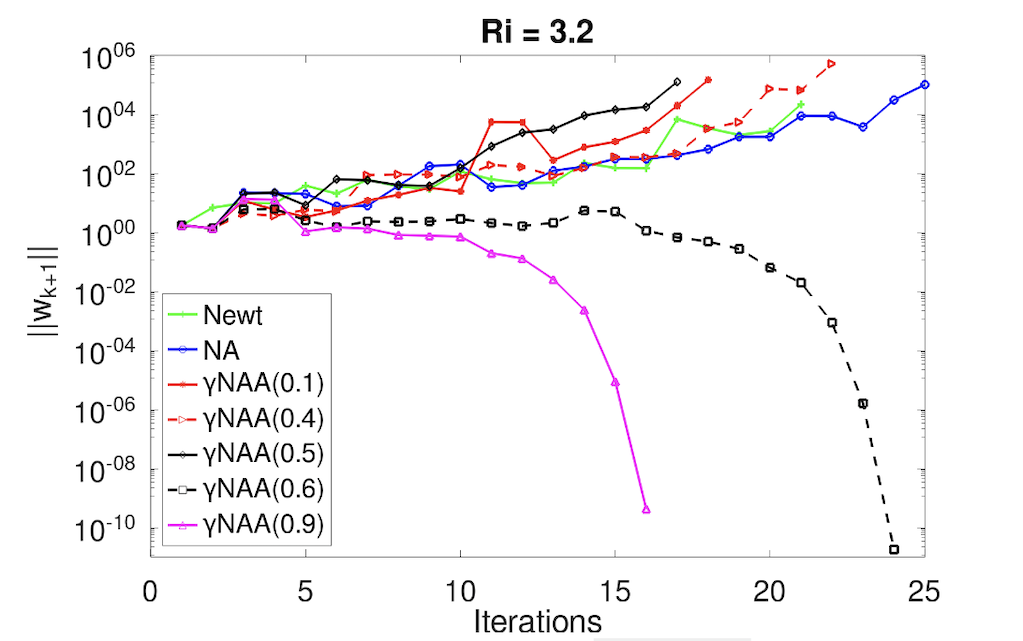
Improving Newton’s Method Near Singular Points with Anderson Acceleration
Nonlinear equations are ubiquitous in the sciences, a famous example being the Navier-Stokes equations in fluid mechanics. To compute a numerical solution for a given nonlinear equation, one often employs an iterative scheme such as Newton’s method. Recent works by Matt Dallas, Sara Pollock, and Leo Rebholz analyze Anderson accelerated Newton’s method applied to singular problems.
Euler Systems
In the mid-19th century, while attempting to prove Fermat’s last theorem, German mathematician Ernst Kummer started investigating arithmetic on novel number systems.
Traffic and Theory: Waves on Roads and Blackboards
The largest live autonomous vehicle traffic experiment ever conducted began the week of November 18, 2022, in Nashville, Tennessee. It involved 100 cars and a workforce of more than 250, around 70 of whom were researchers. One of the goals of the experiment was to analyze how level two autonomous vehicles (think cruise control with a couple added functions, like speed adjustment that uses LIDAR) can impact traffic waves, specifically those representing frustrating “stop and go” conditions...