
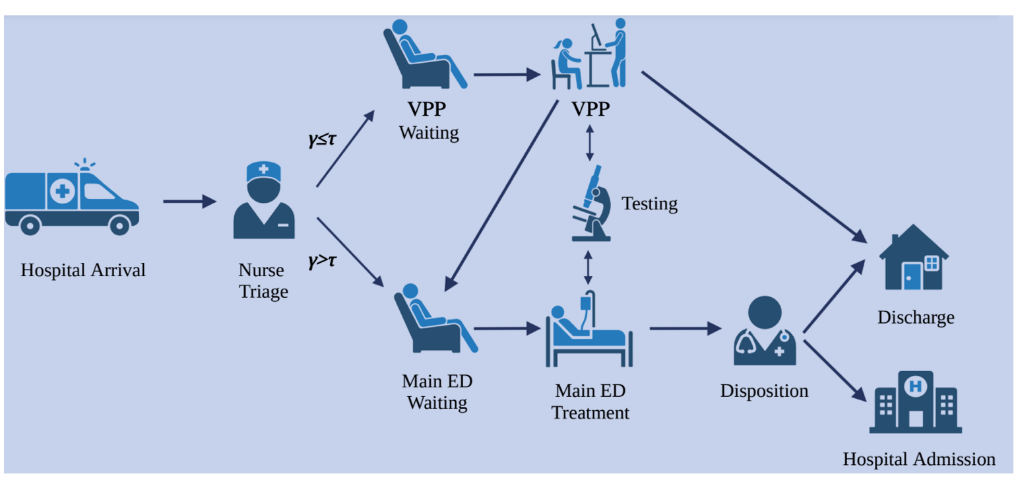
Managing Hospital Emergency Rooms
The quantification of medical and health care has revolutionized human lives, with strong and long-lasting positive social and economic impact. This revolution stems from synergies among mathematics, statistics, data science, medicine, and machine learning (ML) and artificial intelligence (AI) which has prompted the creation of interdisciplinary areas across the various fields. Despite major progress in many scientific directions, there is a continuing need to develop existing areas and set the foundations for new ones. This need has been amplified by emergent events such as the recent COVID-19 pandemic.

The Promise of Computer-Assisted Mathematics
Many mathematicians predict that one of the most important mathematical stories in the 21st century will be the changing role of computers in assisting mathematicians in proving theorems, or even in proving theorems themselves. UCLA professor Terence Tao highlighted some of the history and potential future of machine-assisted mathematics at the 40th anniversary symposium for MSRI/SLMath held in April 2023.


Machine Assisted Proofs
(By Jordan Ellenberg) In February of 2023, IPAM hosted a workshop on Machine-Assisted Proof. This program had been in the works for more than a year, but ended up being held at a timely moment, when unexpectedly rapid development in large language models (LLMs) in late 2022 had brought focused national attention to the question of how machines could assist or even replace human ingenuity.

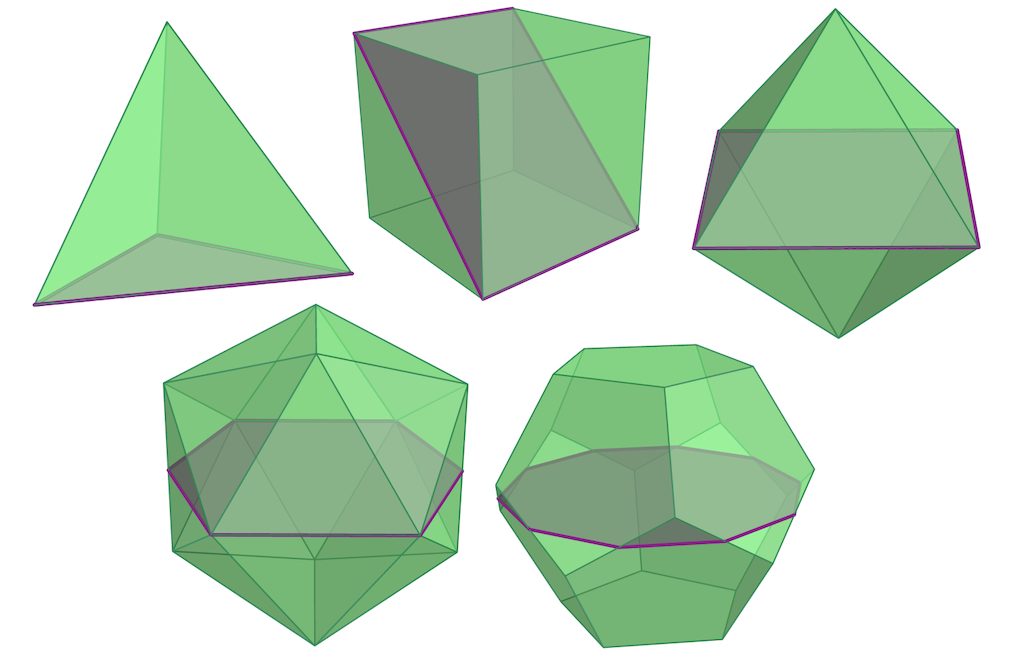
Slices of Polytopes
Given a 3-dimensional cube, the intersection with an affine hyperplane is always a polygon with 3,4,5, or 6 vertices. But how can one understand the slices of a general polytope? And which slice is "the best," e.g., is the slice of maximal volume?

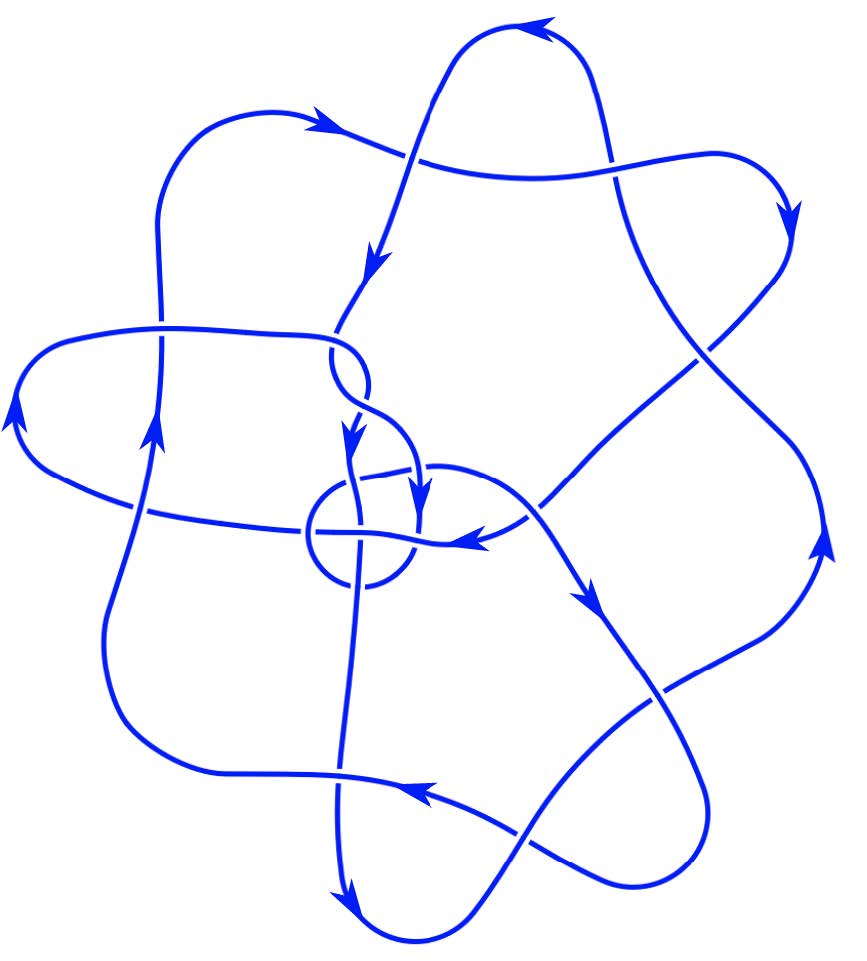
Khovanov Homology of Positive Knots
While there exists a theoretical algorithm that decides in finite time if a given knot is positive or not, this algorithm has a factorial run time and is thus a purely theoretical result that is not working in practice even for very simple knots. In their project, Marc Kegel and Marithania Silvero have developed a different approach to obstruct positivity of knots.

Modularity of Generating Series
Jan Bruinier, Benjamin Howard, Stephen S. Kudla, Michael Rapoport, and Tonghai Yang have a breakthrough result on the modularity of generating series of divisors on unitary Shimura varieties, published in two parts as a monograph in 2020 in Astérisque.
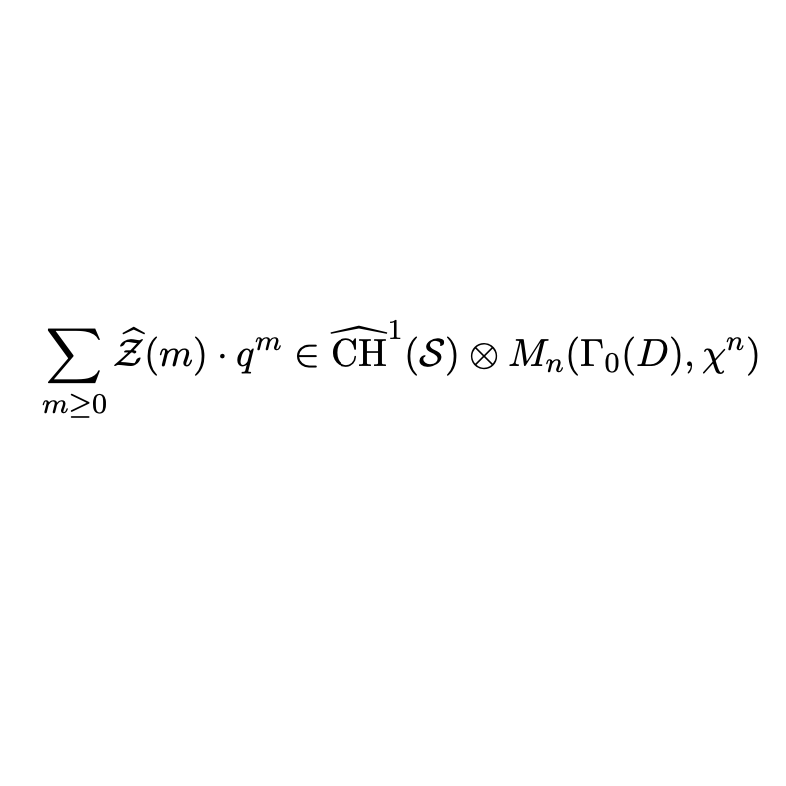

Novel use of ergodic theory leads to breakthrough solution of conjectures of Erdős about sumsets
Mathematicians build on Furstenberg’s correspondence principle to transfer the combinatorial problem to a dynamical one

Applying computational and data science to social justice issues requires a lot of “non-mathematical work”
In order to develop new mathematical techniques to explore and address issues related to social justice, ironically, mathematicians need to do a lot of work considered by many to be “non-mathematical.”


Optimal Transport and a Unified Perspective on Set Dualities
Optimal transport is a powerful framework, used by mathematicians and economists, that studies the allocation of resources. The origin of the subject goes back to the engineer Gaspard Monge, who was interested in the problem of the optimal way of redistributing mass: given a pile of soil, how can it be transported and reshaped to form an embankment with minimal effort? Originally, the cost function of transportation was simply the distance the particles were moved, but as the world evolved, scientists became interested in other, often abstract, cost functions.


White Paper: Computational Microscopy
For more than three centuries, lens-based microscopy, such as optical, phase-contrast, fluorescence, confocal, and electron microscopy, has played an important role in the evolution of modern science and technology.