Traffic and Theory: Waves on Roads and Blackboards
The largest live autonomous vehicle traffic experiment ever conducted began the week of November 18, 2022, in Nashville, Tennessee. It involved 100 cars and a workforce of more than 250, around 70 of whom were researchers. One of the goals of the experiment was to analyze how level two autonomous vehicles (think cruise control with a couple added functions, like speed adjustment that uses LIDAR) can impact traffic waves, specifically those representing frustrating “stop and go” conditions...
Higher uniformity of bounded multiplicative functions
Kaisa Matomäki, Maksym Radziwill, Terence Tao, Joni Teräväinen, and Tamar Ziegler make progress on the conjectures of Sarnak and Chowla with their paper “Higher uniformity of bounded multiplicative functions in short intervals on average” published in the Annals of Mathematics in 2023. The work originated in a working group at the AIM workshop “Sarnak's conjecture” in December 2018.
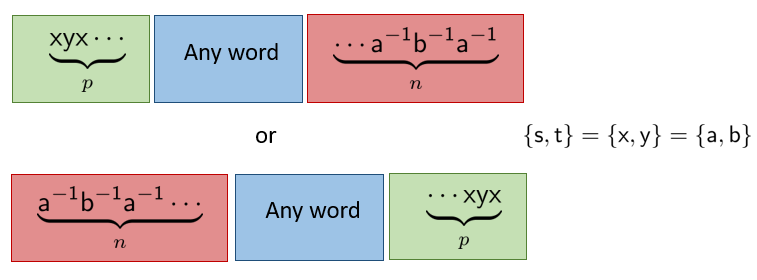
Giving a Quadratic Solution to the Word Problem in 3-free Artin Groups
Artin groups emerged in the 1960s as generalizations of braid groups and modifications of Coxeter reflection groups involving the removal of the involution relation inherent in reflections, thus creating groups that project onto Coxeter groups. For example, braid groups with n strands project onto the symmetric group of n elements. Therefore, Artin groups have a very simple presentation: they are groups generated by a finite set of generators S and relations written as sts · · · = tst . . . for s, t ∈ S, where both words in the equality have the same length. However, groups so simple to define do not admit many common techniques that allow obtaining global results for any Artin group, making them highly enigmatic groups.
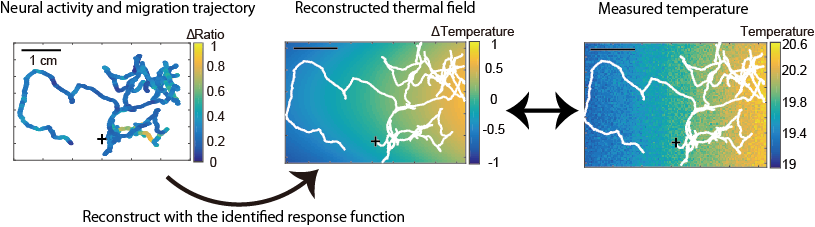
Decoding Neural Representation of Navigation by Mathematical Modeling and Quantitative Data
The navigational behavior of an animal consists of several steps of information processing, including the detection of the surrounding environment, integration of multi-modal sensation, and generation of actions. Whole steps are computed in a neural circuit, or a group of interconnected neurons that work together to carry out a specific function. Understanding how each navigation process is implemented in a neural circuit is a big challenge in neuroscience. Because ensembles of the neural dynamics are only partially observed due to technical limitations, a combination of theoretical and experimental approaches is necessary to tackle this challenge.
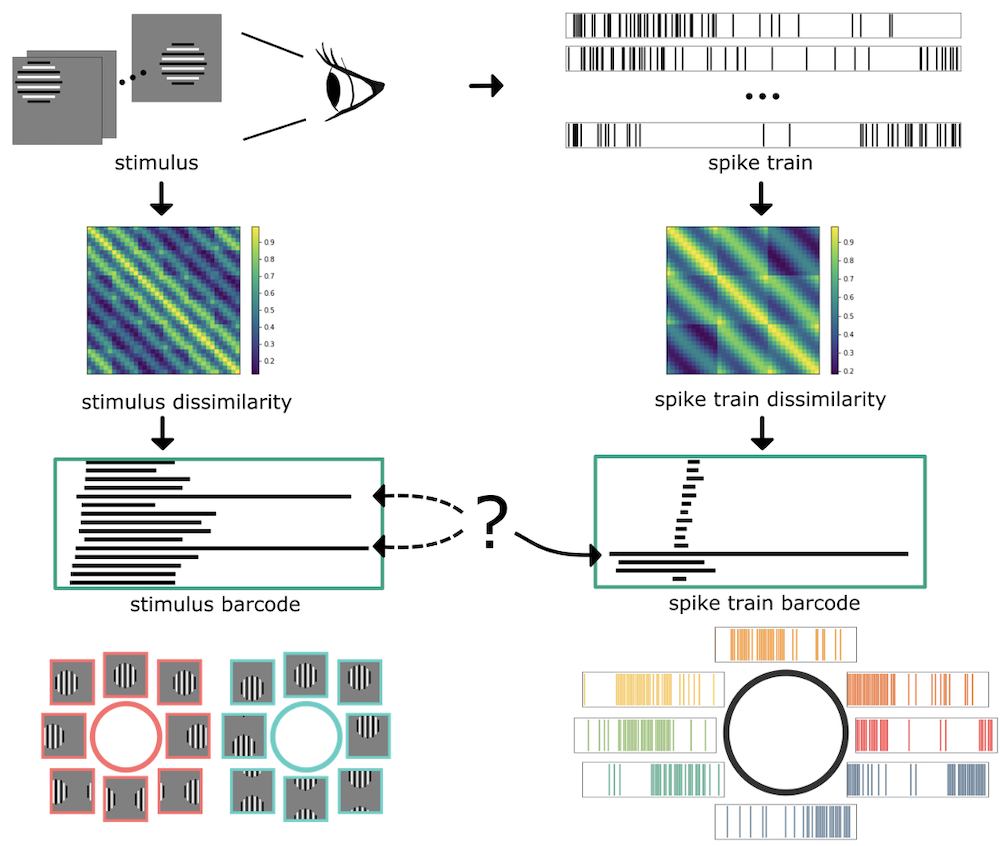
Interpreting the Shape of Neural Activity
An animal’s brain encodes representations of important aspects of the world and its own internal state through its constituent neurons. This information is distributed across many different anatomical regions in the brain, each of which performs computations and passes the results to others, with the end result producing behavior. The foundational observation in topological data analysis, or the study of datasets’ geometric features, is that the coarse, mesoscale structure of data can be described in terms of circles, spheres, tori, and a galaxy of more esoteric objects.
Connections in Gauge Theory
Inspired by the rise of mirror symmetry in theoretical physics, Jason Lotay is investigating connections between mathematical objects called calibrated submanifolds and instantons.
Building Bridges with Floer Homotopy Theory
It started with a walk. Lisa Traynor and Hiro Lee Tanaka liked to hike through the fire trails near the Simons Laufer Mathematical Sciences Institute (SLMath) during their lunch hours. Both were participants in the Floer homotopy theory semester program but came to the program from different edges of the field. Traynor, a professor at Bryn Mawr College, specializes in symplectic and contact structures on manifolds, while Tanaka of Texas State University focuses on homotopy theory. But eventually their walks became a path to a joint research project.
From Soap Bubbles to Singularities: Exploring the Principle of Geometric Minimization
Imagine that you are blowing a soap bubble using a simple children’s wand. Why does the bubble that you create take the form of a sphere? Why not an ovoid? Or a cylindrical shape? Or something totally random? An answer is provided by the concept of “minimization.” In nature, certain phenomena tend toward minimization, meaning that they seem to “want” to take a path that uses minimal energy to return to an equilibrium state, or, in the case of the bubble, form a shape that has the least surface area possible within the system’s required constraints.
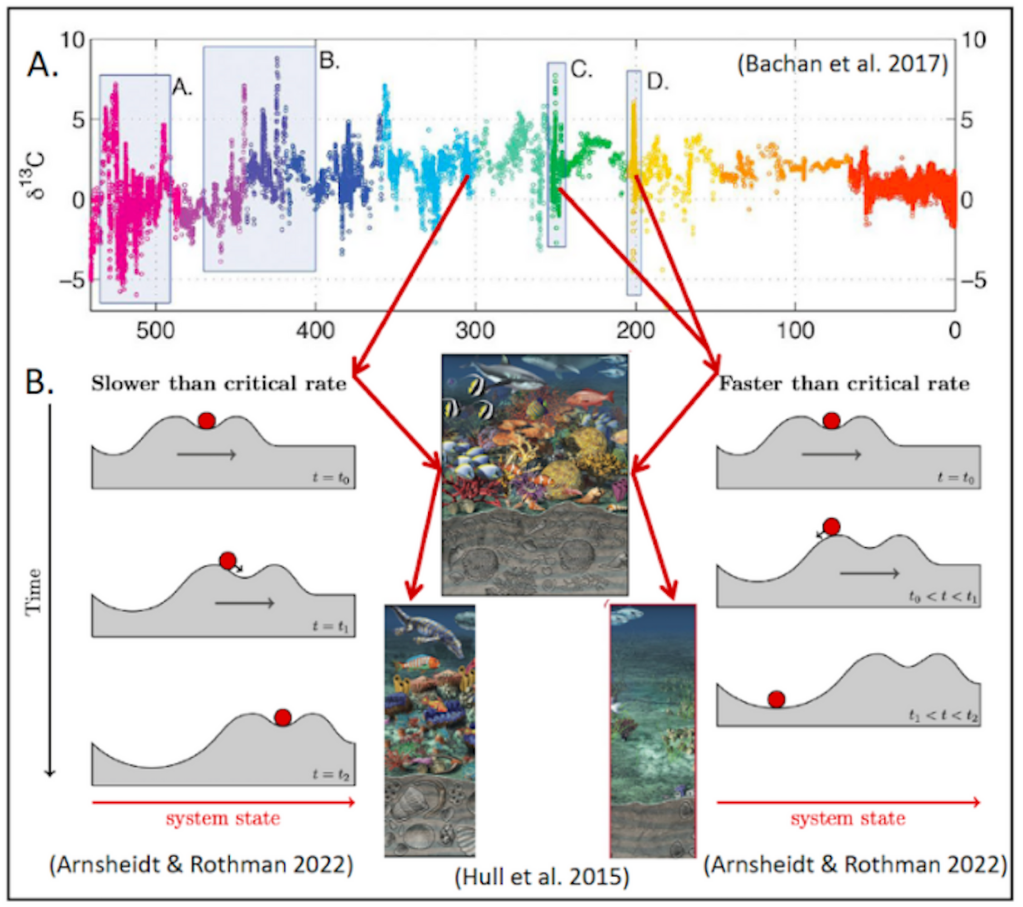
Climate Tipping Phenomena in Nonautonomous Paleoecosystems
IMSI hosted an Interdisciplinary Research Cluster entitled, “Climate Tipping Phenomena in Nonautonomous Paleoecosystems,” June 20-30, 2023, at the University of Chicago. The IRC focused on developing a framework for describing tipping points and regime shifts in a coupled global climate-biota system taking into account the temporal heterogeneity of the processes involved. The focus was the origin and timing of potential extinction triggers. There is evidence in the fossil record that the global ecosystems’ response to a trigger (i.e., increases in CO2) can be diverse, leading to a considerable biodiversity loss (i.e., a mass extinction) in some cases but having a relatively small effect in others.
Managing Hospital Emergency Rooms
The quantification of medical and health care has revolutionized human lives, with strong and long-lasting positive social and economic impact. This revolution stems from synergies among mathematics, statistics, data science, medicine, and machine learning (ML) and artificial intelligence (AI) which has prompted the creation of interdisciplinary areas across the various fields. Despite major progress in many scientific directions, there is a continuing need to develop existing areas and set the foundations for new ones. This need has been amplified by emergent events such as the recent COVID-19 pandemic.
