by Sijing Liu

Fluid-structure interaction (FSI) problems involve the study of how fluids and solid structures behave when they come into contact with each other. These problems are important in many engineering and scientific applications, such as aeronautics, aeroelasticity, aerodynamics, biomechanics, civil engineering, and mechanical engineering. 

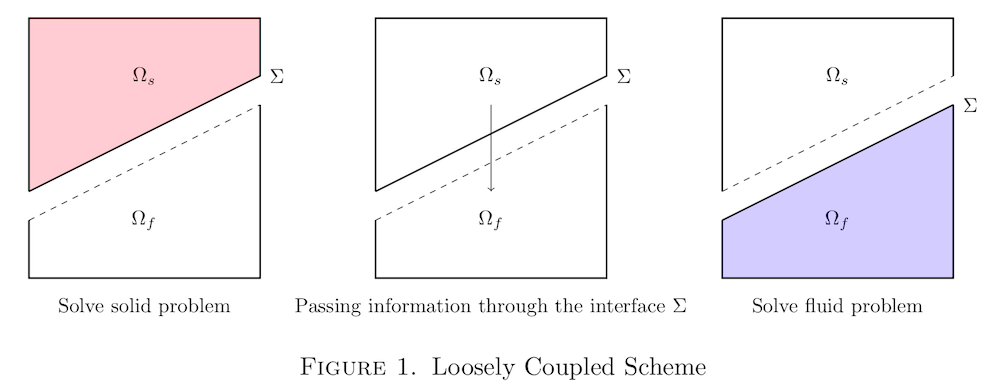
The computational solution of fluid-structure interaction problems remains a challenging problem, especially how to computationally couple the two sub-problems. One popular approach is the loosely coupled scheme, where the solid and fluid systems are solved sequentially. Using this method, information is passed across the coupling interface at discrete time levels with no iteration between the sub-systems within one time-step. See Figure 1 for an illustration of a loosely coupled scheme. Here, \(\Omega_s\) and \(\Omega_f\) represent two subdomains that interact through an interface \(\Sigma\). A solid problem is defined in \(\Omega_s\), while a fluid problem is defined in \(\Omega_f\), with the interaction governed by coupling conditions at \(\Sigma\).

Numerous studies in the literature focus on loosely coupled schemes for incompressible FSI with thick-walled solids. In particular, Robin-Robin loosely coupled schemes have recently been proposed and analyzed [3, 5], demonstrating unconditional stability and, for the first time, a spatially uniform splitting error. The term Robin-Robin refers to the use of Robin-type boundary conditions applied at the interface for both subproblems.

In our recent paper “Estimates of discrete time derivatives for the parabolic-parabolic Robin-Robin coupling method,” we consider a parabolic-parabolic interface problem, a simplified version of FSI. See Figure 2 for an illustration. In each subdomain, a simple heat equation is defined, with the two problems coupled by boundary conditions at the interface \(\Sigma\). It is known that Robin-Robin loosely coupled schemes for parabolic-parabolic interface problems exhibit first-order convergence in time [8, 5]. However, there seems to be a lack of analysis on high-order methods for FSI problems or parabolic-parabolic problems. To the best of our knowledge, there are no existing proofs of second-order accuracy for the defect-correction method applied to PDE interface problems. Our goal is to address this gap by proving second-order convergence of a prediction-correction method with a single correction step (see [6]). This opens the door to more high-order convergent methods and provides insights into the analysis of FSI and other real-world applications.

By leveraging the good stability properties and spatially uniform splitting error of the method in [5, 7], we aim to construct a higher-order scheme in time by building on the prediction-correction methodology [1], with the Robin-Robin loosely coupled method as the prediction step. The guiding principle of our correction method is to have discrete time differences of the prediction step error appearing on the right-hand side of the correction step error equations. Then, one uses the fact that these discrete time differences converge with second-order accuracy (see [4]). We have rigorously proved that our defect-correction methods achieve second-order accuracy in time for a specific case with a horizontal interface. Additionally, numerical results indicate second-order convergence in more general scenarios. We refer to [6, 4] for more details.

Sijing Liu was an Institute Postdoctoral Fellow at ICERM during the Fall 2023 semester and the Spring 2024 Numerical PDEs: Analysis, Algorithms, and Data Challenges program. He focused on this problem during his fellowship, collaborating with Drs. Erik Burman, Miguel A. Fernández, Rebecca Durst And Johnny Guzmán. Additionally, he worked on projects concerning convection-dominated problems and optimal control problems, leading to the submission of four manuscripts [4, 9, 2, 6].

References

[1] K. Böhmer, P. W. Hemker, and H. J. Stetter. The defect correction approach. Defect Correction Methods: Theory and Applications, 1–32, 1984. 

[2] S. B. Boyana, T. Lewis, S. Liu, and Y. Zhang. Convergence analysis of novel discontinuous Galerkin methods for a convection dominated problem. Computers & Mathematics with Applications, 175:224-235, 2024.

[3] E. Burman, R. Durst, M. Fernández, and J. Guzmán. Loosely coupled, non-iterative time-splitting scheme based on Robin–Robin coupling: Unified analysis for parabolic/parabolic and parabolic/hyperbolic problems. Journal of Numerical Mathematics, 31(1):59–77, 2023.

[4] E. Burman, R. Durst, M. Fernández, J. Guzmán, and S. Liu. Estimates of discrete time derivatives for the parabolic parabolic Robin-Robin coupling method. Numerical Algorithms, 2024. DOI: 10.1007/s11075-024-01902-z. 

[5] E. Burman, R. Durst, M. A. Fernández, and J. Guzmán. Fully discrete loosely coupled Robin-Robin scheme for incompressible fluid-structure interaction: stability and error analysis. Numerische Mathematik, 151(4):807–840, 2022. 

[6] E. Burman, R. Durst, M. A. Fernández, J. Guzmán, and S. Liu. A second-order correction method for loosely coupled discretizations applied to parabolic-parabolic interface problems. IMA Journal of Numerical Analysis, 2024. 

[7] E. Burman, R. Durst, M. A. Fernández, J. Guzmán, and O. Ruz. Robin-Robin loose coupling for incompressible fluid-structure interaction: non-linear setting and nearly-optimal error analysis. 2023. https://inria.hal.science/hal 04258861. 

[8] E. Burman, R. Durst, and J. Guzmán. Stability and error analysis of a splitting method using Robin–Robin coupling applied to a fluid-structure interaction problem. Numerical Methods for Partial Differential Equations, 38(5):1396– 1406, 2022. 

[9] S. Liu and V. Simoncini. Multigrid preconditioning for discontinuous Galerkin discretizations of an elliptic optimal control problem with a convection-dominated state equation. Journal of Scientific Computing, 101(Article 79), 2024. 

Contacts

Sijing Liu, Department of Mathematical Sciences, Worcester Polytechnic Institute, Worcester, MA 01609, USA 

Erik Burman, Department of Mathematics, University College London, London, UKWC1E 6BT, United Kingdom 

Rebecca Durst, Argonne National Laboratory, 9700 S. Cass Avenue, Lemont, IL 60439, USA 

Miguel A. Fernandez, Sorbonne Université & CNRS, UMR 7598 LJLL, 75005 Paris France – Inria, 75012  Paris, France 

Johnny Guzman, Division of Applied Mathematics, Brown University, 182 George Street, Providence, RI 02912, USA