
Big Data meets Number Theory
Researchers from ICERM’s special semester “Computational Aspects of the Langlands Program” are creating new data-driven models for collaborative research in number theory, culminating in the May 10, 2016 official release of the L-functions and Modular Forms Database (LMFDB) at www.lmfdb.org. Computation is not new to number theory – in Babylon huge tablets of sines and cosines were created and...


Identifying Links Between the S&P500 and VIX Derivatives
By Andrew Papanicolaou The technique of volatility trading has been common practice since the 1970’s. Typically, a long (short) position in volatility included a long (short) position in options. In 2003 there came a more standardized way of trading volatility, as the VIX formula became the universally-accepted predictor of volatility. The VIX is a 30-day predictor of volatility given by a...

Overcoming the Curse of Dimensionality for Control Theory
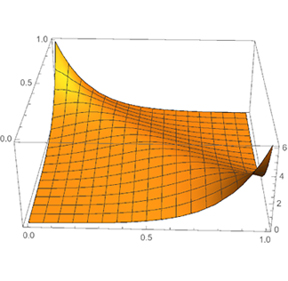
Optimal control problems lead to Hamilton-Jacobi Bellman (HJB) differential equations in many space variables for finding the cost function to be optimized. This beautiful connection has not generally led to effective numerical methods because grid based solutions of partial differential equations in \( n \) variables generally have memory requirements and complexity that is exponential in \( n...

Illumination and Security
ALEX WRIGHT AND KATHRYN MANN Imagine that you are in a room with walls made out of mirrors. The room may be very oddly shaped and have corridors and nooks, but all the walls are at mirrored planes. If you light a single lamp, must every point in the room be illuminated? Perhaps surprisingly, the answer to this problem is “no”, and the reasons are connected with deep mathematical problems whose...

Limits of Permutations
In a well-shuffled deck of cards, about half of the pairs of cards are out of order. Mathematically, we say that in a permutation [math]$\pi$[/math] of [math]$[n]=\{1,2,\dots,n\}$[/math] there are about [math]$\frac12\binom{n}{2}$[/math] inversions, that is, pairs [math]$i<j$[/math] for which [math]$\pi(j)<\pi(i)$[/math]. Suppose we are interested in studying permutations for which the number...

A chromatic look at the homotopy groups of spheres
A hallmark of algebraic topology is its collection of decades-old computational problems that have attracted considerable attention and yet remain unsolved. The most famous is the computation of the homotopy groups of spheres, which asks for a classification of all continuous functions Sk → Sn from the k-dimensional sphere to the n-dimensional sphere up to continuous definition. When k < n, it...

The Topology of Algebraic Varieties
During the academic year 2014-2015, the School of Mathematics conducted a special program on The Topology of Algebraic Varieties. The program was led by Distinguished Visiting Professor Claire Voisin, from CNRS and Institut de Mathématiques de Jussieu, and Member Burt Totaro from UCLA. The central themes of the program were Hodge theory and algebraic cycles. A large group of Members took part...

Modeling the Nation’s Food System
With over seven billion people living on our planet, how do we ensure a secure food system? How do we manage to produce, harvest, process, transport, sell, and eventually prepare the food we consume and do this better so that over one billion people are not hungry? Food system activities, like those described above comprise a highly complex system, with complicated feedback loops. To address...

Topology of Shapes, Persistent Homology and Point Clouds: Where Does it Take Us?
When viewed from the outside, a human brain appears as a volume with a highly wrinkled surface having numerous long crevices. Sulcal fundi are 3D curves that lie in the depths of the cerebral cortex; informally, the fundus of a sulcus is the curve of maximal average depth that spans the length of the sulcus. The sulcal fundi serve as anatomical landmarks, `segmenting’ the cortex into...

Non-equilibrium Dynamics and Random Matrices
During the academic year 2013-2014, the School of Mathematics conducted a special program on Non-equilibrium Dynamics and Random Matrices. This program was led by Distinguished Visiting Professor Horng-Tzer Yau from Harvard University, and Thomas Spencer (IAS). Yau is a leading expert on both random matrix theory and non-equilibrium dynamics. He was joined by senior participants L. Erdös, J....