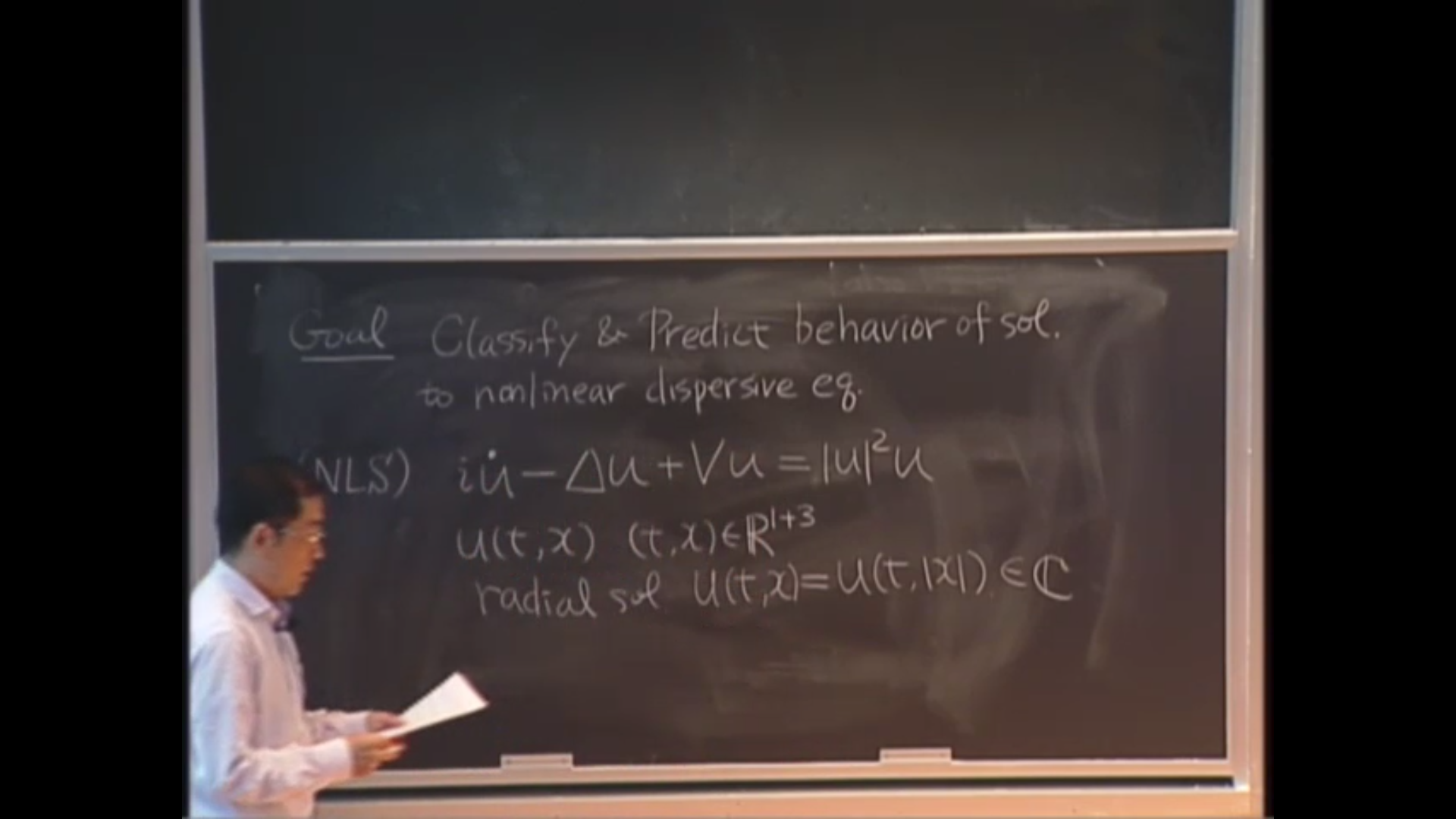Solutions of nonlinear dispersive equations exhibit various space-time behavior, such as blow-up, soliton, and scattering, due to competition between the dispersion and the nonlinearity. Drastic changes are also possible along the evolution. It is hence an important and challenging problem to predict the behavior in all the future and the past from the initial data. Combining variational, dispersive, and spectral analysis, it has become possible to describe the structure of solutions and of initial data in some simple cases. In this talk I will mostly focus on the nonlinear Schrodinger equations with or without potential, which have stable and/or unstable solitons. The main goal is to obtain a global dynamical picture which contains deformation between different types of solitons.