Open-Closed Gromov-Witten theory and Floer homology
Presenter
October 31, 2011
Keywords:
- Shiing-Shen Chern
- differential geometry
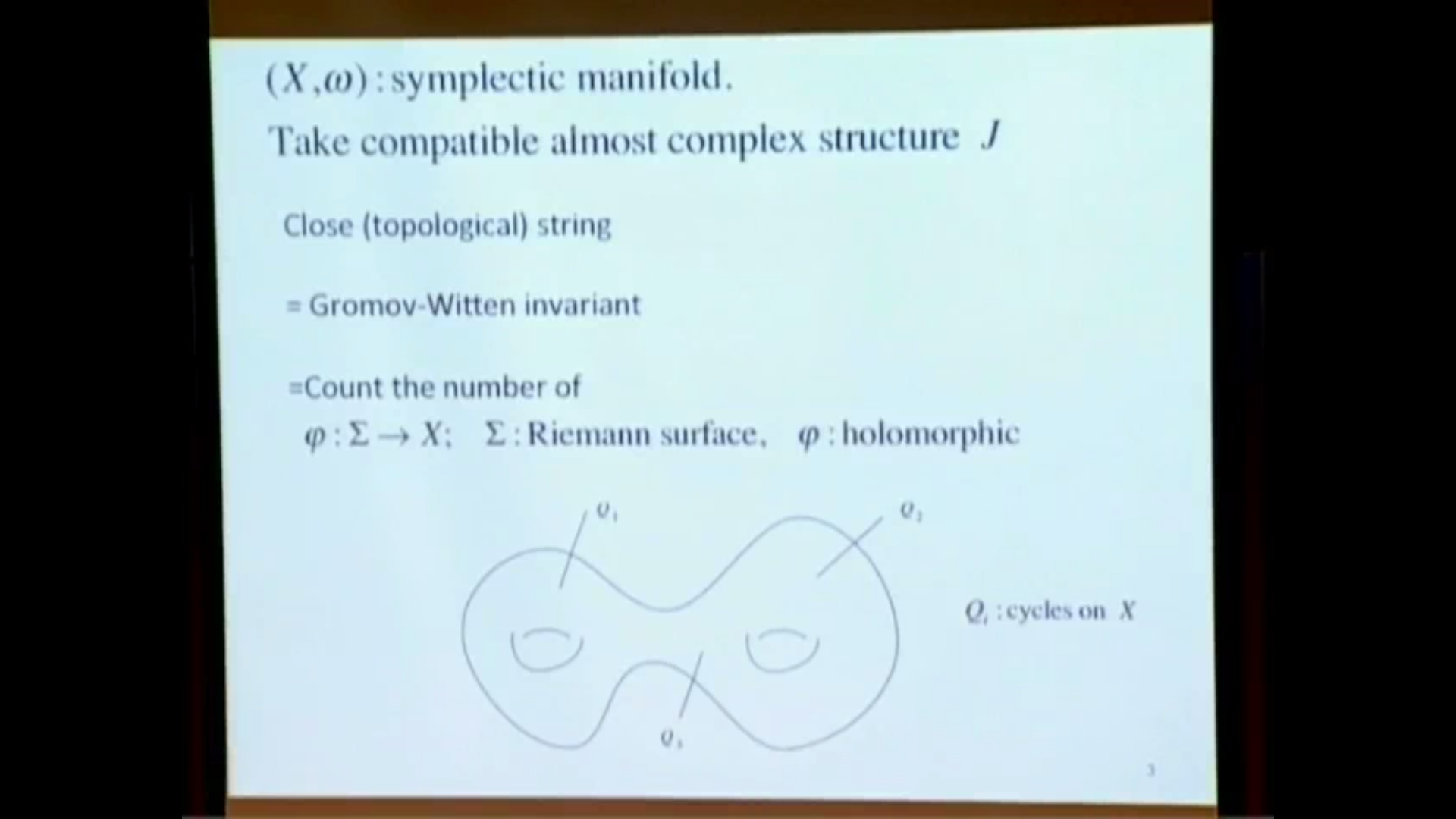
- Gromov-Witten theory
- moduli spaces
- Lagrangian Floer homology
- mirror symmetry
- symplectic geometry
MSC:
- 53D45
- 53D42
- 53D40
- 53D37
- 53Dxx
- 53-xx
- 57R58
Abstract
Open-closed Gromov-Witten theory is a study of moduli space of bordered pseudo-holomorphic curve in a symplectic manifold that bounds Lagrangian submanifold(s). It plays an important role to connect Lagrangian Floer theory with (closed) Gromov-Witten theory. In this talk I want to explain some of the results obtained in Open-closed Gromov-Witten theory and its application to symplectic topology and Mirror symmetry.