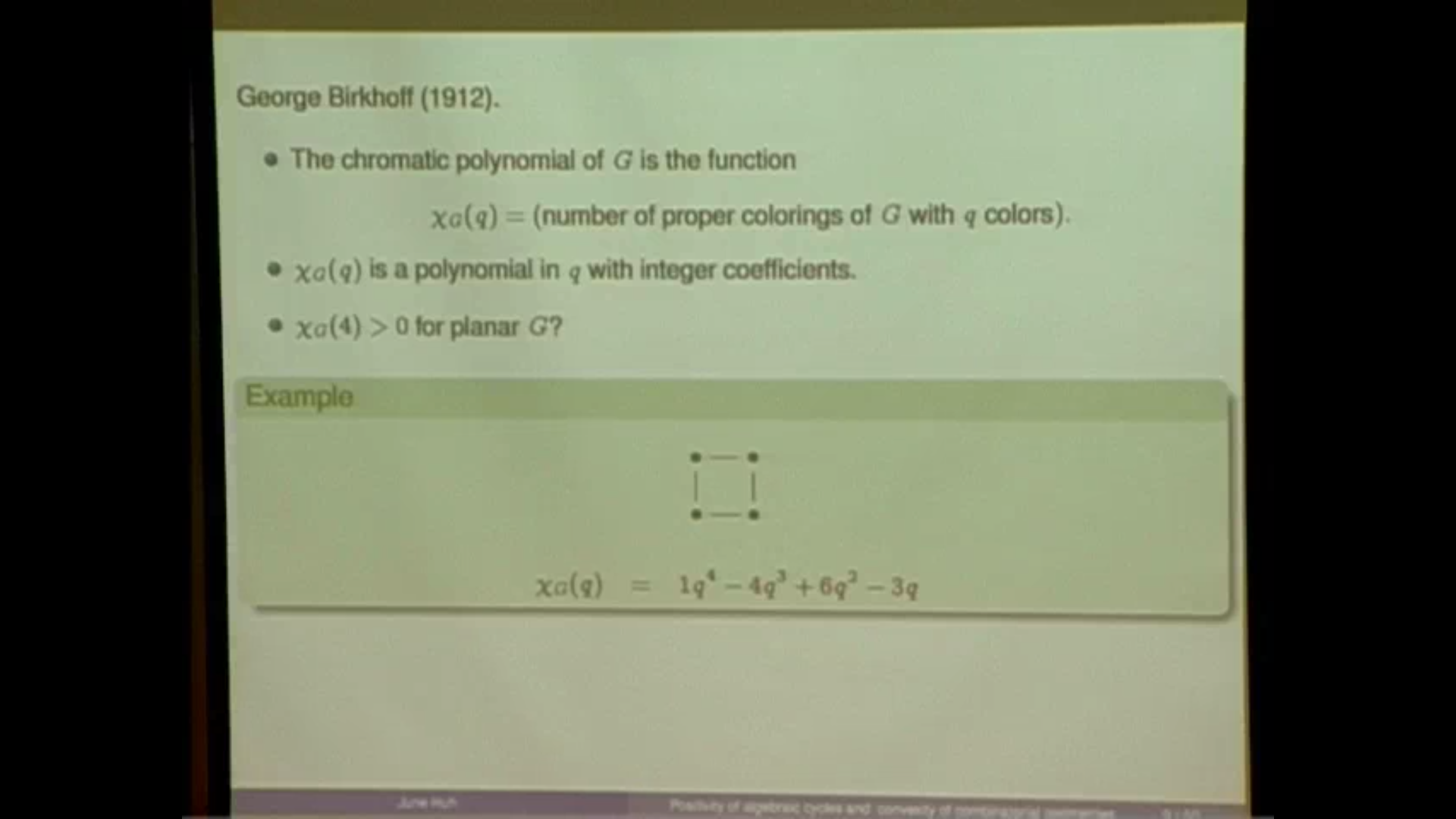
Rota's conjecture predicts that the coefficients of the characteristic polynomial of a matroid form a log-concave sequence. I will outline a proof for representable matroids and explain its relation to a theory of characteristic classes of algebraic varieties. The conjecture in the general case (for possibly nonrepresentable matroids) leads to several intriguing questions on higher codimension algebraic cycles in toric varieties.