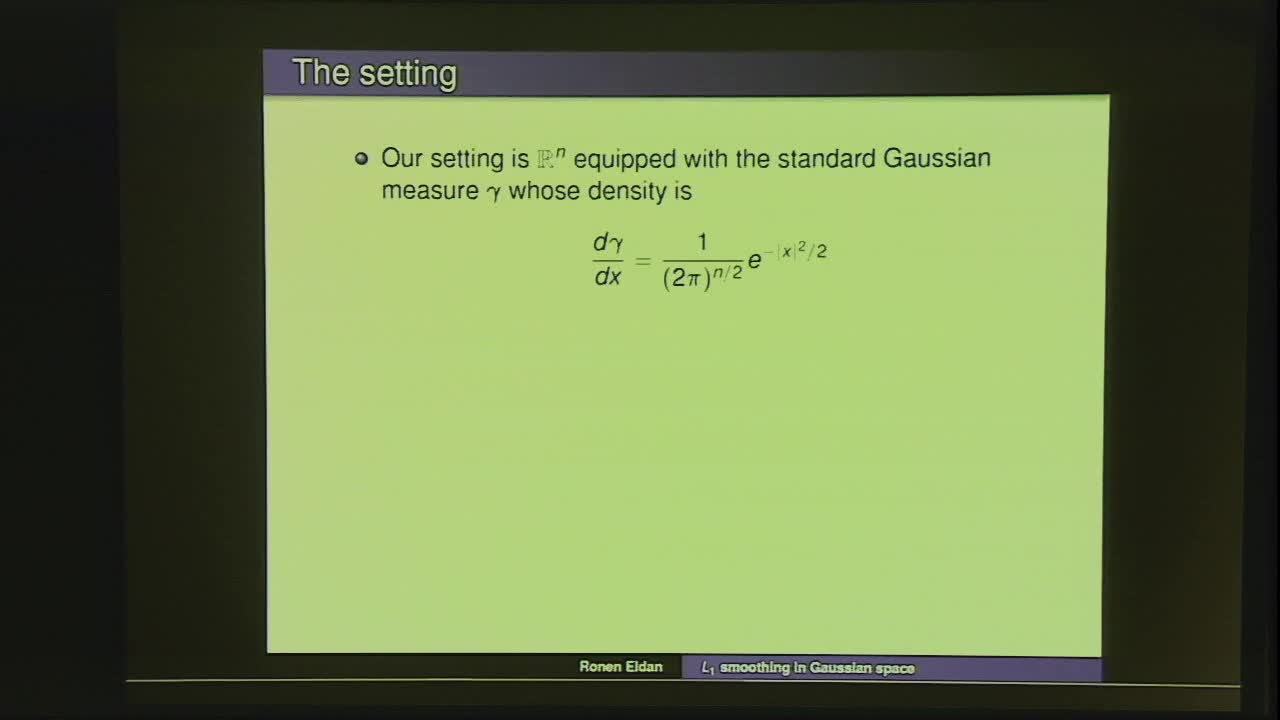
We prove that any non-negative function f on Gaussian space that is not too log-concave (namely, a function satisfying Hess(log(f)) > - C Id) has tails strictly better than those given by Markov's inequality: P(f > c) < E[f]/(c (log c)^{1/2}) where E[f] denotes the (Gaussian) expectation of f. An immediate consequence is a positive answer to the Gaussian variant of Talagrand's (1989) question about regularization of L^1 functions under the convolution semigroup.