Embedding Percolation
Presenter
February 20, 2012
Keywords:
- mathematical statistical mechanics
- percolation
- probability theory
- phase transitions
- scaling laws
- SLE
- Ising model
- queueing theory
MSC:
- 82C20
- 82C26
- 82C27
- 82C05
- 82C44
- 82C43
- 82Cxx
- 82-xx
- 60K35
- 60K25
- 60Kxx
Abstract
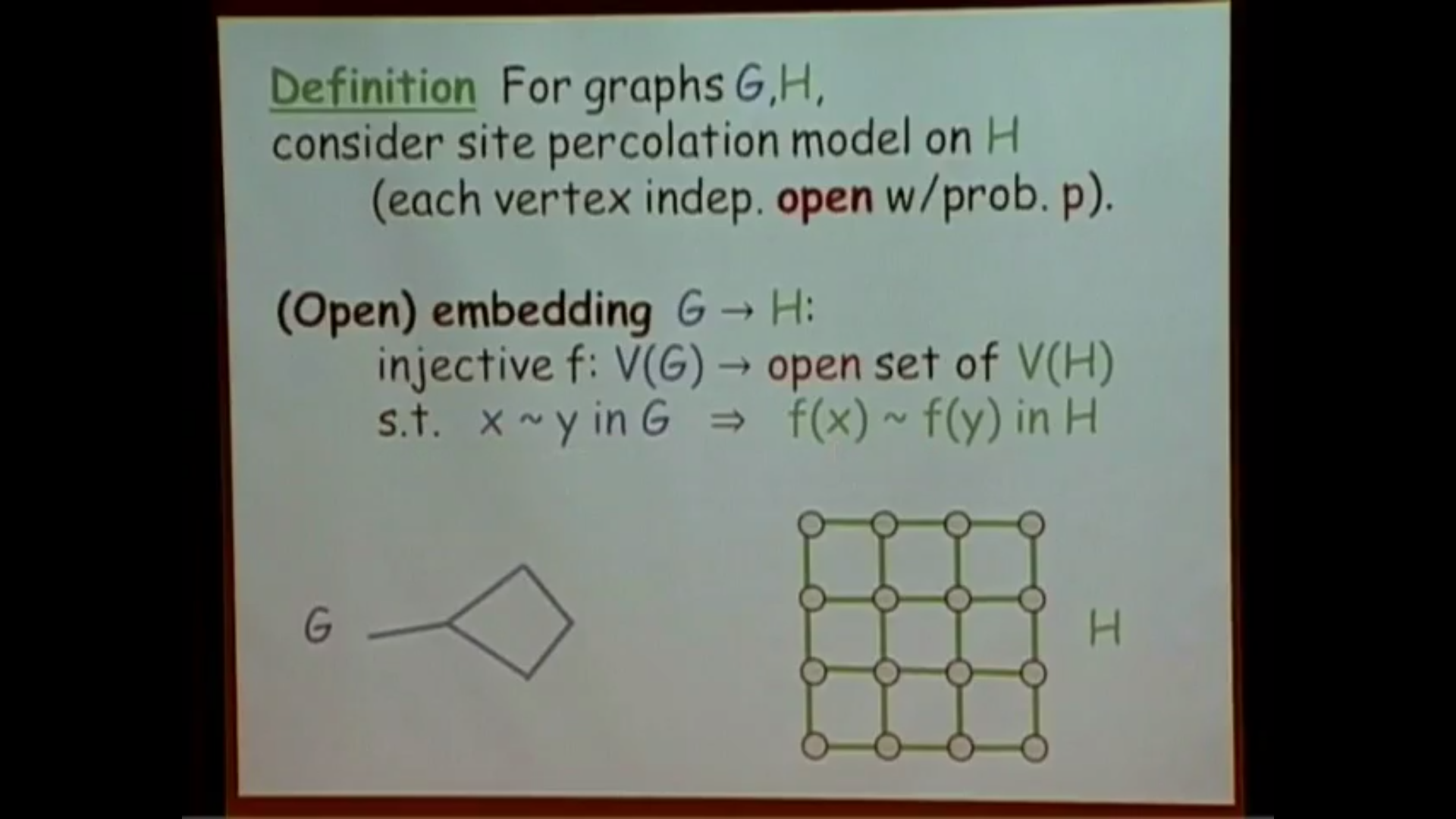
Percolation is concerned with the existence of an infinite path in a random subgraph of a given graph H. We can rephrase this as the existence of an injective graph homomorphism (or an injective 1-Lipschitz map) from the infinite line Z+ to the random subgraph. What happens if we replace Z+ with another graph G? Answering this for various choices of G and H will lead us to a surprising range of topics, including topological combinatorics, first-passage percolation, and queueing theory. Based on joint works with Dirr, Dondl, Grimmett, Martin and Scheutzow.