The nested loop approach to the O(n) model on random maps
Presenter
March 26, 2012
Keywords:
- mathematical physics
- mathematical statistical mechanics
- conformal field theory
- conformal invariance
- probability theory
- random point process
- SLE
- loop gases
- dynamical random lattices
- partition functions
MSC:
- 60K35
- 60J45
- 60J65
- 60J67
- 60Jxx
- 60-xx
- 60G57
- 60G60
- 82-xx
- 82B43
- 82B44
Abstract
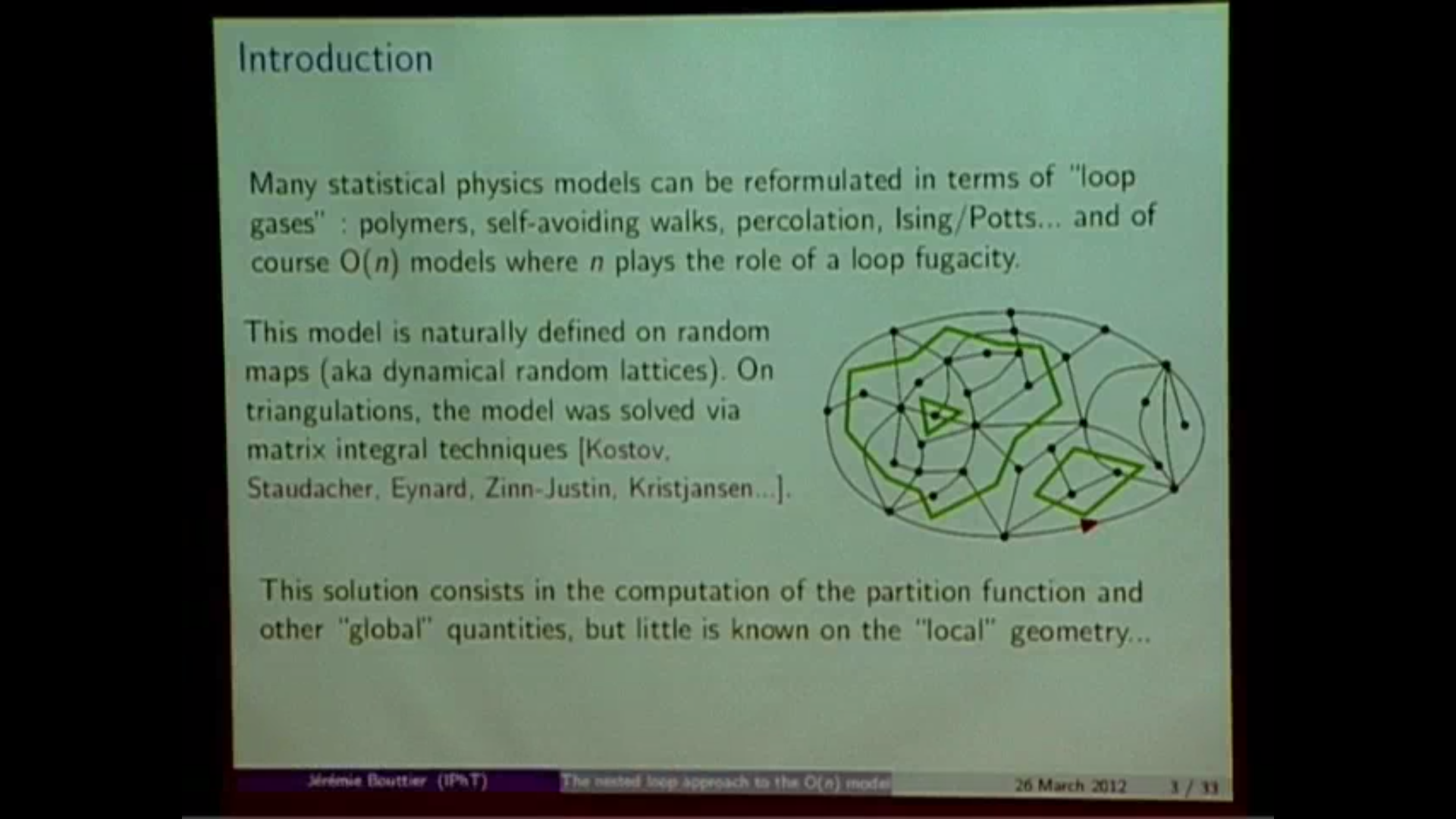
We consider the O(n) loop model on random planar maps (i.e. graphs embedded in the sphere). We explain how an elementary combinatorial decomposition, which consists in cutting the maps along the outermost loops, allows to relate the O(n) model to the simpler problem of counting maps with controlled face degrees. This translates into a functional relation for the ``resolvent'' of the model, which is exactly solvable in several interesting ases. We then look for critical points of the model: our construction shows that at the so-
called non-generic critical points, the O(n) model is related to the ``stable'' maps introduced by Le Gall and Miermont.