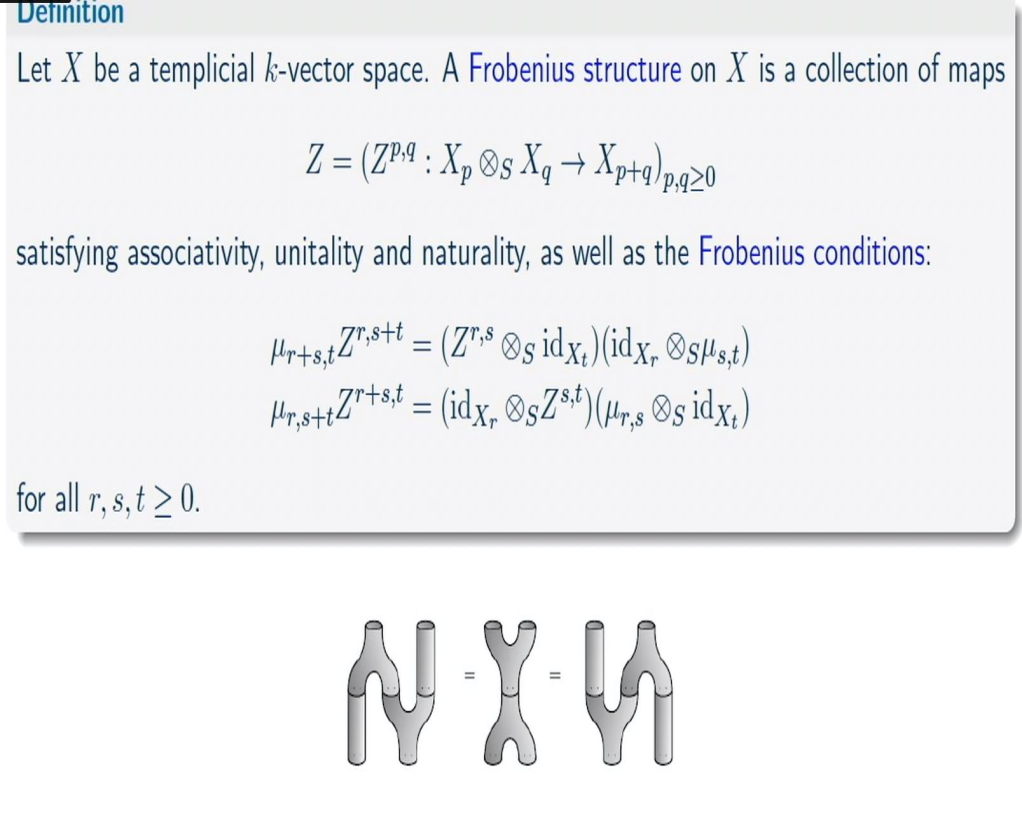
Dg-categories have taken centre stage as models for spaces in noncommutative algebraic geometry through the work of Van den Bergh and others. From a homotopical viewpoint however, dg-categories are "too strict". For example, they possess a model structure and a monoidal structure, but these are not compatible. In higher category theory, a similar problem arises with simplicial categories, and this is resolved by turning to a Quillen-equivalent model of infinity-categories such as Joyal's quasi-categories. In this talk I will introduce a candidate model for non-negatively graded dg-categories which is analogous to that of quasi-categories. We call them "quasi-categories in vectorspaces". Equipping these with some extra structure, they are (on the nose) equivalent to non-negatively graded dg-categories, and the comparison goes through a lift of the dg-nerve. At present, no analogue of Joyal's model structure exists for quasi-categories in vectorspaces, but I will outline some partial results in that direction.