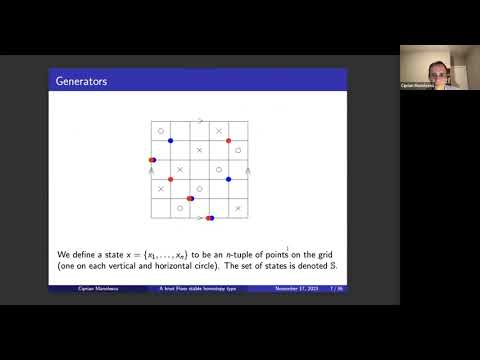
Given a grid diagram for a knot or link in the three-sphere, we construct a spectrum whose homology is the knot Floer homology of . We conjecture that the homotopy type of the spectrum is an invariant of . Our construction does not use holomorphic geometry, but rather builds on the combinatorial definition of grid homology. We inductively define models for the moduli spaces of pseudo-holomorphic strips and disk bubbles, and patch them together into a framed flow category. The inductive step relies on the vanishing of an obstruction class that takes values in a complex of positive domains with partitions. (This is joint work with Sucharit Sarkar.)