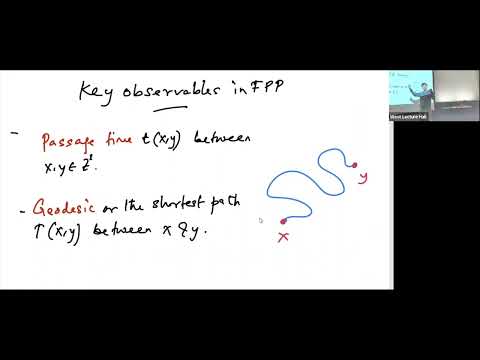
Planar last passage percolation models are canonical examples of stochastic growth, polymers and random geometry in the Kardar-Parisi-Zhang universality class, where one considers oriented paths between points in a random environment accruing the integral of the noise along itself as its weight. Given the endpoints, the extremal path with the maximum weight is termed as the geodesic.  These models can also be viewed as examples of  disordered systems admitting complex energy landscapes. In this formulation, the geodesic is the ground state, the configuration with lowest energy, lying at the base of the deepest valley.  In this talk we will review various recent results about such models related to fractal geometry, noise-sensitivity and chaos.