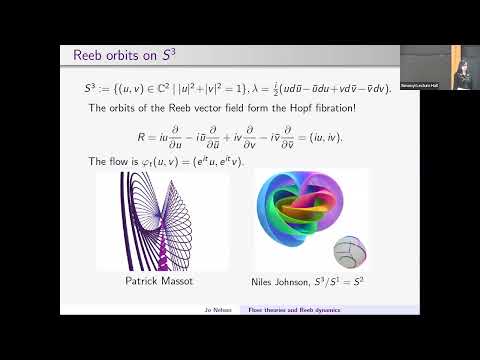
Contact topology is the study of certain geometric structures on odd dimensional smooth manifolds. A contact structure is a hyperplane field specified by a one form which satisfies a nondegeneracy condition called maximal non-integrability. The associated one form is called a contact form and uniquely determines a Hamiltonian-like vector field called the Reeb vector field on the manifold. I will give some background on this subject, including motivation from classical mechanics. I will then explain how to construct and compute Floer theoretic contact invariants. These are a sort of infinite dimensional version of Morse theory wherein the chain complexes are generated by closed Reeb orbits and the differential counts certain J-holomorphic curves. This talk will feature numerous graphics and anecdotes.