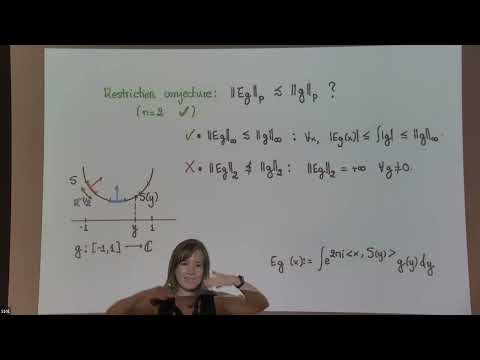The restriction conjecture, one of the most central problems in harmonic analysis, studies the Fourier transform of functions defined on curved surfaces; specifically, it claims that the level sets of such Fourier transforms are relatively small. The Mizohata-Takeuchi conjecture further studies the shape of these level sets, and in particular the extent to which they can avoid clustering on lines. In this talk we will present a small improvement on the Mizohata-Takeuchi conjecture. This is joint work with Anthony Carbery and Hong Wang.