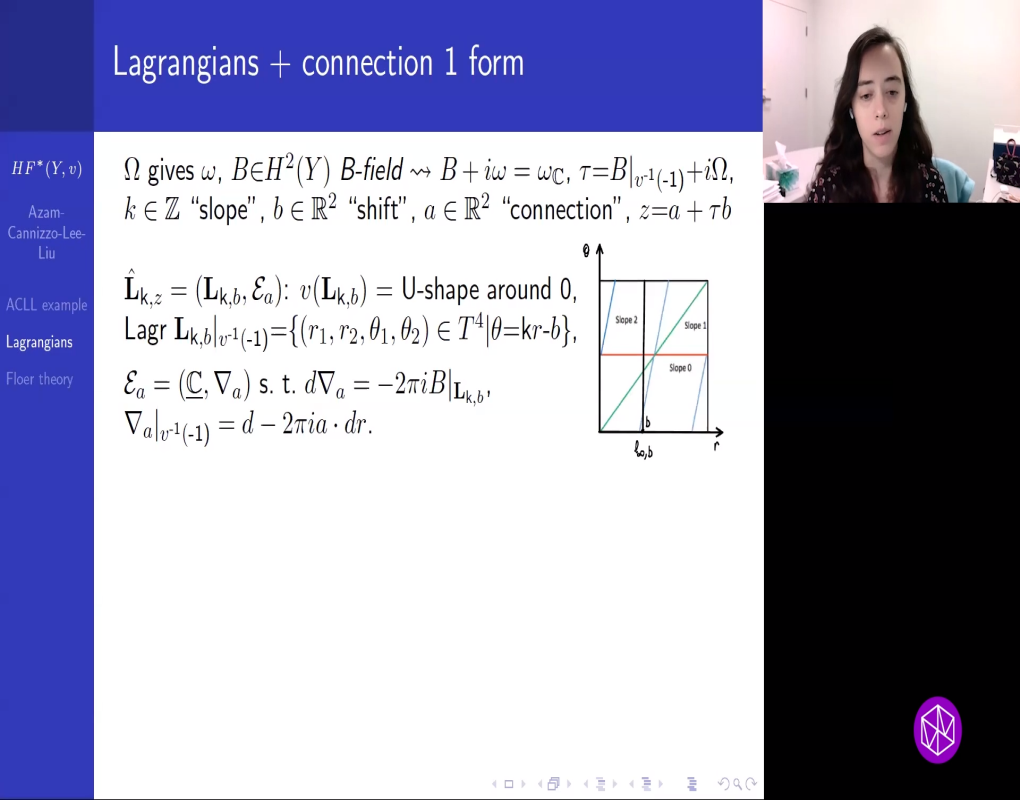
Lagrangian intersection Floer theory defines morphisms in the Fukaya category of a closed symplectic manifold, or the Fukaya-Seidel category of a symplectic fibration over a two dimensional base with boundary. We give an example of this cohomology theory in a certain symplectic fibration with T^4 fibers, critical locus given by the banana manifold, and "U-shaped" Lagrangians.