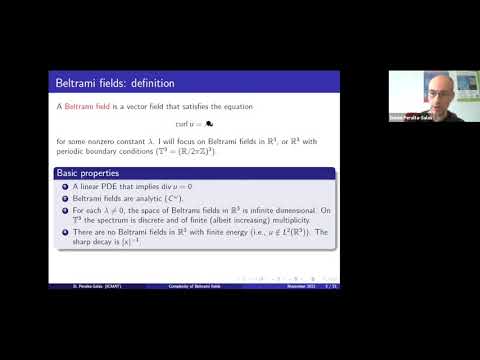
Beltrami fields, that is vector fields on $\mathbb R^3$ whose curl is proportional to the field, play an important role in fluid mechanics and magnetohydrodynamics (where they are known as force-free fields). In this lecture I will review recent results on the complexity exhibited by these fields from different viewpoints: probabilistic, computational and dynamical. In particular, I will show the existence of Beltrami fields that can simulate a universal Turing machine (joint with Cardona and Miranda), and that a random Beltrami field has positive topological entropy almost surely (joint with Enciso and Romaniega).