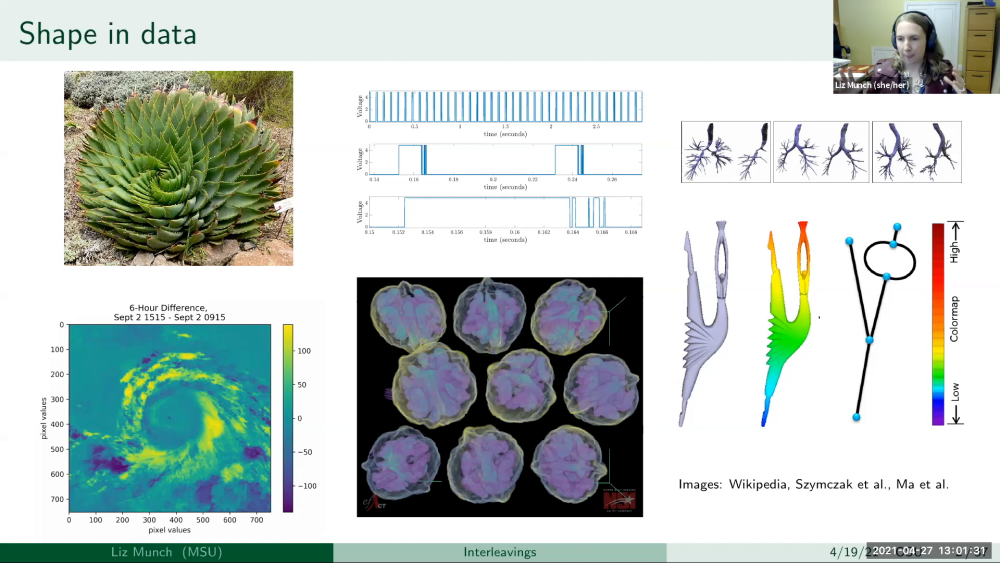
Reeb graphs and main other related graphical signatures have extensive use in applications, but only recently has there been intense interest in finding metrics for these objects. In this talk, we focus on the interleaving distance, which is a categorical reforumlation of the epynomous metric from persistence modules. In this talk, we introduce an extension of smoothing on Reeb graphs, which we call truncated smoothing; this in turn allows us to define a new family of metrics which generalize the interleaving distance for Reeb graphs. Intuitively, we “chop off” parts near local minima and maxima during the course of smoothing. After formalizing truncation as a functor, we show that when applied after the smoothing functor, this prevents extensive expansion of the range of the function, and yields particularly nice properties. Further, for certain choices of the truncation parameter, we can construct a categorical flow for any choice of slope m ∈ [0, 1], which gives a family of interleaving distances. While the resulting metrics are not stable, we show that any pair of these for m, m' ∈ [0,1) are strongly equivalent metrics, which in turn gives stability of each metric up to a multiplicative constant