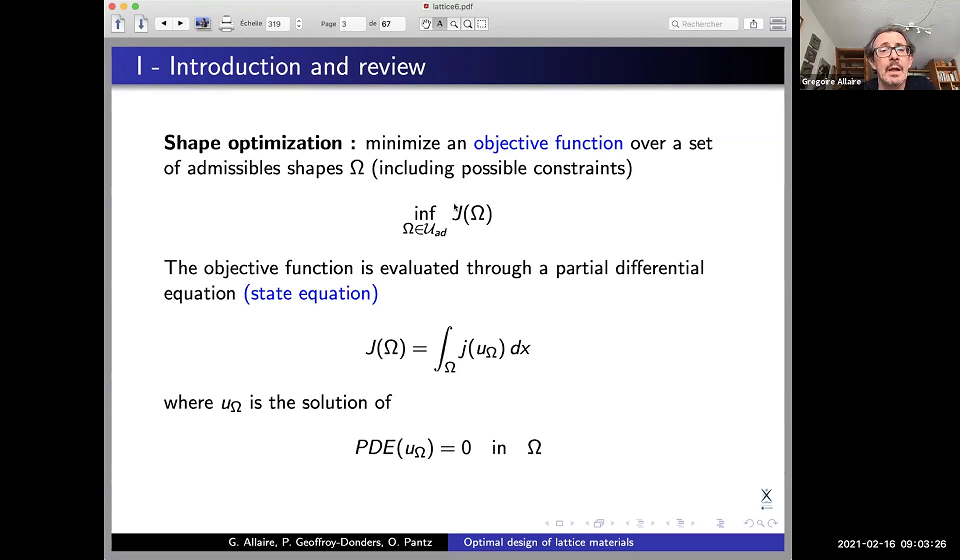
This work is concerned with the topology optimization of so-called lattice materials, i.e., porous structures made of periodically perforated material, where the microscopic periodic cell can be macroscopically modulated and oriented. Lattice materials are becoming increasingly popular since they can be built by additive manufacturing techniques. The main idea is to optimize the homogenized formulation of this problem, which is an easy task of parametric optimization, then to project the optimal microstructure at a desired length-scale, which is a delicate issue, albeit computationally cheap. The main novelty of our work is, in a plane setting, the conformal treatment of the optimal orientation of the microstructure. In other words, although the periodicity cell has varying parameters and orientation throughout the computational domain, the angles between its members or bars are conserved. Several numerical examples are presented for compliance minimization in 2-d. Extension to the 3-d case will also be discussed.