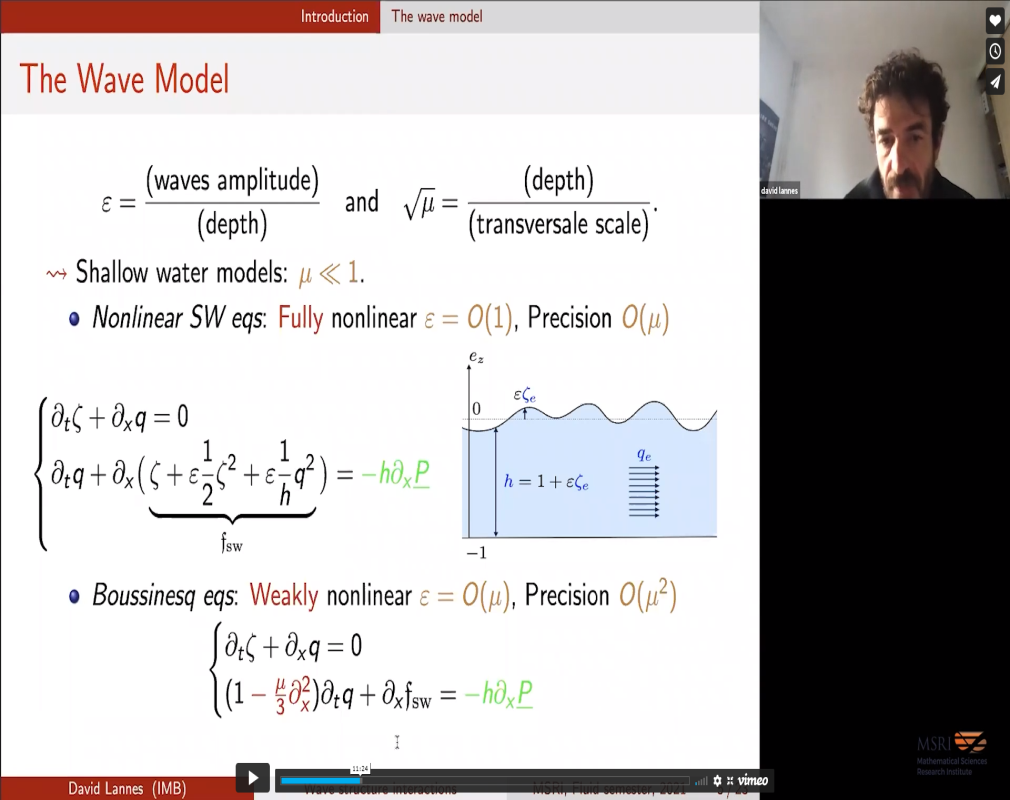
There are different formulations of the water waves problem. One of them is to formulate it as a system of equations coupling two quantities, e.g. the free surface elevation $\zeta$ and the horizontal discharge $Q$. Actually, one can understand the water waves problem as a system on three quantities, $\zeta$, $Q$ and the surface pressure $P_s$ under the constraint that $P_s$ is constant (and therefore disappears from the equations). When we consider in addition a floating body then, under the body, we still have a system of equations on the same three quantities, but this time the constraint is not on the pressure but on the surface of the water, that much coincide with the bottom of the floating object. Wave-structure interactions can be understood as the coupling of these two different constrained problems. We shall briefly analyse this coupling and show among other things how it dictates the evolution of the contact line between the surface of the water and the surface of the floating body, and how to transform it into transmission problems that raise many mathematical issues such as fully nonlineary hyperbolic initial boundary value problems, dispersive boundary layers, initial boundary value problems for nonlocal equations, etc.