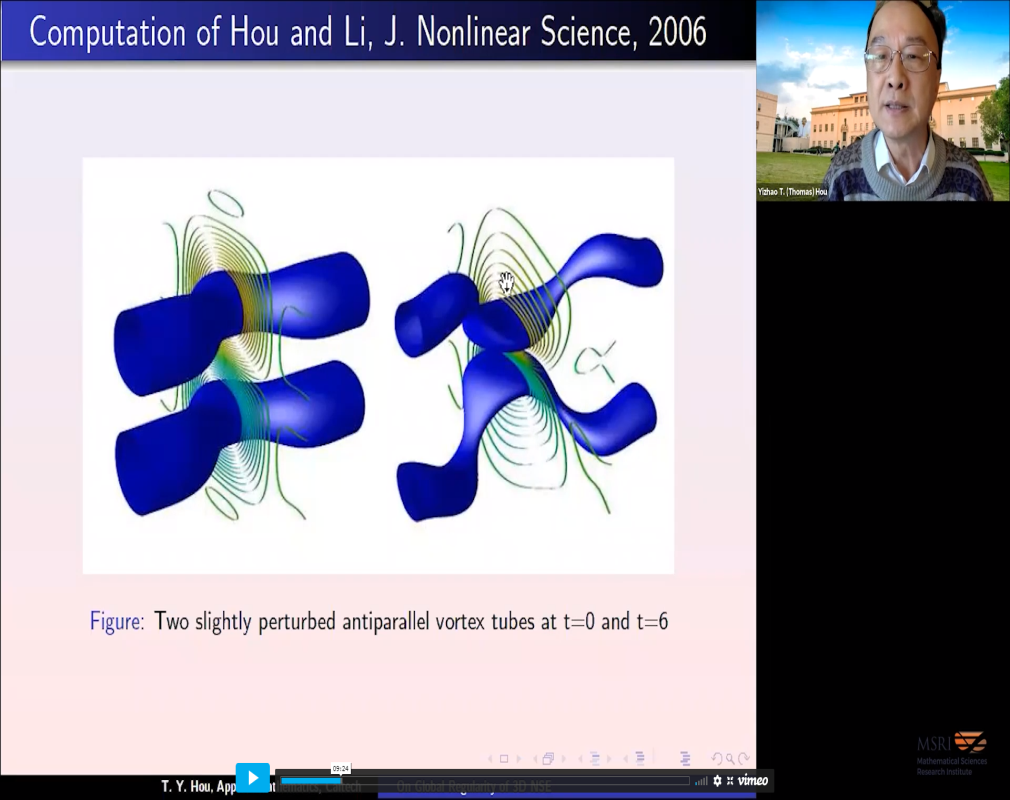
Whether the 3D incompressible Euler equations can develop a singularity in finite time from smooth initial data is one of the most challenging problems in mathematical fluid dynamics. We first review the numerical evidence of finite time singularity for 3D axisymmetric Euler equations by Luo and Hou. The singularity is a ring like singularity that occurs at a stagnation point in the symmetry plane located at the boundary of the cylinder. We then present a novel method of analysis and prove that the 1D HL model and the original De Gregorio model develop finite time self-similar singularity. We will also report our recent progress in analyzing the finite time singularity of the axisymmetric 3D Euler equations with initial data considered by Luo and Hou. Finally, we present some recent numerical results on singularity formation of the 3D axisymmetric Euler equation along the symmetry axis.