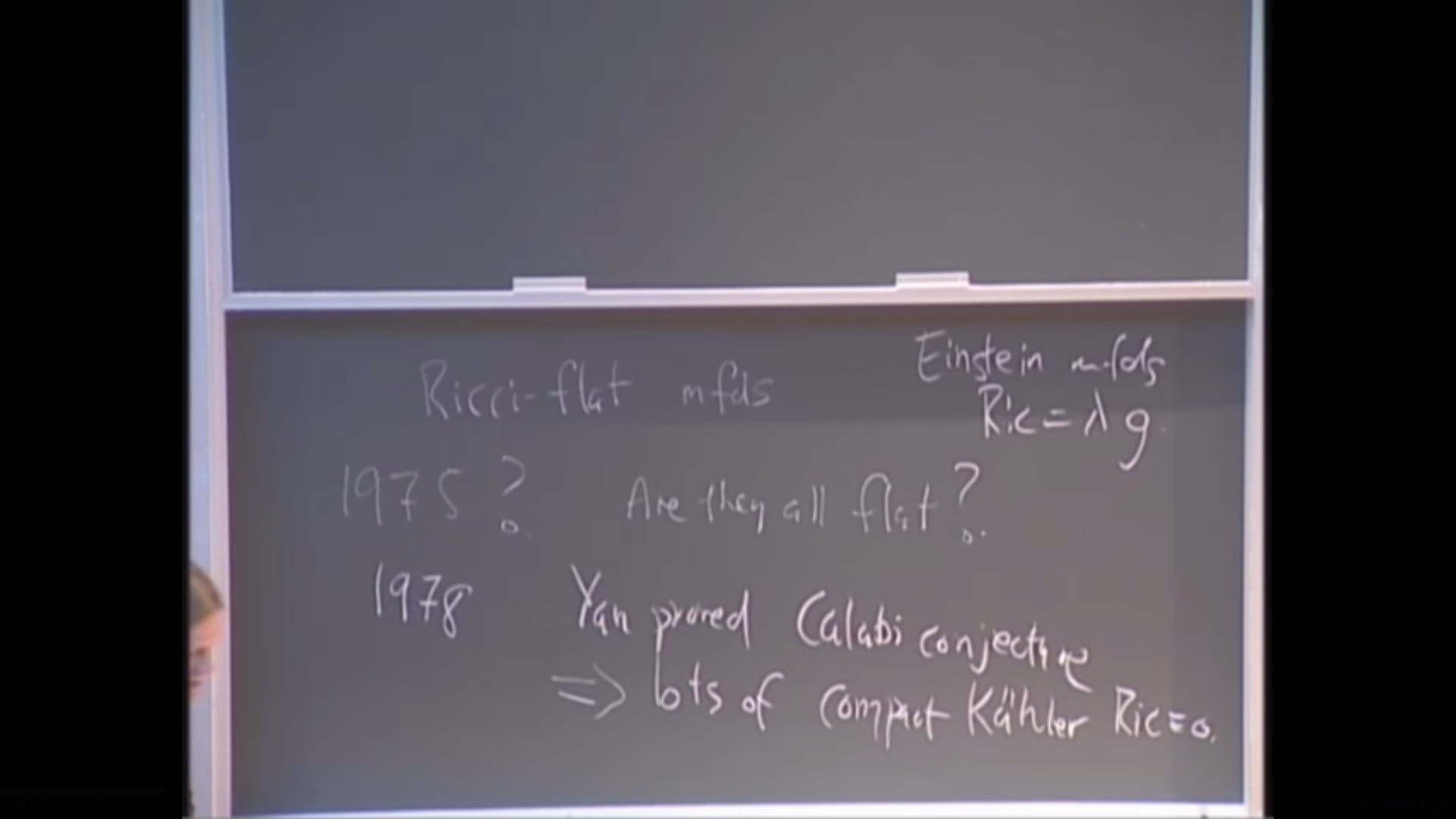Ricci flat spaces and metrics with special or exceptional holonomy I
Presenter
January 18, 2016
Keywords:
- differential geometry
- Riemannian geometry
- modern geometry
- curvature
- curvature estimates
- Ricci curvature
- Ricci curvature lower bounds
- holonomy
- convergence of metric spaces
- Ricci flatness
MSC:
- 53-XX
- 53CXX
- 53C21
- 53C44
- 54E50
- 53C29
Abstract
To this day the only compact irreducible complete Ricci-flat Riemannian metrics arise as manifolds with special or exceptional holonomy. This pair of talks will give an introduction to special and exceptional holonomy metrics and the resulting constructions of both compact and noncompact Ricci-flat manifolds. We will indicate how ideas from Riemannian convergence theory have provided motivation for the currently known (and possibly for future) constructions of metrics with exceptional holonomy.