Approximating general data by optimally flat data
Presenter
November 20, 2013
Keywords:
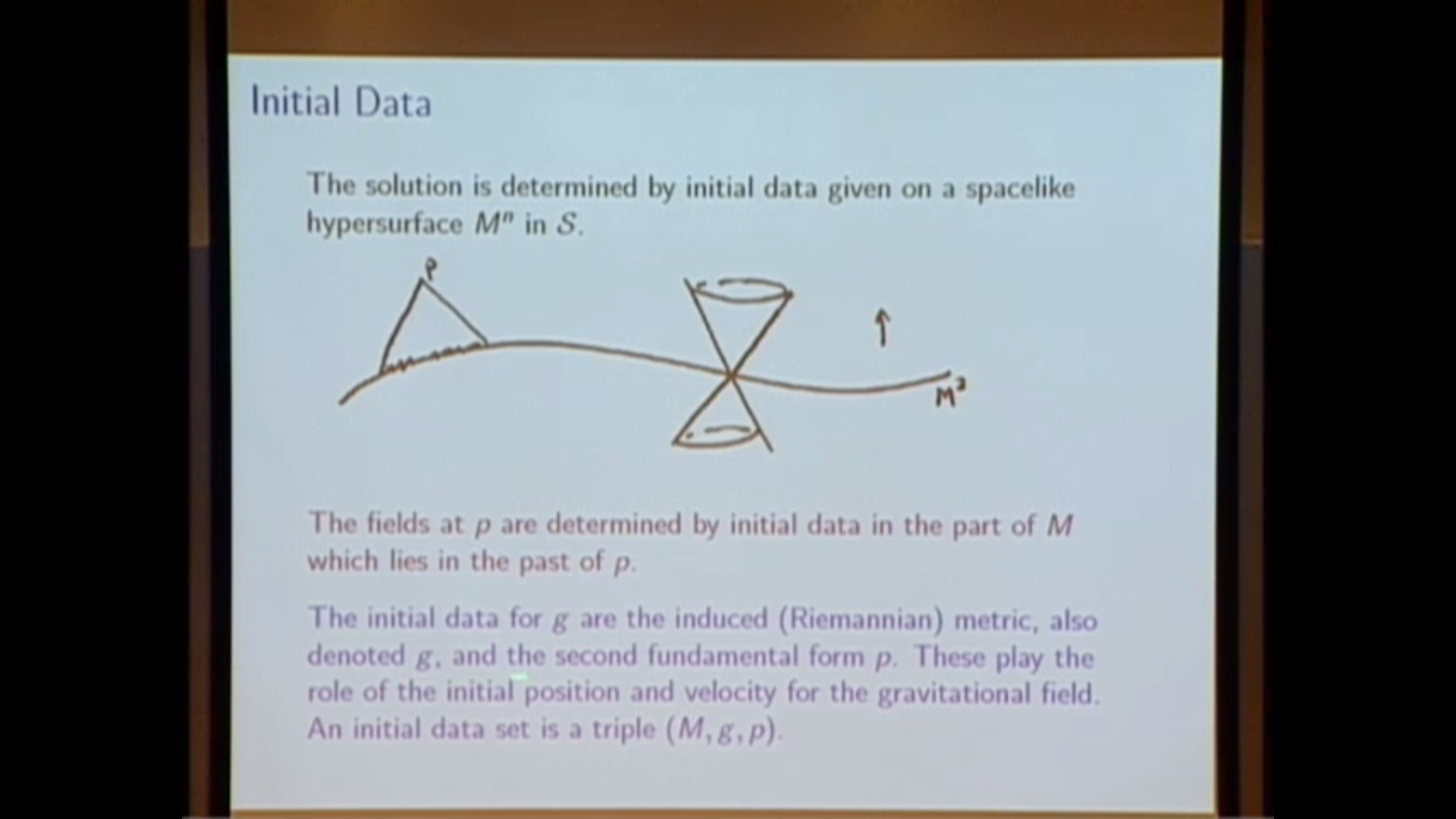
- initial data
- Einstein equations
- Ricci curvature
- differential geometry
- mathematical general relativity
- Lorentzian geometry
- spacelike hypersurfaces
- mean curvature
- minimum energy estimates
MSC:
- 83Cxx
- 83C05
- 83C10
- 83C75
- 83C40
- 83C35
- 83-xx
Abstract
This talk will describe recent work with A. Carlotto on a method for approximating general asymptotically flat initial data sets by ones which are trivial outside cones of arbitrarily small angle. We will explain why this type of approximation is optimal in a certain sense, and we will give some applications of the construction.