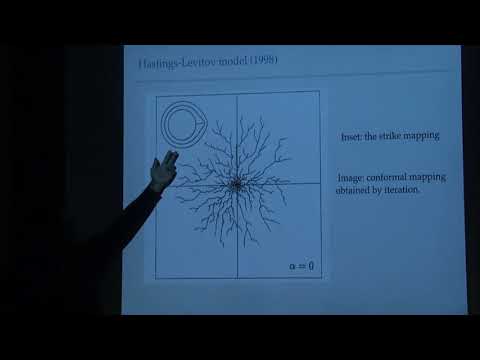
In the early 1920s, Loewner introduced a constructive approach to the Riemann mapping theorem that realized a conformal mapping as the solution to a differential equation. Roughly, the “input” to Loewner’s differential equation is a driving measure and the “output” is a family of nested, conformally equivalent domains. This theory was revitalized in the late 1990s by Schramm. The Schramm-Loewner evolution (SLE) is a stochastic family of slit mappings driven by Loewner’s equation when the driving measure is an atom executing Brownian motion. It is known to describe the scaling limits of several fundamental lattice models.While most of SLE theory is devoted to slit mappings, several particle models such as Diffusion Limited Aggregation (DLA) lead to conformal mappings of domains with “tree-like” boundaries. These models are motivated by physical phenomena such as dielectric breakdown (i.e. lightning). Despite an extensive physics literature, very little is known about the scaling limits of such models. In particular, we do not know if such scaling limits can be described by Schramm-Loewner theory. The purpose of our work is to address this question. We introduce a new stochastic model that extends Schramm-Loewner theory by including branching. The key step is to recognize that the conformal mappings inherit the branching structure of the driving measure provided we choose the driving measure to be a branching Dyson Brownian motion. This allows us to describe the scaling limit of the model when particles undergo branching in continuous time. In this limit, the driving measure is a superprocess described by a new stochastic partial differential equation that has several appealing features. There are few technicalities in the talk, since a large part of it involves explaining how to fit together two theories (conformal mapping, branching processes) that are usually not studied together. This is joint with Vivian Olsiewski Healey (University of Chicago).