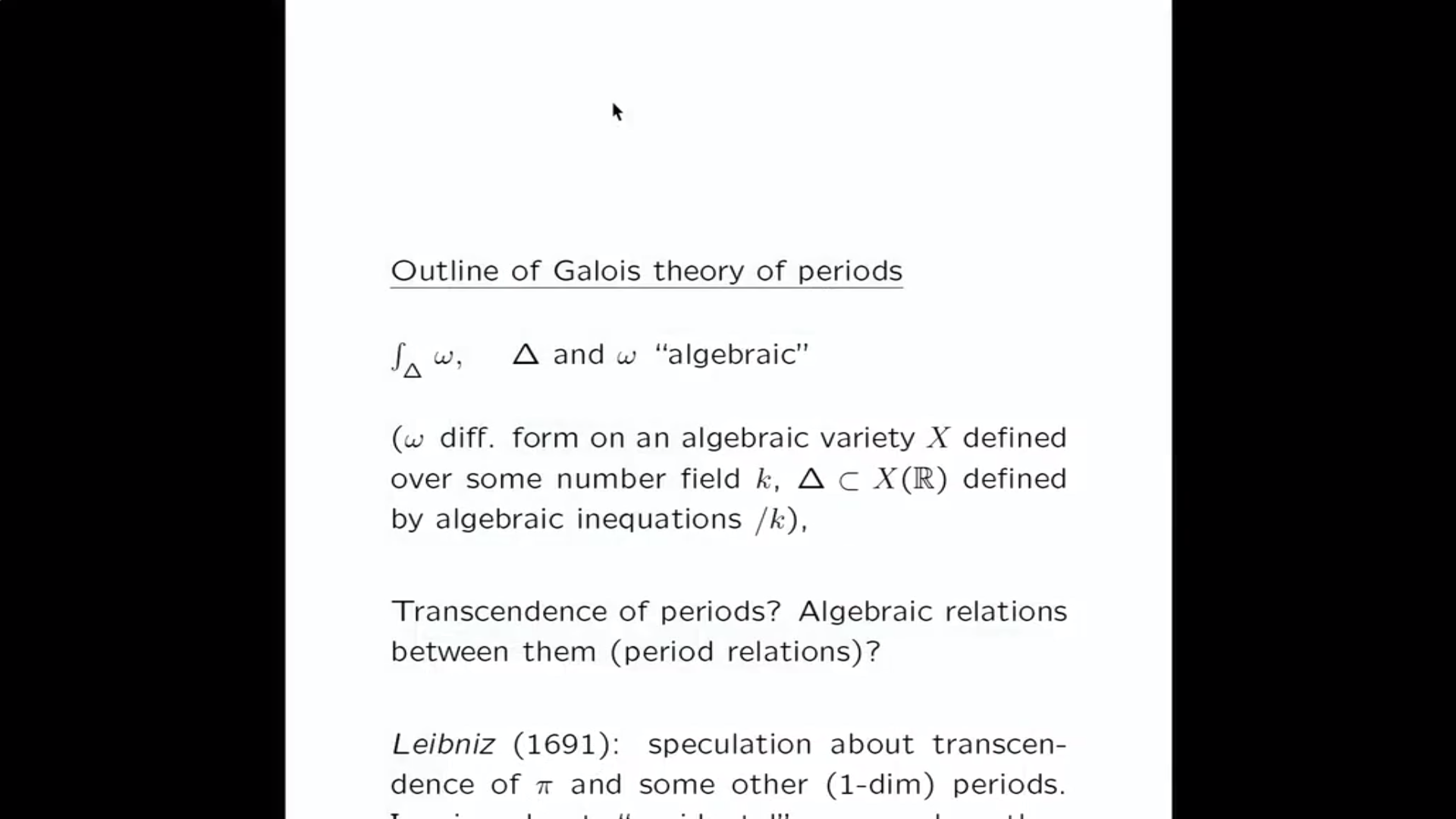
Galois theory of period and the André-Oort conjecture
Presenter
March 27, 2017
Keywords:
- Galois theory
- periods
- Galois orbits
- integration
- algebraic varieties
- algebraic geometry
- motivic geometry
- Kontsevich conjecture
- transcendental numbers
- number fields
- de Rham cohomology
- abelian varieties
MSC:
- 11R34
- 11R45
- 11R32
- 11R70
- 11Rxx
- 11-xx
- 14C15
- 14C35
- 14C30
- 14Cxx
- 14-xx
- 14E18
- 14F42
- 14F40
Abstract
The idea of a Galois theory of periods comes from an insight of Grothendieck. I shall briefly outline of this (conjectural) theory, then sketch the path which led me from it to the AO conjecture, as well as some paths backward. Principally polarized abelian varieties of dimension g are parametrized by the algebraic variety A_g, those with prescribed extra "symmetries" by special subvarieties of A_g, and those with maximal symmetry (complex multiplication) by special points. The AO conjecture characterizes special subvarieties of A_g by the density of their special points. It has been proven last year, after two decades of collaborative efforts putting together many different areas.