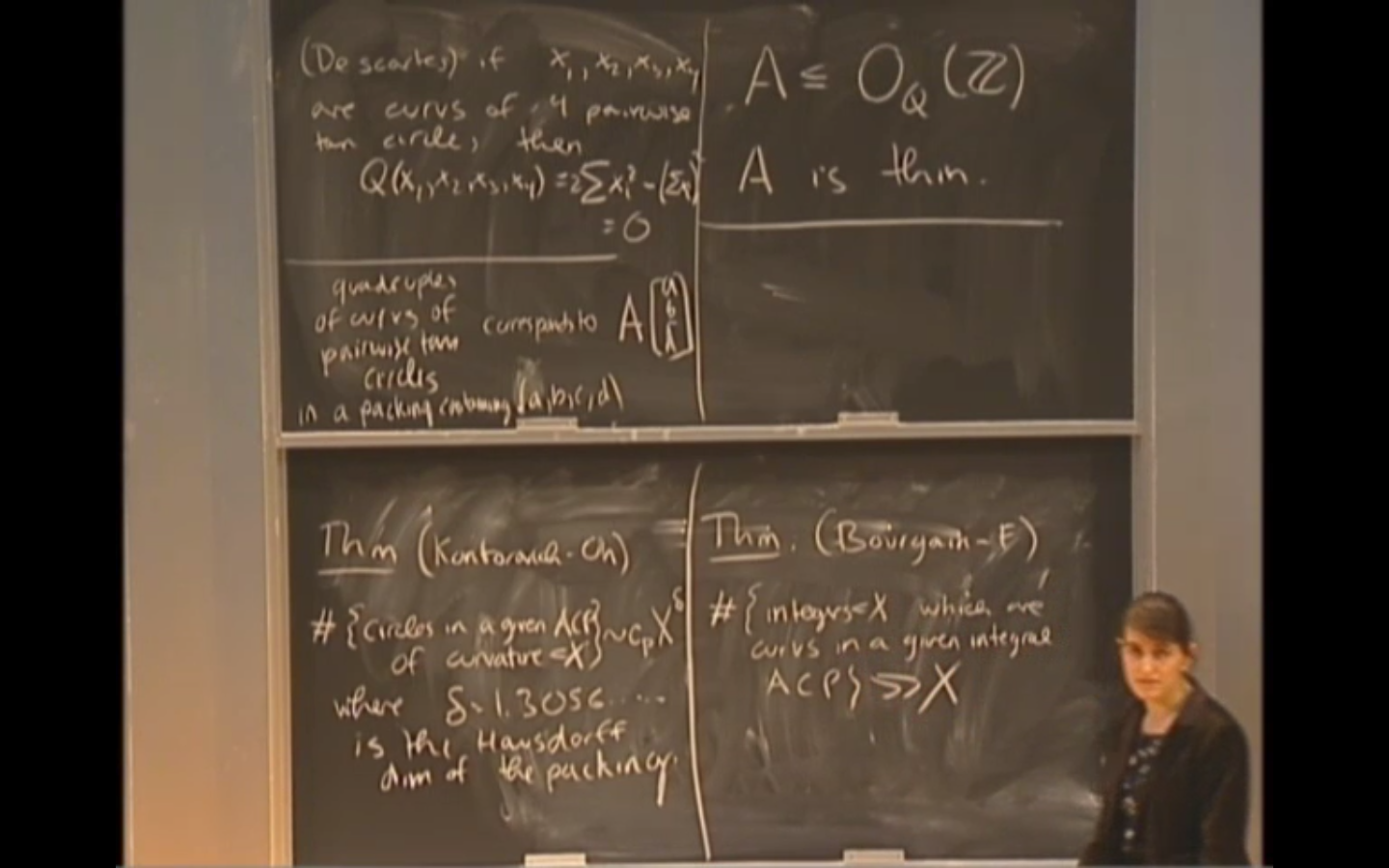Thin groups: arithmetic and geometric viewpoints
Presenter
February 6, 2015
Keywords:
- algebraic groups
- GL(n,Z)
- GL(n,C)
- Apollonian circles
- Hausdorff dimension
- geometric group theory
- arithmetic geometry
- monodromy groups
MSC:
- 20Gxx
- 20-xx
- 37-xx
- 20G20
- 20G99
- 34M35
- 34Mxx
- 20F65
Abstract
In the past decade, substantial progress has been made in several beautiful arithmetic problems connected to thin groups: Apollonian packings, Zaremba's conjecture, and so on. This progress was made possible by a series of developments, in particular the Affine Sieve of Bourgain-Gamburd-Sarnak from a decade ago. In this talk we will focus on the arithmetic of Apollonian packings, and then move on to consider thin groups in their own right, apart from arithmetic applications. Specifically, we will discuss how hyperbolic geometric methods have shed light on thinness of certain monodromy groups of hypergeometric equations, and how one might go about distinguishing thin groups from their non-thin counterparts in general.