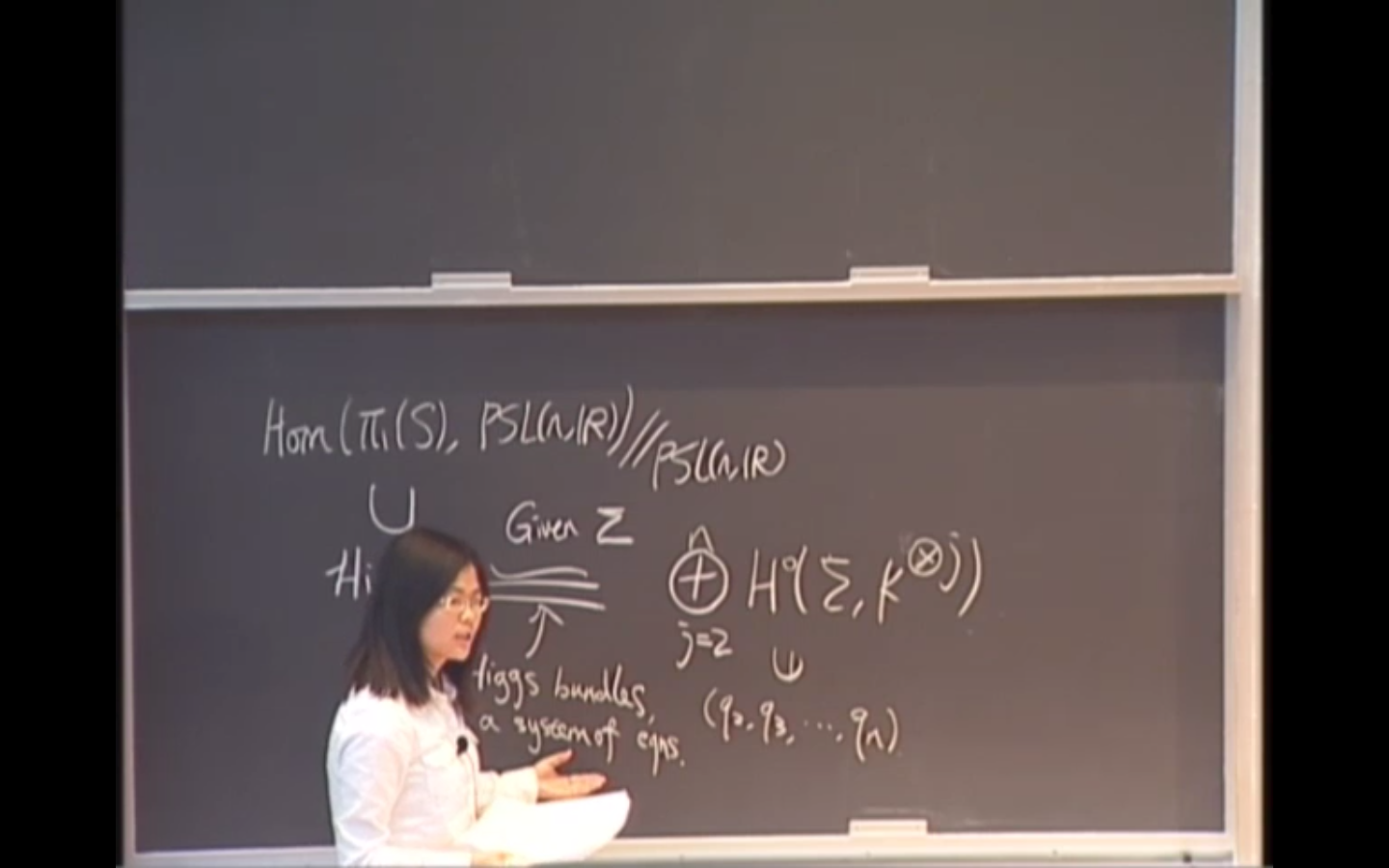Asymptotic Behavior of Certain Families of Higgs bundles in Hitchin Components
Presenter
April 16, 2015
Keywords:
- Hitchin representation
- Hitchin component
- SL(n,R)
- Higgs bundle
- asymptotic geometry
- compactifications of moduli spaces
- Hitchin fibration
MSC:
- 53D18
- 14D20
- 14D06
- 14Dxx
- 14-xx
- 14D21
- 30E15
Abstract
Hitchin component for $SL(n,R)$ is the component in the space of surface group representations into $SL(n,R)$ which can deform to Fuchsian locus. The Hitchin component is in correspondence with the moduli space of $SL(n,R)$-Higgs bundles. I will introduce recent work with Brian Collier on asymptotic behaviors of families in Hitchin component in terms of certain families of Higgs bundles. Namely, given a family of Higgs bundles by scaling Higgs field by $t$, we analyze the asymptotic behavior of the corresponding representations as $t$ goes to $\infty$ in two special cases.