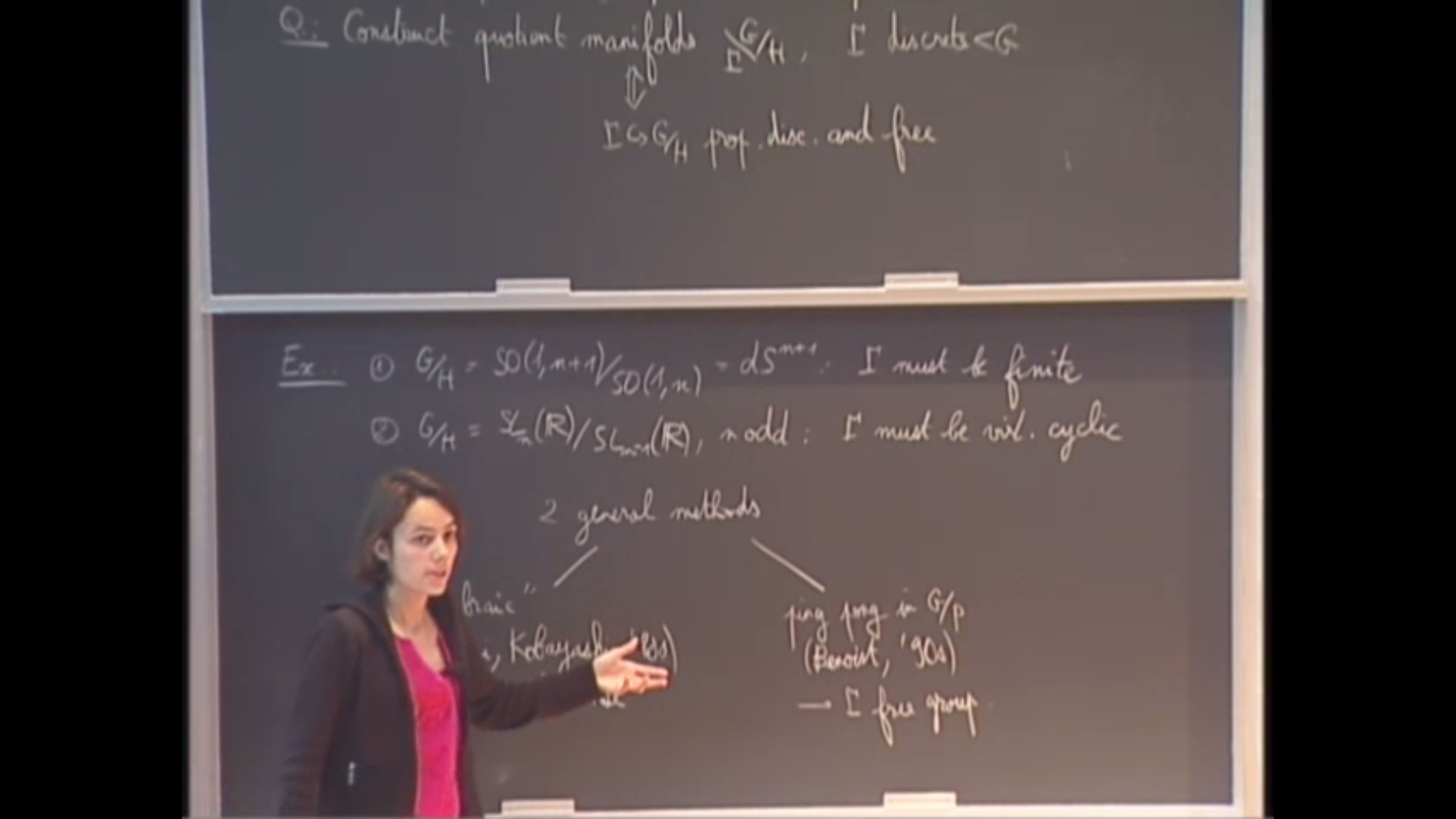Anosov representations and proper actions
Presenter
April 14, 2015
Keywords:
- quotient manifolds
- discrete subgroups
- orthogonal and unitary groups of non-definite signature
- Lorentz group
- Cartan decomposition
- proper actions
- parabolic subgroup
MSC:
- 37D20
- 37Dxx
- 37-xx
- 20F67
- 20Fxx
- 20F65
Abstract
Anosov representations of word hyperbolic groups into reductive Lie groups provide a generalization of convex cocompact representations to higher real rank. I will explain how these representations can be used to construct properly discontinuous actions on homogeneous spaces. For a rank-one simple group G, this construction covers all proper actions on G, by left and right multiplication, of quasi-isometrically embedded discrete subgroups of G×G; in particular, such actions remain proper after small deformations, and we can describe them explicitly. This is joint work with F. Guéritaud, O. Guichard, and A. Wienhard.