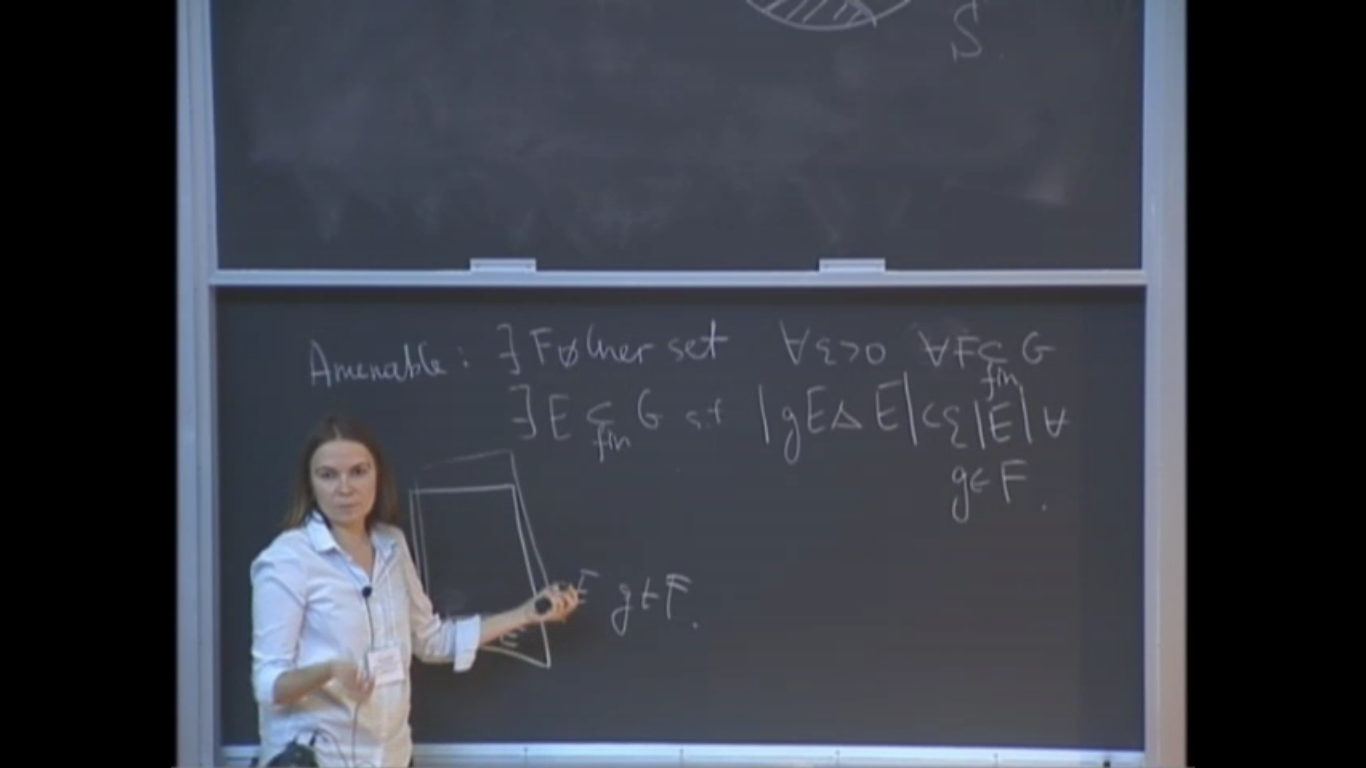Glueing together copies of amenable groups
Presenter
December 8, 2016
Keywords:
- sofic groups
- higman group
- amenability
- a-T-menability
- fixed point properties
- group cohomology
- Banach space
- hyperbolic groups and generalizations
- expander graph
- index theory
- non-commutative geometry
MSC:
- 20F65
- 11B37
- 43A07
- 19-xx
- 20-xx
- 43-xx
- 46-xx
- 57-xx
- 58-xx
- 43A07
- 20J06
- 46L80
Abstract
We suggest the beginning of a possible strategy towards finding a non-sofic group. In particular, we show that, if the Higman group were sofic, there would be a map from Z/pZ to itself, locally like an exponential map, satisfying a rather strong recurrence property. We also improve on existing bounds on the recurrence of exponential maps on Z/pZ. Our approach to (non)-soficity is based on the study of sofic representations of amenable subgroups of a sofic group.