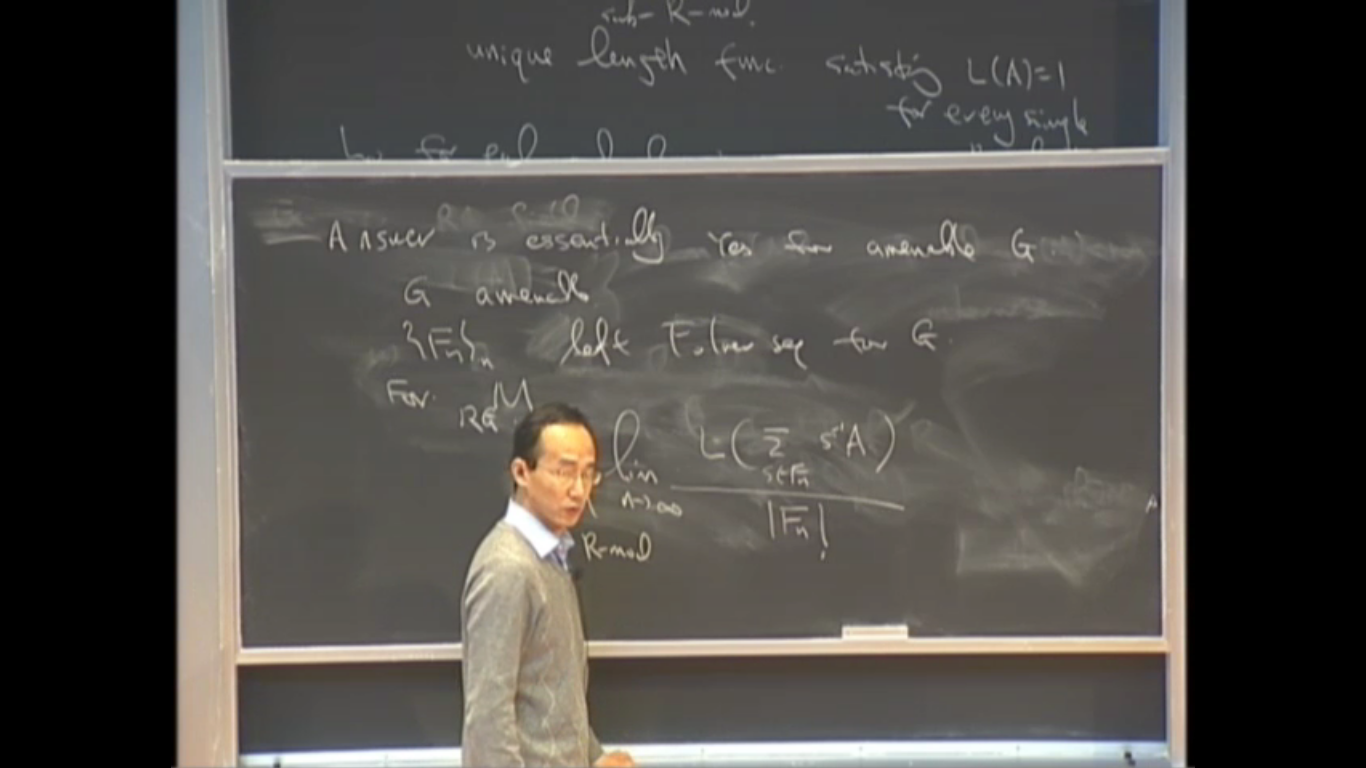For a unital ring R, a length function on left R-modules assigns a (possibly infinite) nonnegative number to each module being additive for short exact sequences of modules. For any unital ring R and any group G, one can form the group ring RG of G with coefficients in R. The modules of RG are exactly R-modules equipped with a G-action. I will discuss the question of how to define a length function for RG-modules, given a length function for R-modules. An application will be given to the question of direct finiteness of RG, i.e. whether every one-sided invertible element of RG is two-sided invertible. This is based on joint work with Bingbing Liang