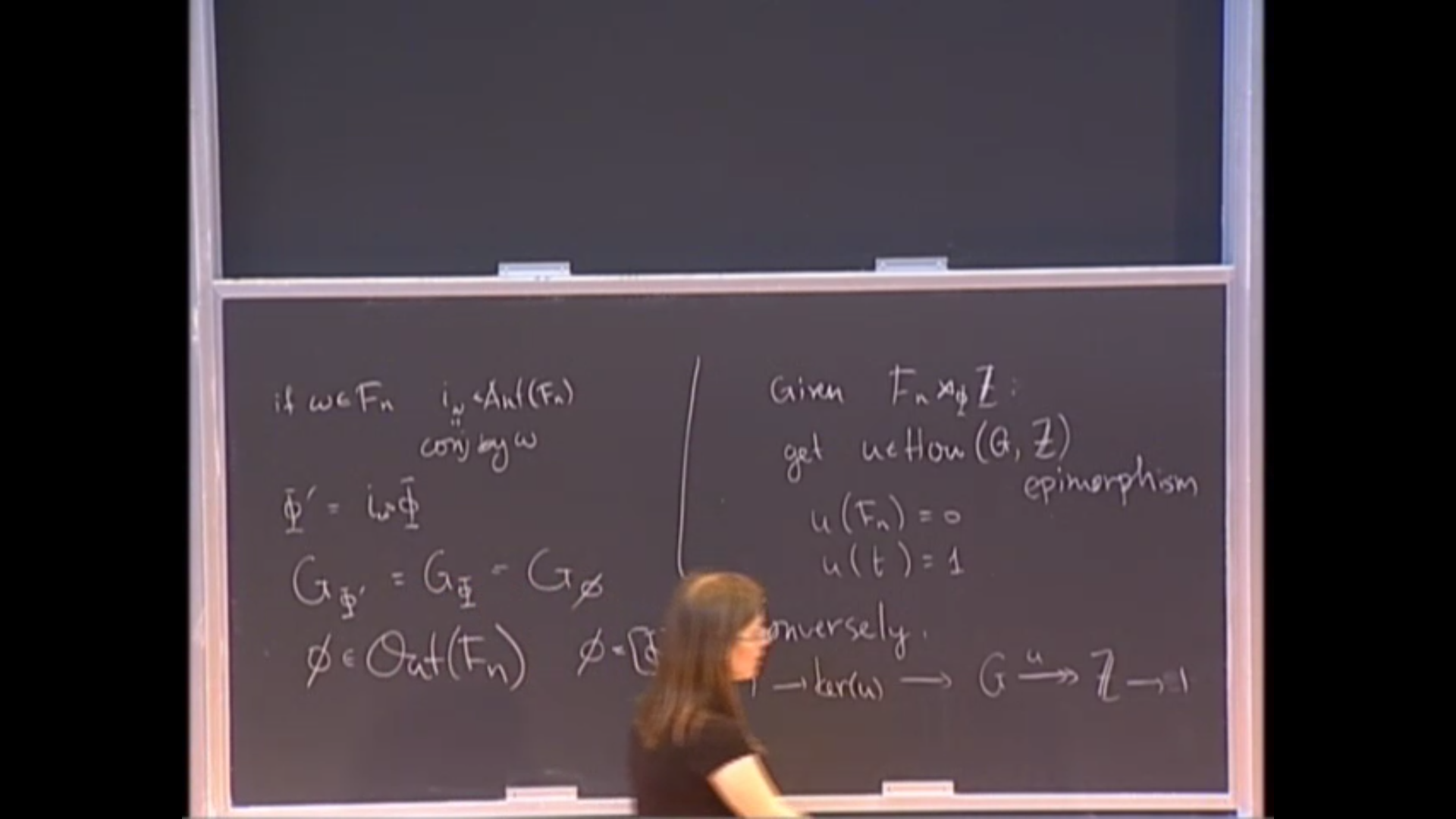Fiberations of free-by-cyclic groups
Presenter
August 19, 2016
Keywords:
- automorphism groups
- Out(F_n)
- free-by-cyclic groups
- free groups
- mapping class group
- geometric group theory
- folded mapping torus
- semidirect products
- fibrations
- dilations
- train-track maps
- Dowdall-Kapovich-Leininger's construction
MSC:
- 20F65
- 20F67
- 20Fxx
- 20-xx
- 14Dxx
Abstract
There is a beautiful and well developed theory (due to Thurston, Fried and McMullen) classifying all of the fiberations over the circle of a given 3-manifold.
These fibrations have common characteristics in particular if one fibration has a monodromy that is pseudo-Anosov then all fibrations have this property, and the dilatations are related via an element in the group ring of the first homology of the manifold.
We will discuss a theory of fiberations of free-by-cyclic groups that was developed in analogy to the 3-manifold case.
In particular we will discuss Dowdall-Kapovich-Leininger's construction of an open cone of fiberations of a free-by-cyclic group, and their theorem that if the original outer automorphism was fully irreducible then the monodromy of each element in this cone is an irreducible train-track map.
We then describe a polynomial that packages all of the dilatations of all of these train-track maps (by joint work with Hironaka and Rafi).