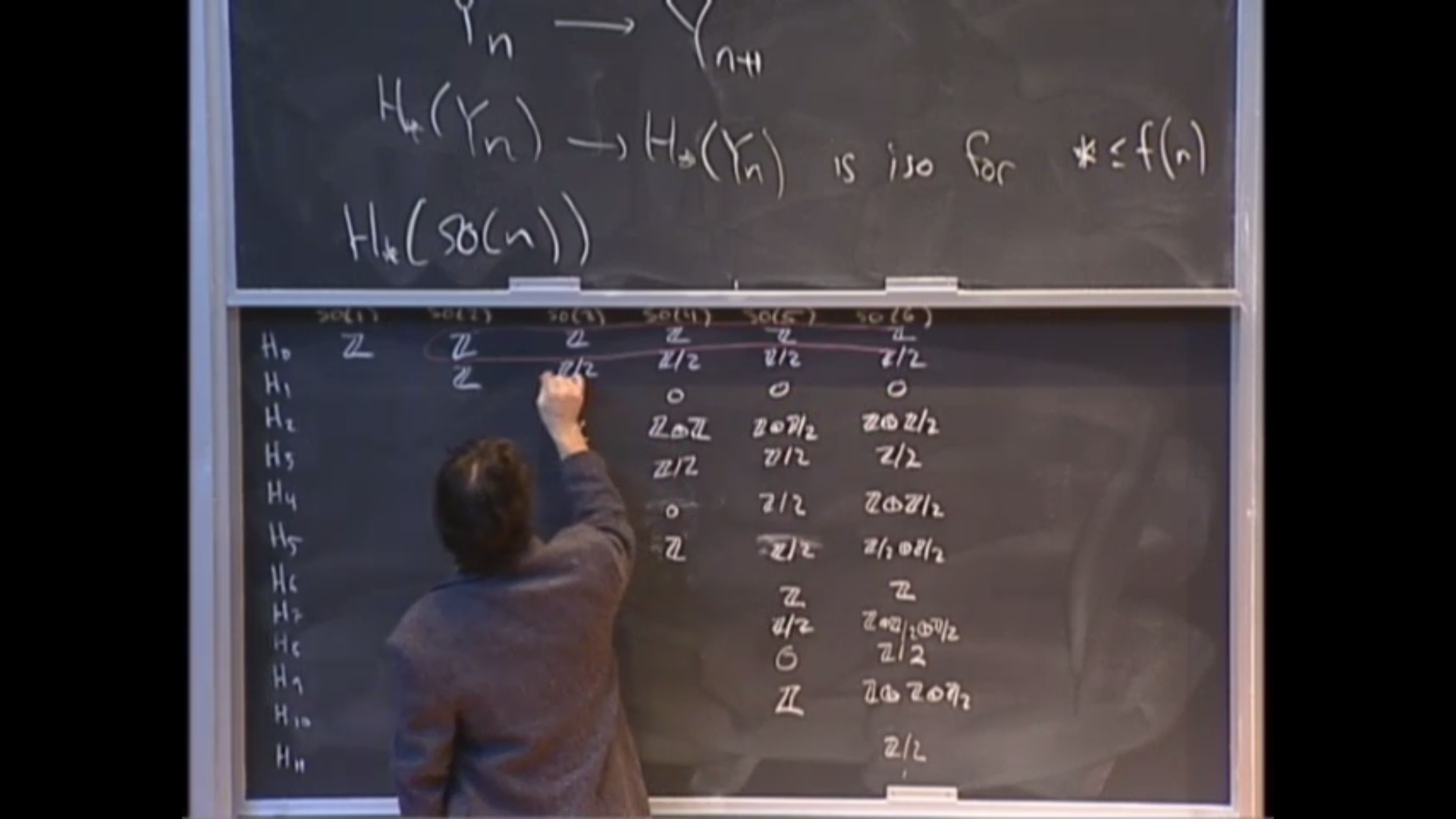Homological stability, representation stability, and FI-modules
Presenter
August 26, 2016
Keywords:
- geometric group theory
- classical Lie groups
- stable homotopy groups
- Church-Bestvina conjecture
- mapping spaces
- configuration space
- moduli spaces
- GL(n,Z)
- GL(n,R)
MSC:
- 20F65
- 20F67
- 20F05
- 20Fxx
- 20-xx
- 19A13
- 19B14
- 19B10
Abstract
Homological stability is the classical phenomenon that for many natural families of moduli spaces the homology groups stabilize. Often the limit is the homology of another interesting space; for example, the homology of the braid groups converges to the homology of the space of self-maps of the Riemann sphere. Representation stability makes it possible to extend this to situations where classical homological stability simply does not hold, using ideas inspired by asymptotic representation theory. I will give a broad survey of homological stability and a gentle introduction to the tools and results of representation stability, focusing on its applications in topology.