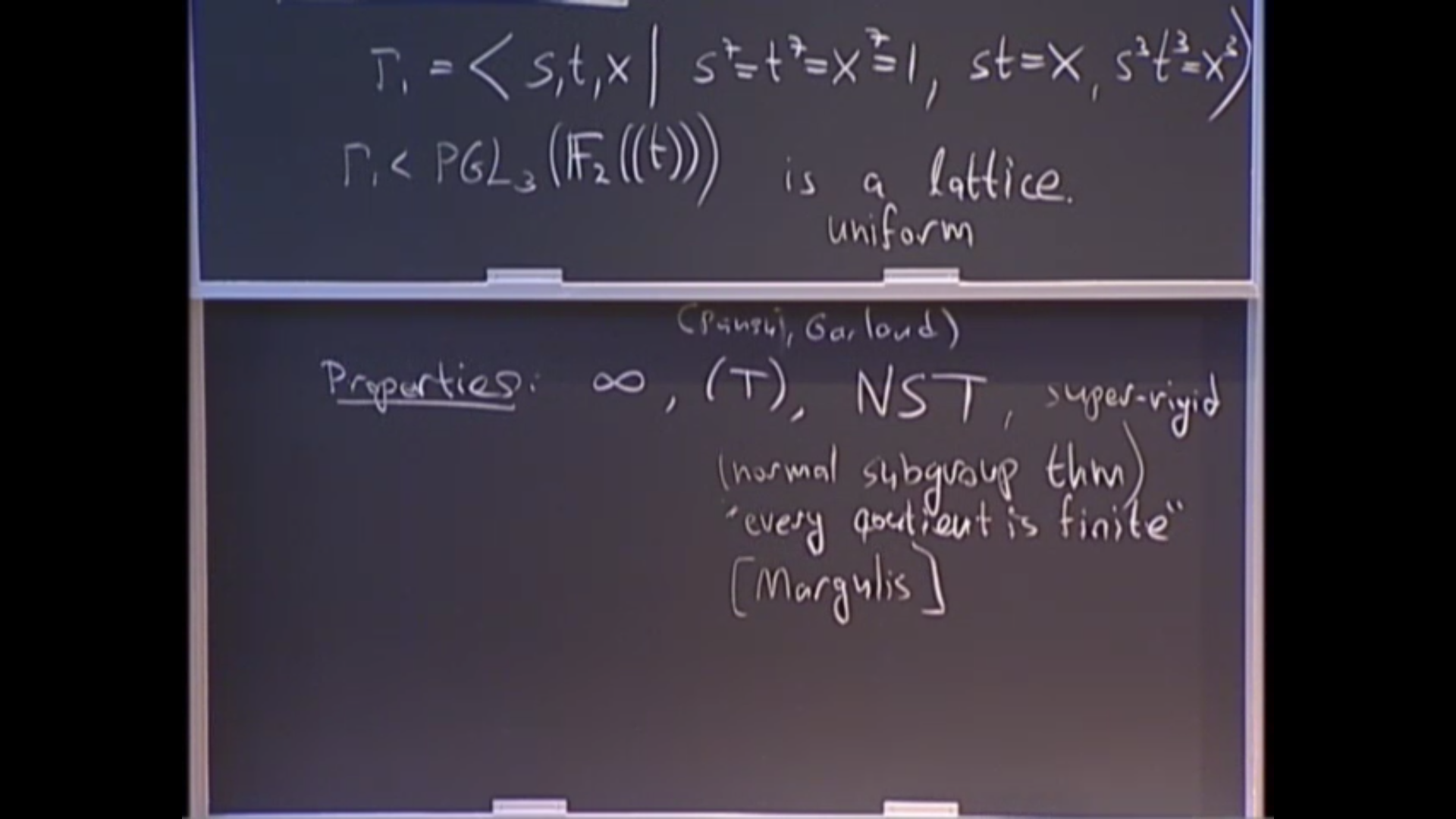One of the most prominent class of CAT(0) spaces is the class of Affine Buildings. In dimension 1, an affine building is nothing but a tree. In dimension 3 and higher (irreducible) affine buildings are always classical, that is they are the Bruhat-Tits buildings of algebraic groups over valued fields. In dimension 2 there are loads of exotic (ie, non-classical) buildings. Some are intimately related with some sporadic finite simple groups. Many have a cocompact group of isometries.