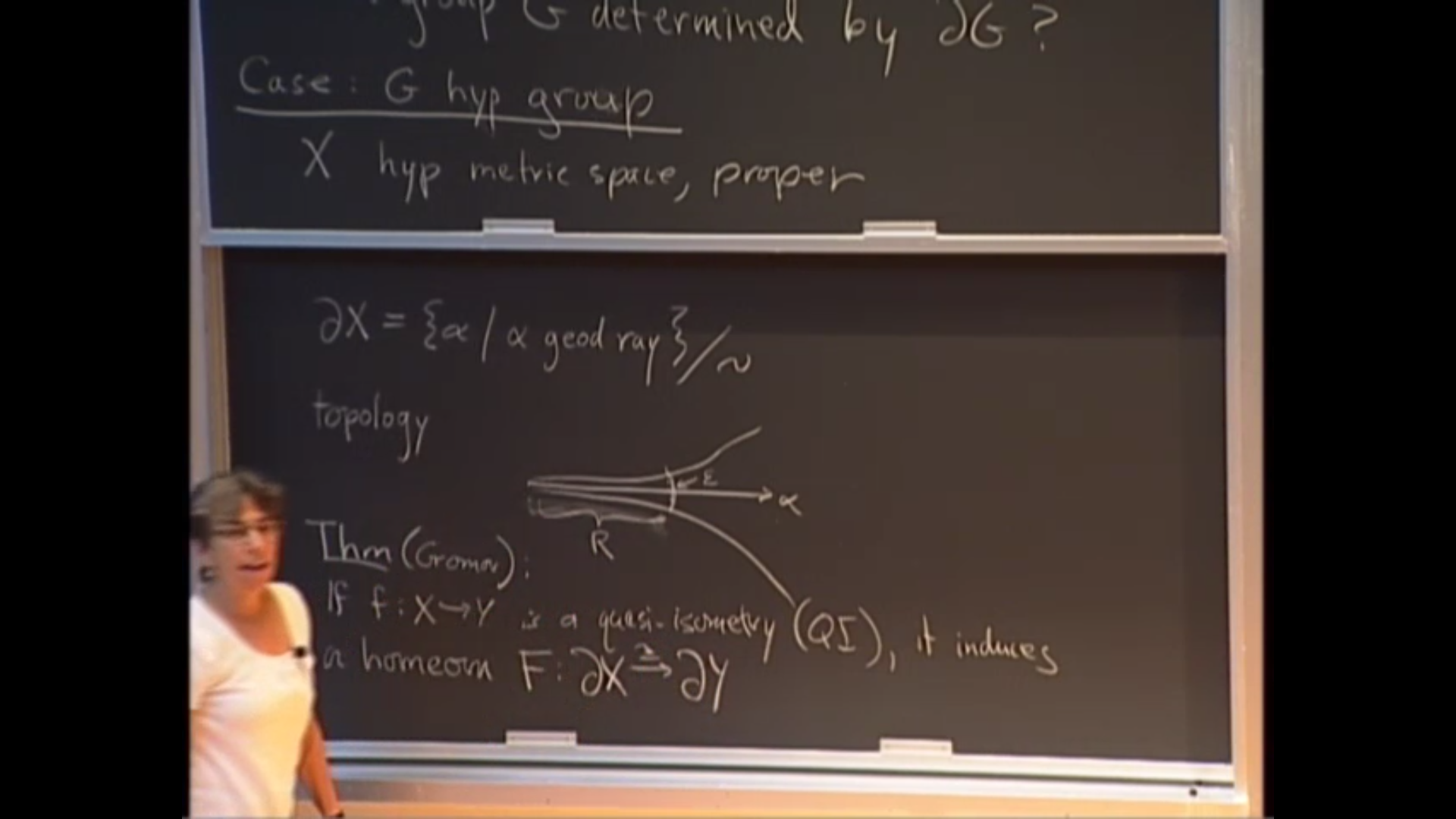Quasi-mobius maps between Morse boundaries of CAT(0) spaces
Presenter
September 29, 2016
Keywords:
- CAT(0) space
- negative curvature manifolds
- Riemannian geometry
- visual boundary
- Morse boundary
MSC:
- 57M60
- 57-xx
- 58-xx
- 58Dxx
- 58D05
- 58D19
- 58E05
- 58E09
- 58E10
- 58Exx
- 58E40
Abstract
The Morse boundary of a geodesic metric space is a topological space consisting of equivalence classes of geodesic rays satisfying a Morse condition. A key property of this boundary is quasi-isometry invariance: a quasi-isometry between two proper geodesic metric spaces induces a homeomorphism on their Morse boundaries. In the case of a hyperbolic metric space, the Morse boundary is the usual Gromov boundary and Paulin proved that this boundary, together with its quasi-mobius structure, determines the space up to quasi-isometry. I will discuss an analogue of Paulin’s theorem for Morse boundaries of CAT(0) spaces. This is joint work with Devin Murray.