30 Years of Calderón's Problem I
Presenter
August 23, 2010
Keywords:
- inverse problems
- scattering theory
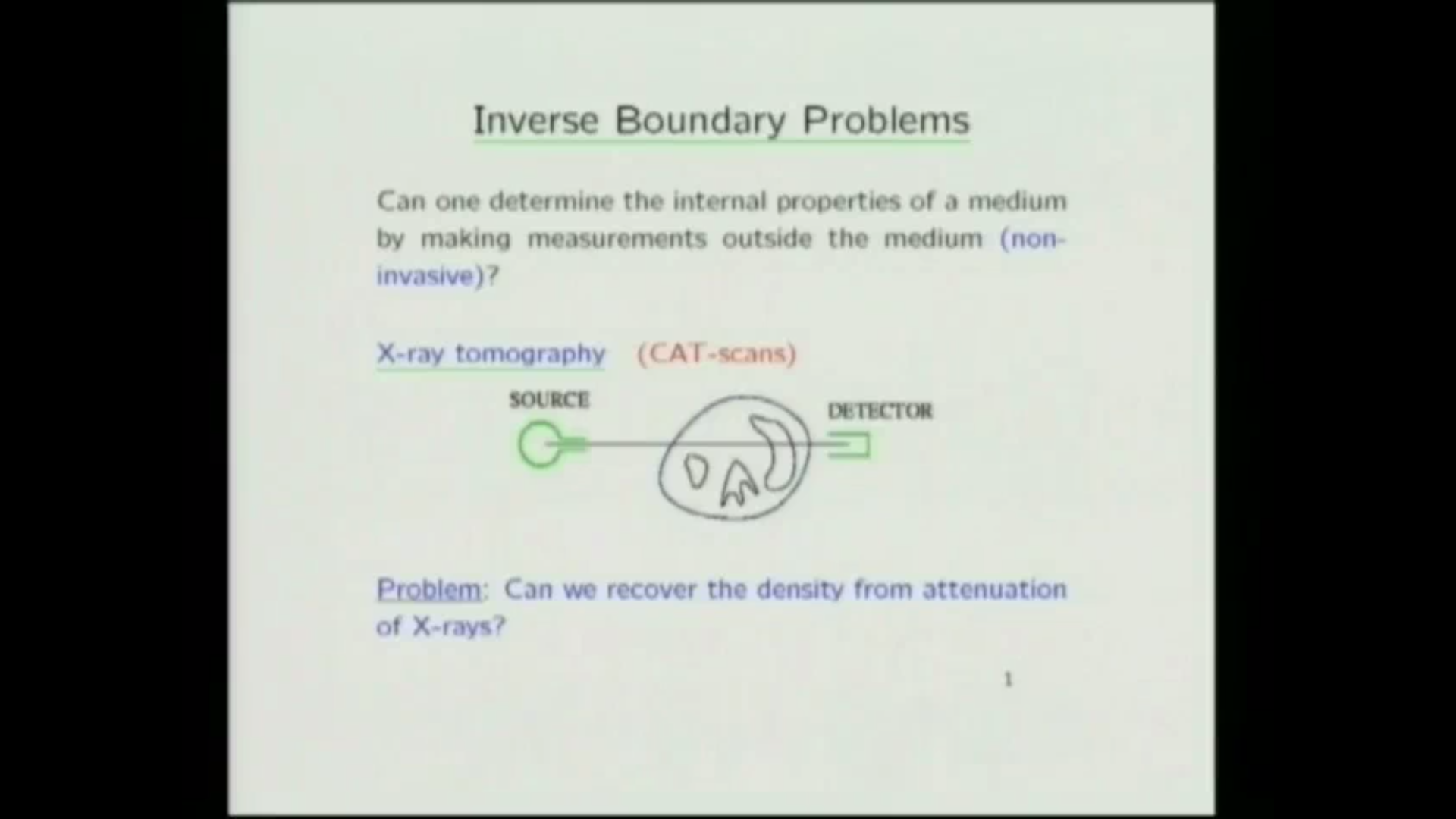
- electrical impedance tomography
- geometric optics
- boundary problems
MSC:
- 35R30
- 35R25
- 35Rxx
- 35-xx
- 35A27
- 35A30
- 35A30
Abstract
In 1980 Calderón published a short paper [3] in which he pioneered the mathematical study of the inverse problem of determining the conductivity of a medium by making voltage and current measurements at the boundary. This inverse method is also called Electrical Impedance Tomography. There has been fundamental progress made on this problem, which is now called Calderón’s problem, during the following thirty years but several fundamental questions remain unanswered. For a recent survey see [8]. We will consider some of the most important development concentrating in applications of complex geometrical optics. We will follow the notes of Mikko Salo and also discuss the two dimensional case [2], [7]. We will also consider counterexamples to uniqueness to Calder´on’s problem which are relevant for cloaking and invisibility [4],[5]. Time permitting we will give an application of complex geometrical optics to photoacoustics[1]. This inverse problem will be discussed in Peter Kuchment’s minicourse.