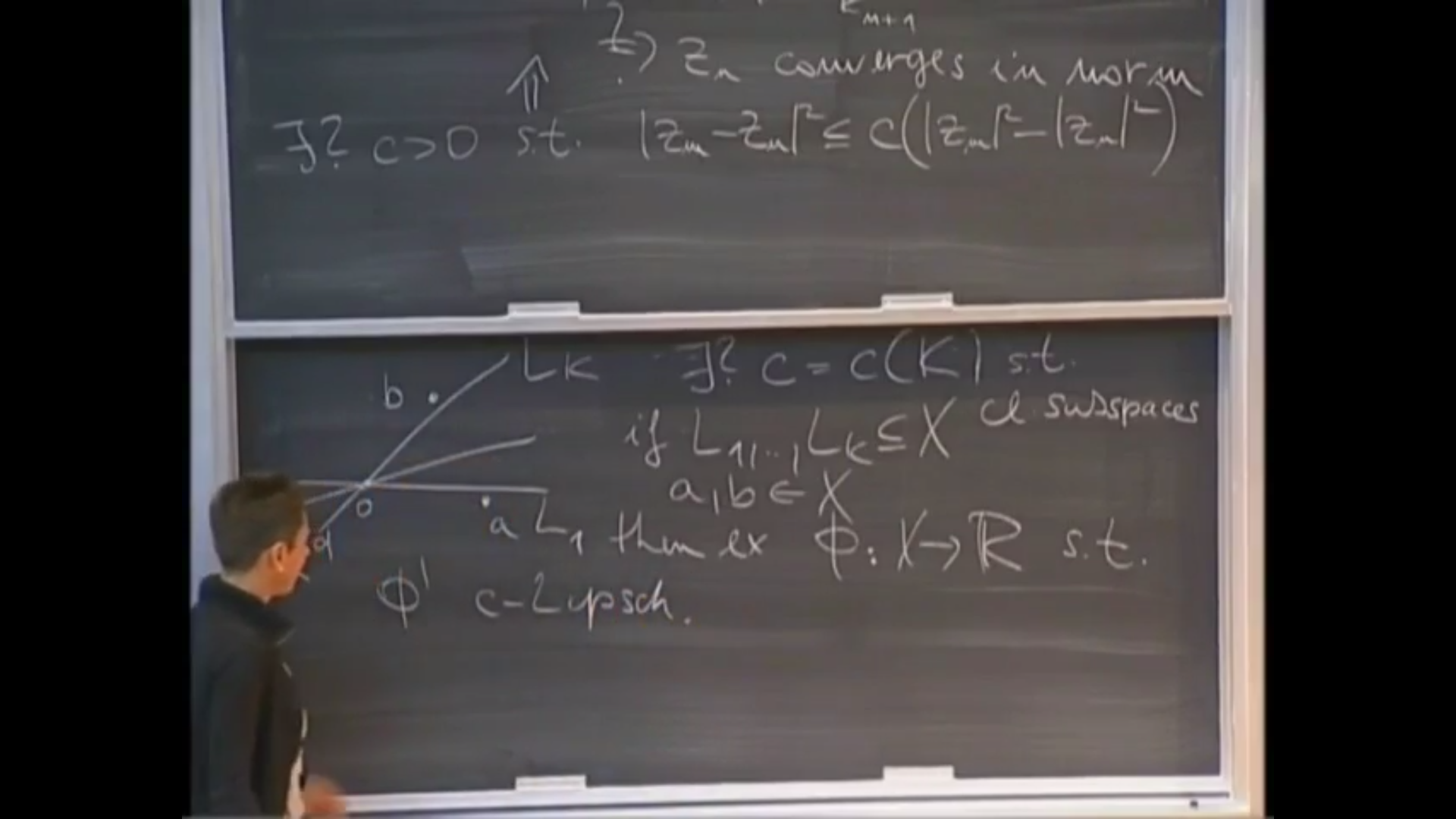Products of Projections II
Presenter
August 19, 2011
Keywords:
- quantitative geometry
- embedding theorems
- random sequences of symmetries
- Banach space
- orthogonality
MSC:
- 47A46
- 47Axx
- 47-xx
- 46-xx
- 46Cxx
- 46C07
Abstract
Let X and Y be two closed subspaces of a Hilbert space. If we send a point back and forth between them by orthogonal projection, the iterates converge to the projection of the point on the intersection of X and Y. We will investigate when a sequence of orthoprojections of a point in a Hilbert space on a finite family of closed subspaces, or more generally, closed convex subsets, converges.