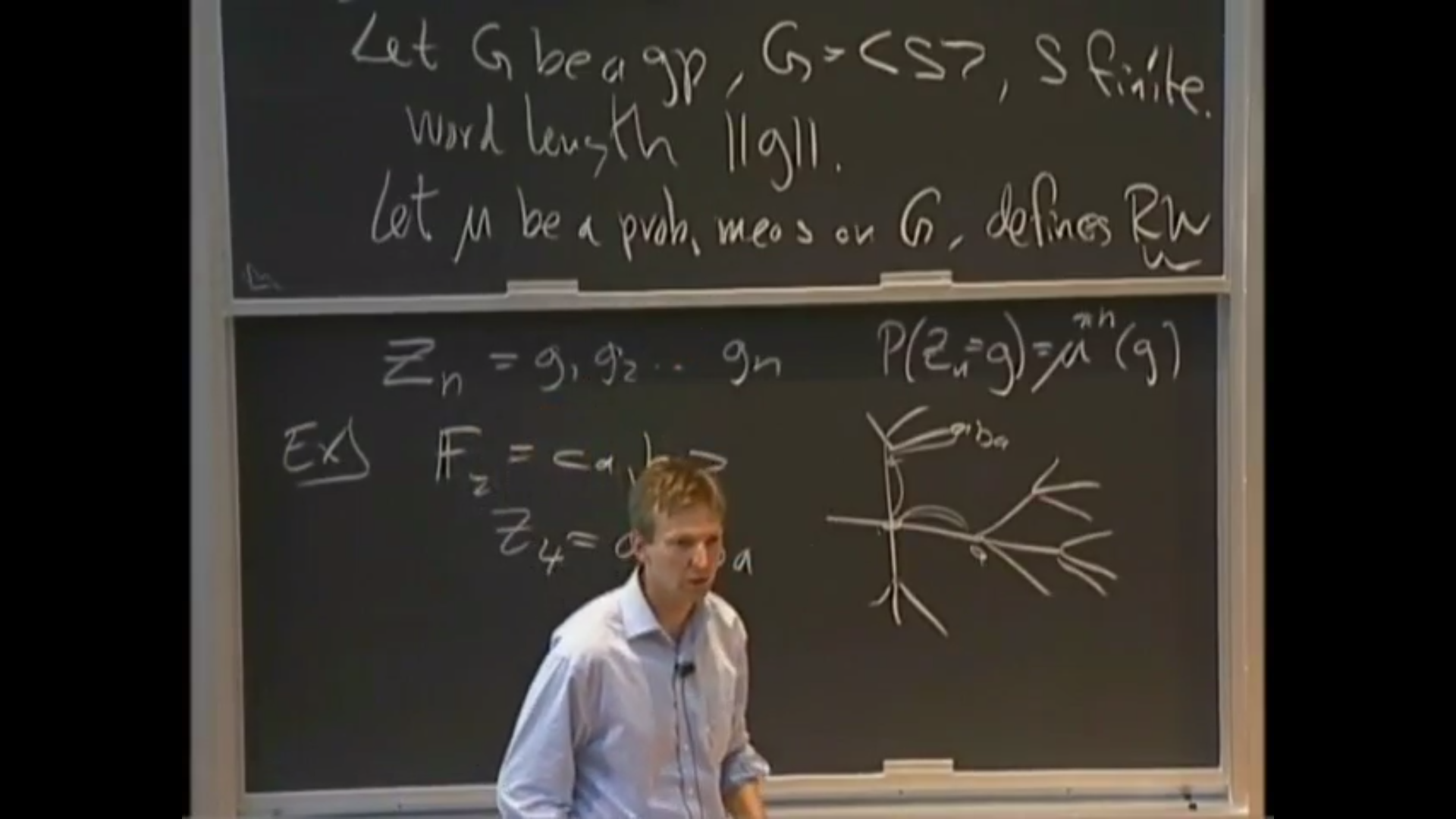Homomorphisms from Random Walks
Presenter
September 23, 2011
Keywords:
- geometric group theory
- geometric measure theory
- Cayley graphs
- quantitative geometry
- probability theory
- random walks
- entropy formulas
MSC:
- 60Gxx
- 60-xx
- 46-xx
- 46Bxx
- 46B06
- 46B09
- 46B20
- 46B80
Abstract
I will discuss a construction of homomorphisms from finitely generated groups G to the reals R coming from random walks on G. If the square of the drift grows faster than the entropy, one can get a nontrivial such homomorphism. One consequence is that if G lacks nontrivial homomorphisms to R or if the random walk is symmetric of finite second moment, then one has that the entropy must dominate the square of the drift, even in cases where the Varopoulos-Carne bounds are not available. Some further variants of this construction and consequences may be discussed. This is based on joint work with A. Erschler.