Optimal Gaussian Partitions
Presenter
September 22, 2011
Keywords:
- asymptotic combinatorics
- quantitative geometry
- probability theory
- Gaussian measure
- spherical partition problem
- isoperimetric inequalities
MSC:
- 60G15
- 60Gxx
- 60-xx
- 05C70
- 15B51
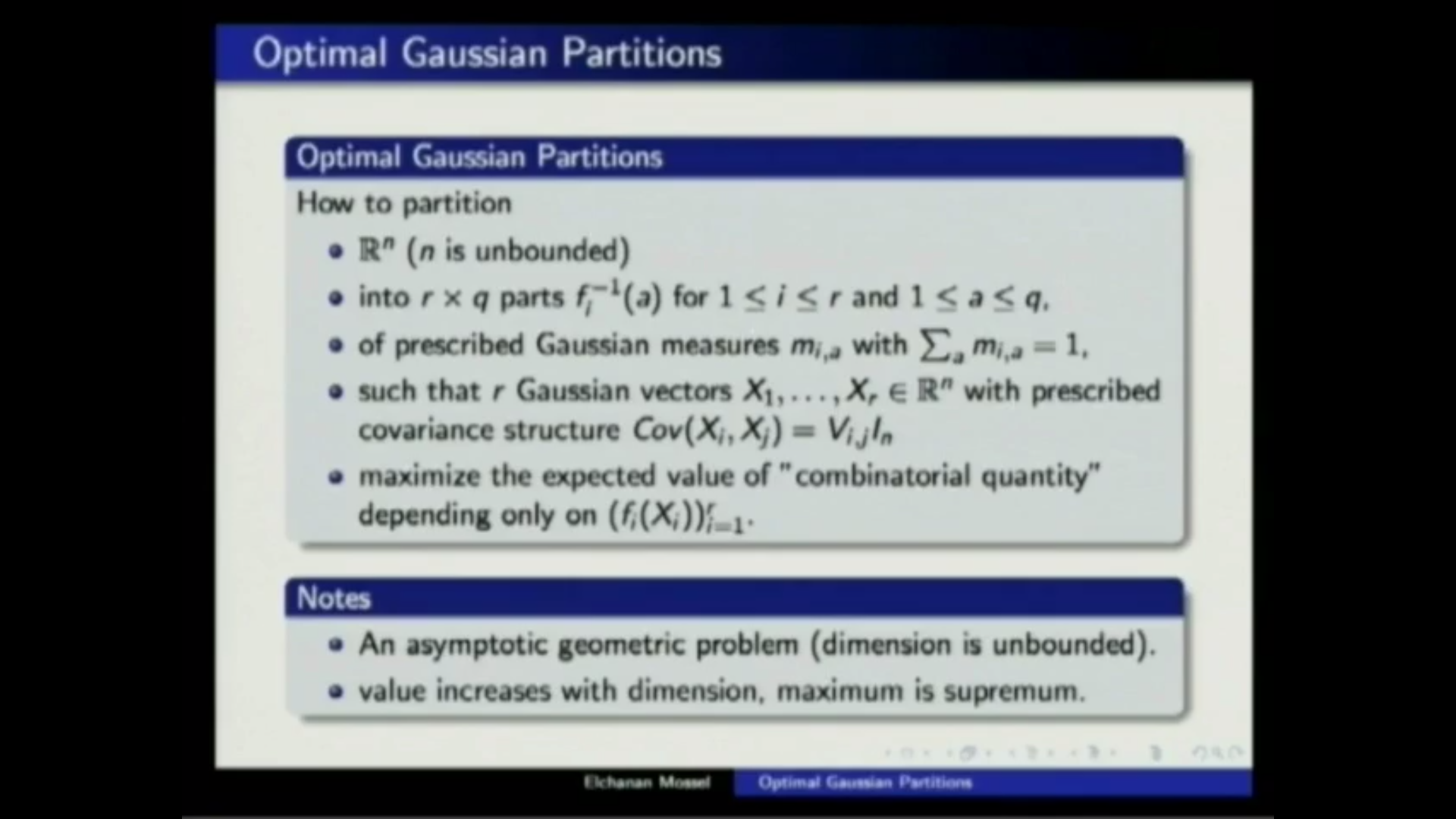
Abstract
Suppose X_1,...,X_k are n-dimensional Gaussian vectors with a given covariance structure. What is the partition of R^n into r sets of given Gaussian measures m_1,...,m_r which maximizes P[ (X_1,...,X_k) fall in the same part of the partition]? I will give an overview of what is known and conjectured about this question in various setups as well as various reasons for studying it and connections to classical isoperimetric problems.