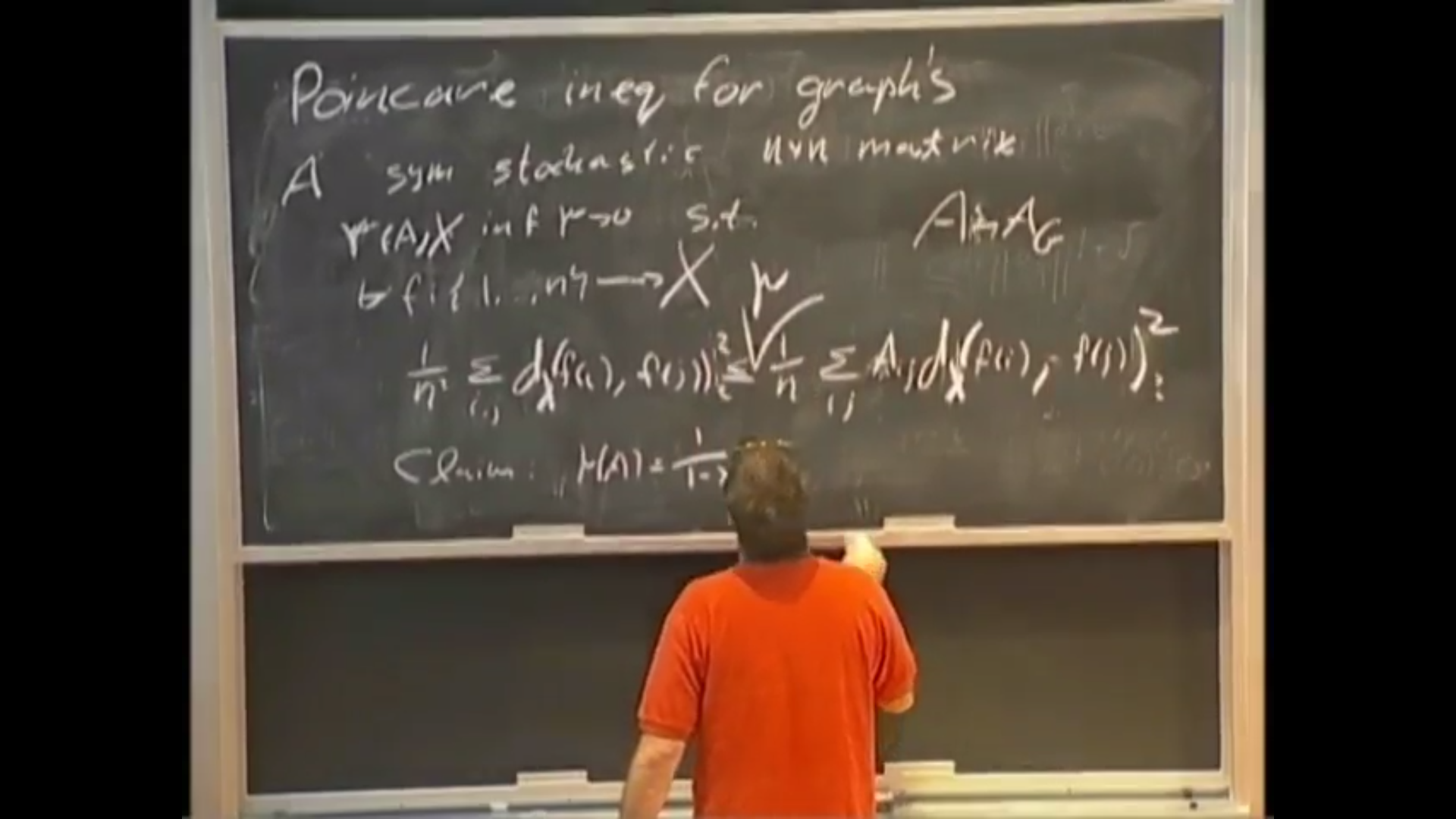The spectral gap of symmetric stochastic matrix A=(a_{ij}) is equal to the reciprocal of the smallest constant g in the associated Poincare-type inequality: For every mapping into the real line f:{1,...,n}-->R, the average over all pairs i,j of |f(i)-f(j)|^2 is bounded from above by (g/n) times \sum_{ij} a_{ij} |f(i)-f(j)|^2. Motivated by applications for proving non-embeddability results, one can consider more general (and stronger) inequalities in which the real line is replaced with another metric space X. The resulting constant g=g(A,X) is called (the reciprocal of) spectral gap of A with respect to X. We will discuss the behvoir of the spectral gap of powers of the matrices with respect to uniformly convex Banach spaces and CAT(0) spaces. Key inequalities in this investigation are Ball's Markov cotype inequality, and Pisier's Martingale cotype inequality. We will also mention recent applications of this theory: 1. Expander graphs having spectral gaps with rescpect to a. all uniformly convex Banach spaces, b. some CAT(0) spaces that also contains expanders, and c. the metric of constant degree random graphs. 2. Lipschitz extension theorems for CAT(0) codomains based on Ball's Lipshcitz extension theorem. Based on a joint work with Assaf Naor. Parts of it appeared in arXiv:0910.2041 and the rest is unpublished yet.