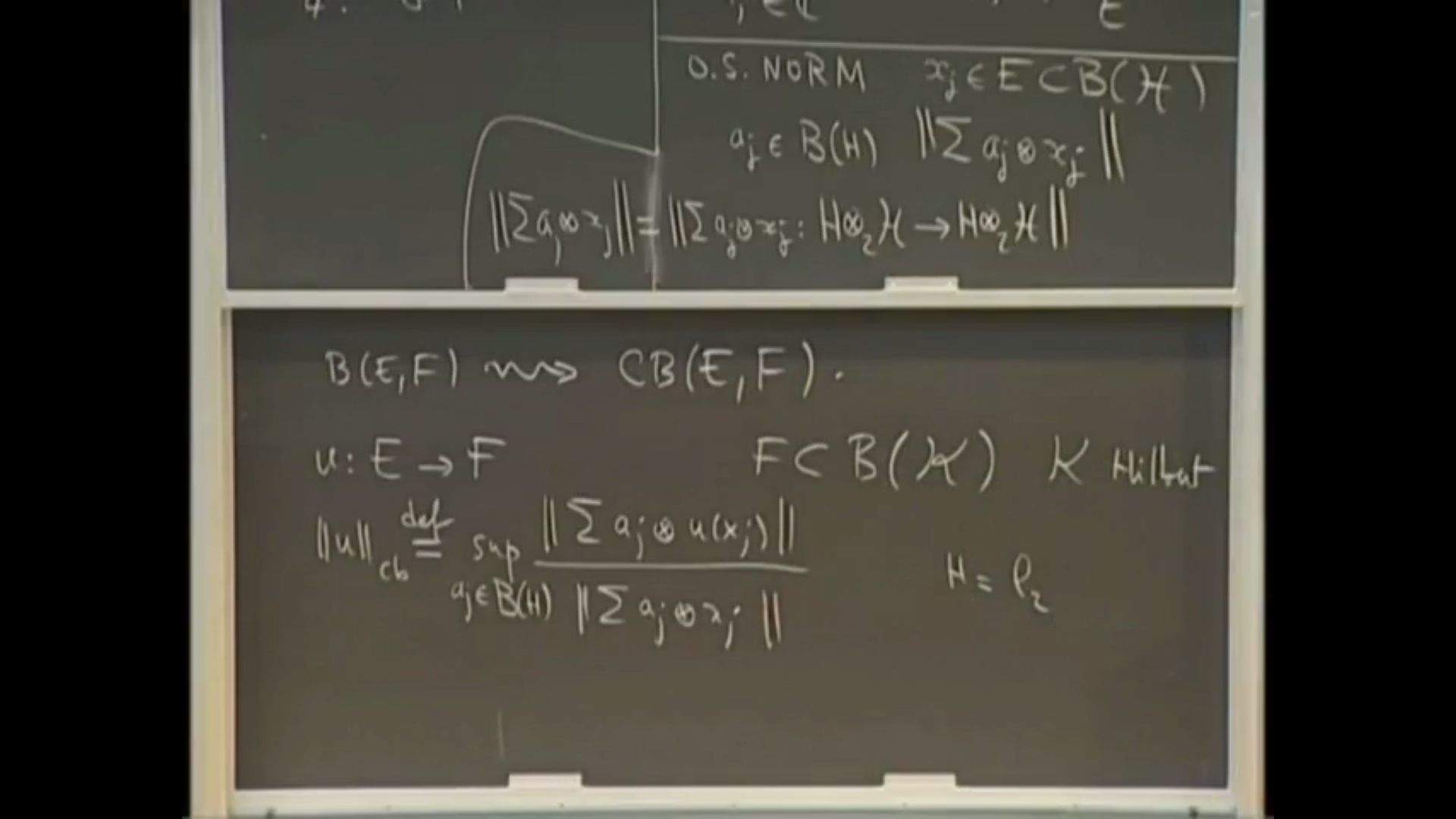We will explain the notion of exact operator space (a certain form of joint approximation property of operators by matrices) and show how it can be combined with properties of Gaussian random matrices to prove certain versions of Grothendieck’s factorization theorem for completely bounded bilinear maps on the product of two exact operator spaces. This is closely connected to previous joint work with Junge and Shlyakhtenko and also to work by Haagerup, Thorbjoernsen and Musat. We will also discuss a generalized form of exactness for which the factorization theorem still holds.