Extending Lipschitz Mappings Continuously
Presenter
October 21, 2011
Keywords:
- geometric group theory
- embedding theorems
- Banach space
- bounded operators
- extensions
MSC:
- 58C20
- 46-xx
- 46Bxx
- 46B20
- 26A16
- 54C25
Abstract
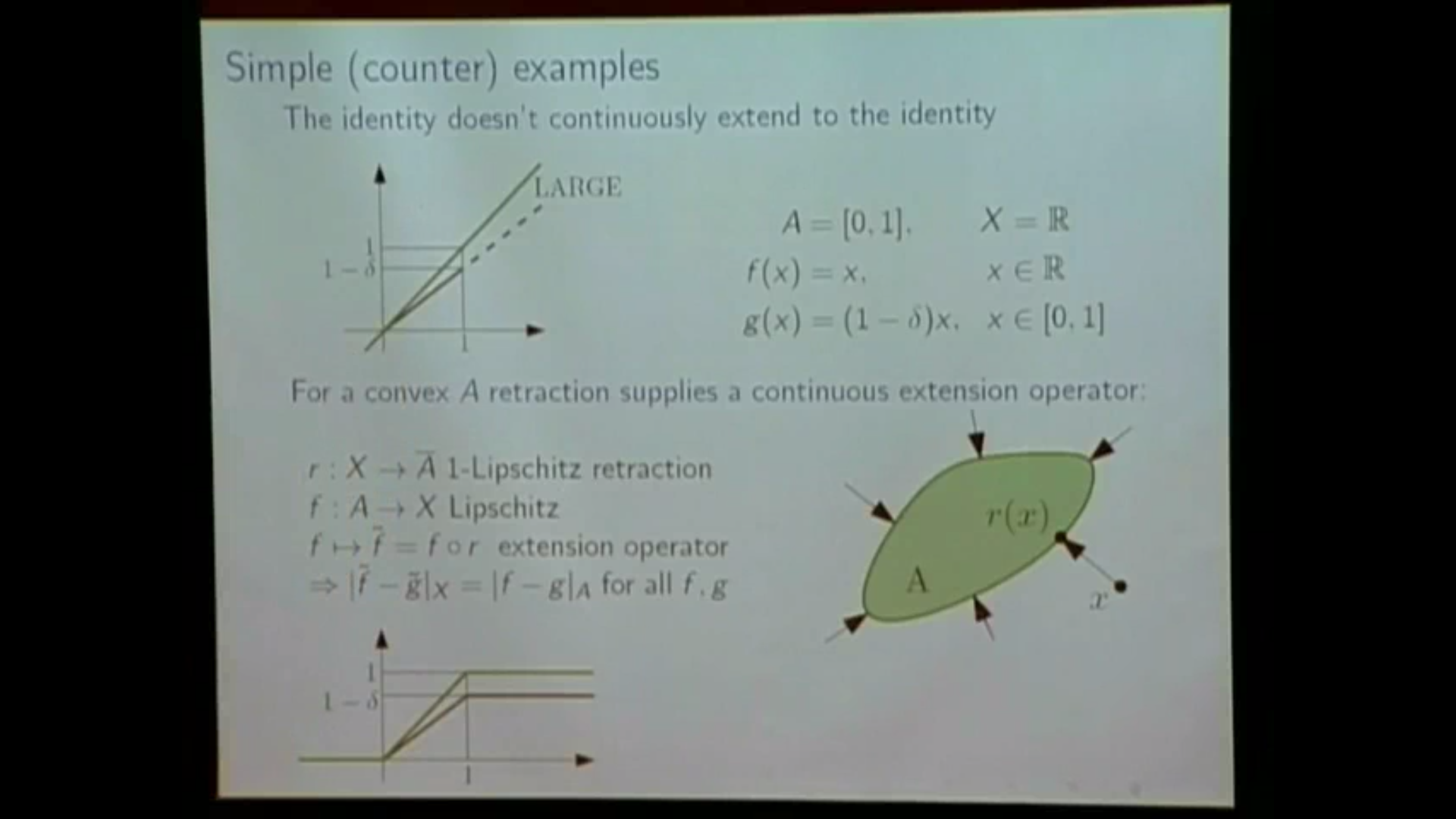
We consider the space L(K) of bounded contractive mappings from a subset K of a Hilbert H space into that space. By Kirszbraun's theorem, any contractive mapping can be extended to the entire space to be a contraction again. In general, the extension is not unique. We show that there are single-valued extension operators from L(K) to L(H) which are continuous in the supremum norm.
When K is a compact subset of a Euclidean space H, this implies that the restriction to K of the set of those Lipschitz isometries of H which, moreover, preserve the lengths of curves is residual in L(K).