Thin Sets, Differentiability of Functions and Geometric Measure Theory
Presenter
October 20, 2011
Keywords:
- Banach space
- embedding theorems
- geometric group theory
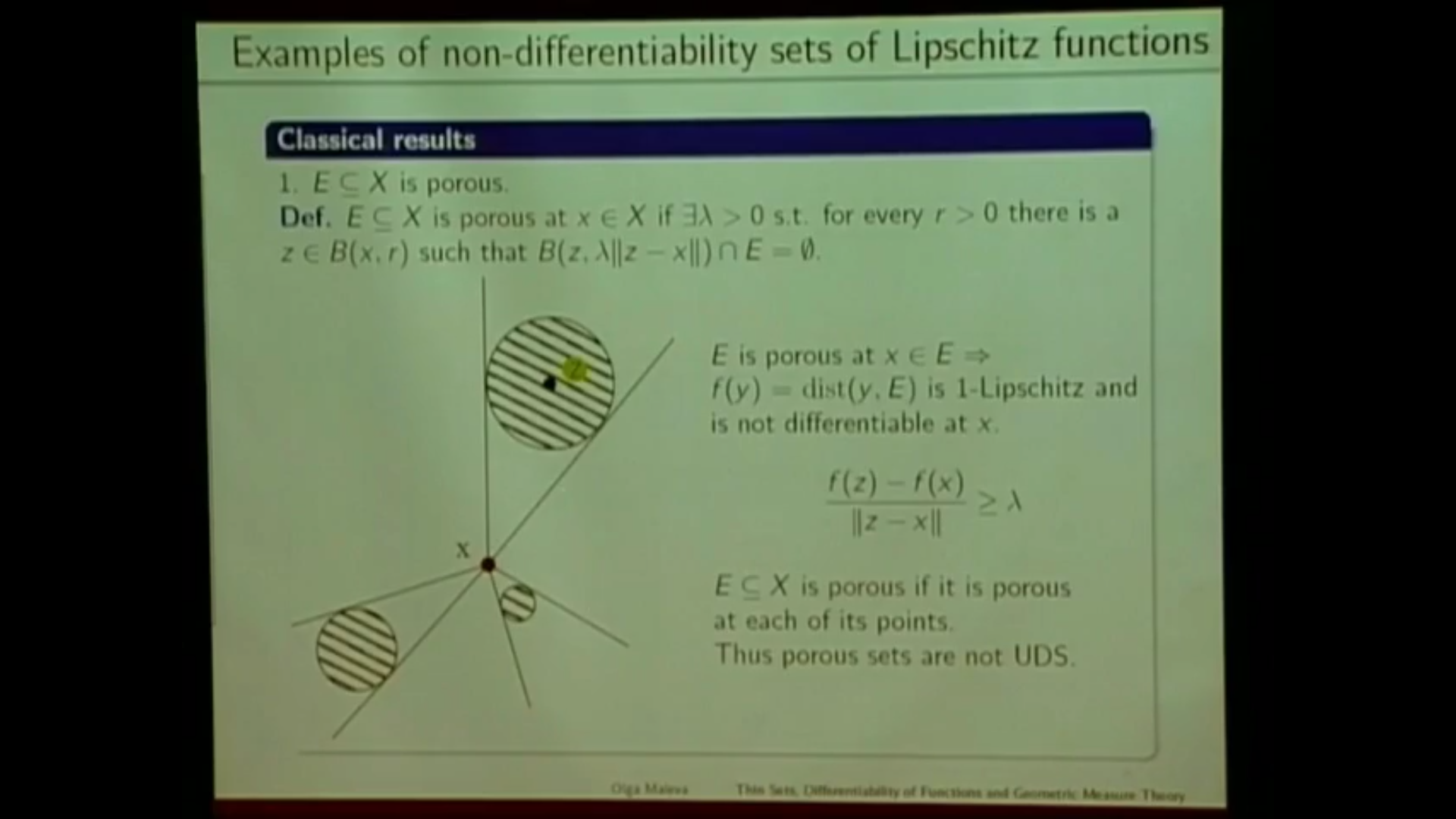
- geometric measure theory
- Lipschitz continuity
- Rademacher's theorem
- universal examples
- Hausdorff dimension
MSC:
- 58C20
- 46-xx
- 46Bxx
- 46B20
- 46B22
- 54C25
- 26A16
Abstract
We discuss universal differentiability sets (those on which every Lipschitz function must be differentiable at some point) and show that in a space with separable dual every open ball contains a closed universal differentiability subset of Hausdorff dimension 1. Moreover, this subset can be chosen to be totally disconnected. We show how the methods used in the construction of such sets may lead to a solution of a long‐standing open problem in the geometric measure theory.
The talk is based on joint work with M. Dore.