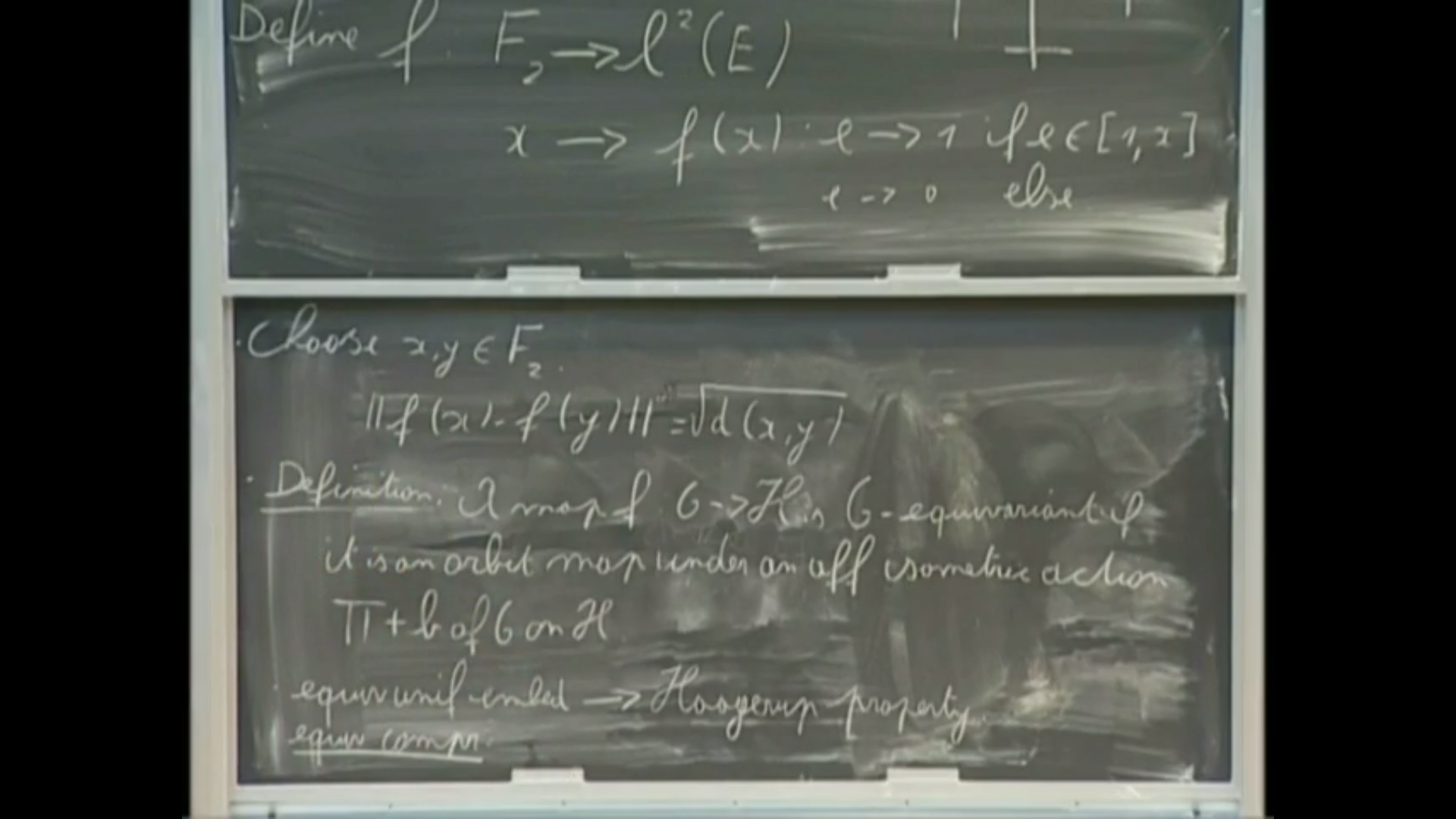Let H be a finitely generated group equipped with the word length metric relative to a finite symmetric generating subset. Uniform embeddability of H into a Hilbert space is an interesting notion since it implies e.g. that H satisfies the coarse Baum-Connes Conjecture [2]. The Hilbert space compression of a group indicates how well a certain group embeds uniformly into a Hilbert space. Here, there are connections with Yu's property (A) [1]. More precisely, the Hilbert space compression of a finitely generated group G is a number between 0 and 1 that describes how close a uniform embedding f: G to ell^2(Z) can be to being quasi-isometric. If this number is strictly greater than 1/2, then the group satisfies Yu's property (A) [1]. The equivariant Hilbert space compression only takes into account those uniform embeddings which are G-equivariant relative to some affine isometric action of G on ell^2(Z) and the left multiplication action of G on itself. If this number is strictly greater than 1/2, then the group is amenable [1]. We elaborate on the behavior of the (equivariant) Hilbert space compression under group constructions such as the free product, certain group extensions (e.g. by groups of polynomial growth or hyperbolic groups), and so forth.