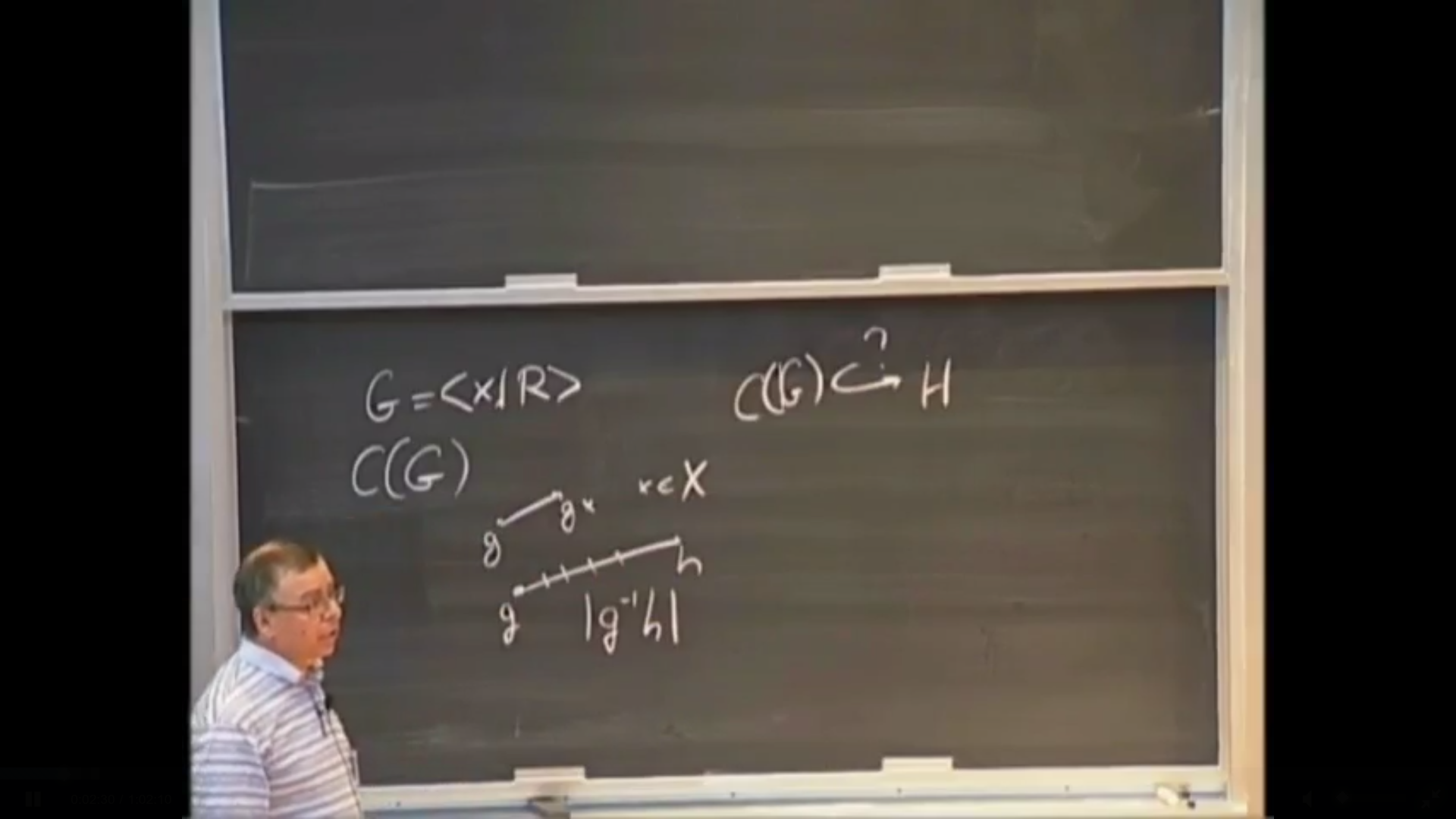We prove that every aspherical recursively presented group embeds into a group with finite aspherical presentation complex. By results of Gromov and Davis, this implies that there exists a closed aspherical manifold of any dimension >3 (smooth in dimension >4) with universal cover of infinite asymptotic dimension, and not embeddable uniformly into a Hilbert space. It is also a counterexample to the Baum-Connes conjecture with coefficients.