Finsler Geometry and Dynamics
Presenter
November 2, 2011
Keywords:
- Shiing-Shen Chern
- differential geometry
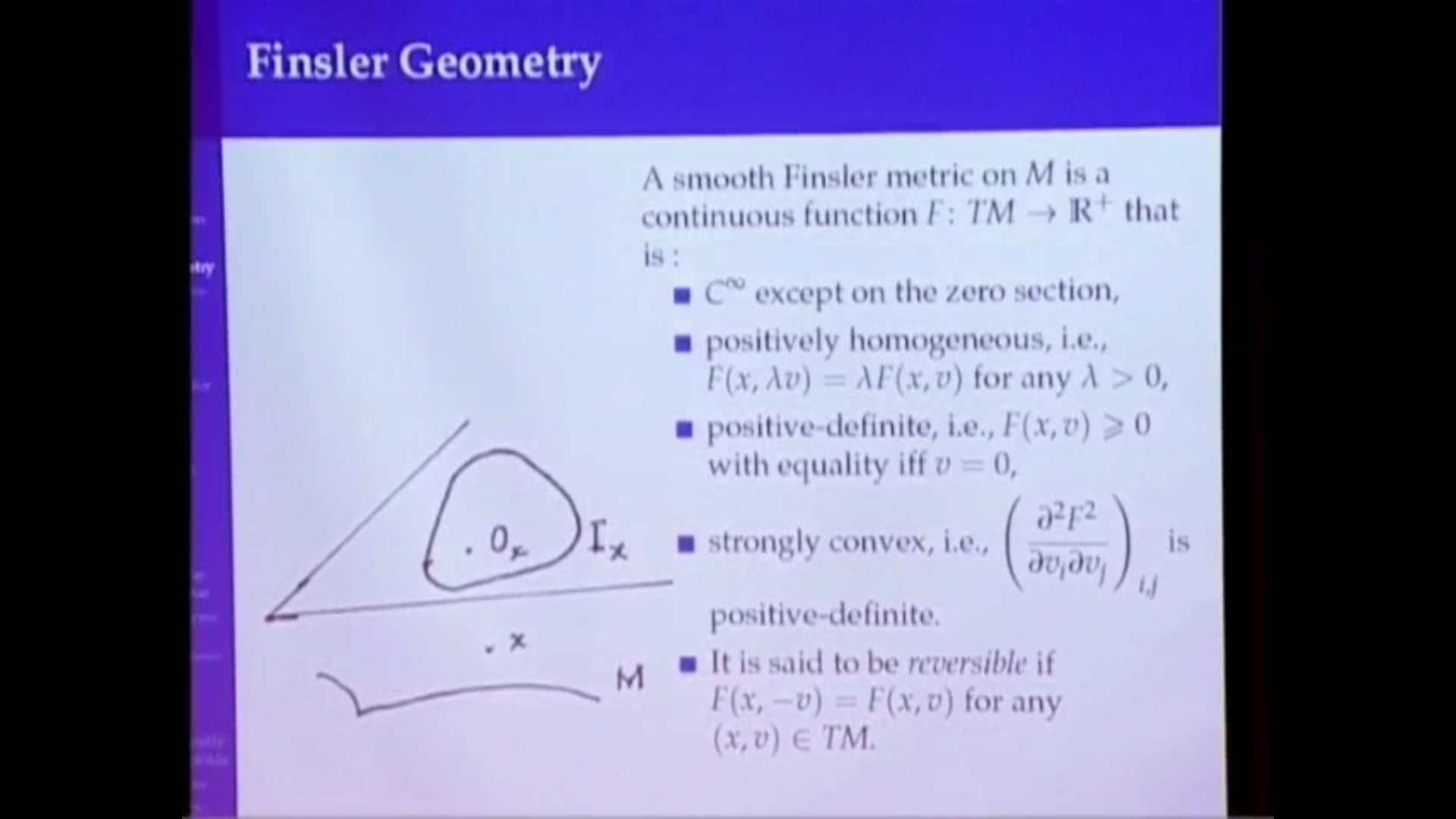
- Finsler spaces
- Laplacians
- complex manifolds
- complex dynamics
- spectrum of Laplacian
MSC:
- 53-xx
- 53D40
- 53C60
- 53C65
- 53C21
- 53Cxx
- 37-XX
- 37FXX
Abstract
We will investigate the geometry of some typical examples of Finsler structures such as Katok-Ziller metrics and Hilbert geometries. Katok-Ziller metrics on spheres are prototypes of smooth non- reversible metrics of constant positive flag curvature (boat sailing metrics). Following Thomas Barthelme's work we introduce a new solid angle associated with the Holmes-Thompson volume. An immediate byproduct is the existence of a linear natural Finsler Laplace operator. I shall present some of Barthelme's results concerning the spectrum of these metrics and emphasize the interplay between the lambda_1 and the Finsler volume. I shall also comment briefly on the existence of harmonic measures for negatively curved smooth Finsler metrics. Hilbert geometry and some quotients, the co-compact convex projective structures, are very nice examples of reversible non-smooth Finsler metrics for which the idea of extending the construction of a part of Chern's connection dynamically turns out to be very fruitful. To show this, I shall report on work by Mickael Crampon which gives a sharp estimate of the volume entropy and thus provides a very neat approach for the counting problem of closed geodesics.