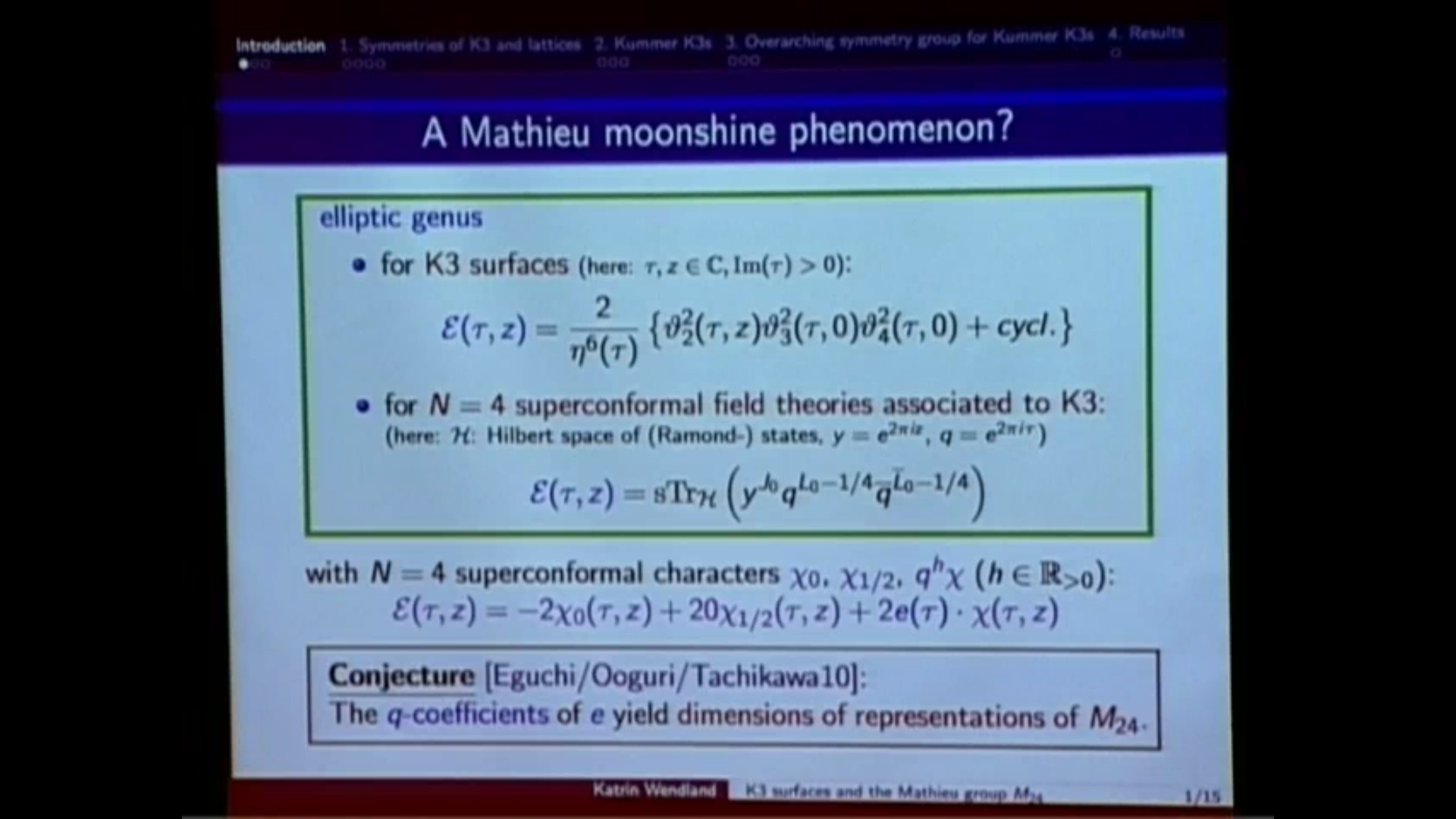
By classical results due to Nikulin, Mukai, Xiao and Kondo in the 1980's and 90's, the finite symplectic automorphism groups of K3 surfaces are always subgroups of the sporadic simple group known as the Mathieu group M_{24}. More precisely, there are eleven "maximal" such subgroups of M_{24}, which were also determined by Mukai, such that every finite symplectic automorphism group of a K3 surface is contained in one of these eleven groups as a subgroup. However, each of these eleven groups is by orders of magnitude smaller than M_{24}. Moreover, according to a recent observation by Eguchi, Ooguri and Tachikawa, the elliptic genus of K3 surfaces seems to contain a mysterious footprint of M_{24}: If one decomposes the elliptic genus into irreducible characters of the N=4 superconformal algebra, which is natural in view of superconformal field theories associated to K3, then the coefficients of the so-called non- BPS characters coincide with the dimensions of representations of M_{24}. In this talk, I will attempt to give an overview of these classical and recent developments, concerning K3 surfaces and the Mathieu group M_{24}.