The method of equivalence was introduced by Elie Cartan in 1908 as a procedure for finding invariants of geometric structures under the actions of pseudogroups, which most commonly appear as subgroups of the diffeomorphism group of a manifold preserving some underlying geometric structure. The method was further developed and systematized over the course of the 20th century, and it has been applied in a great variety of contexts. 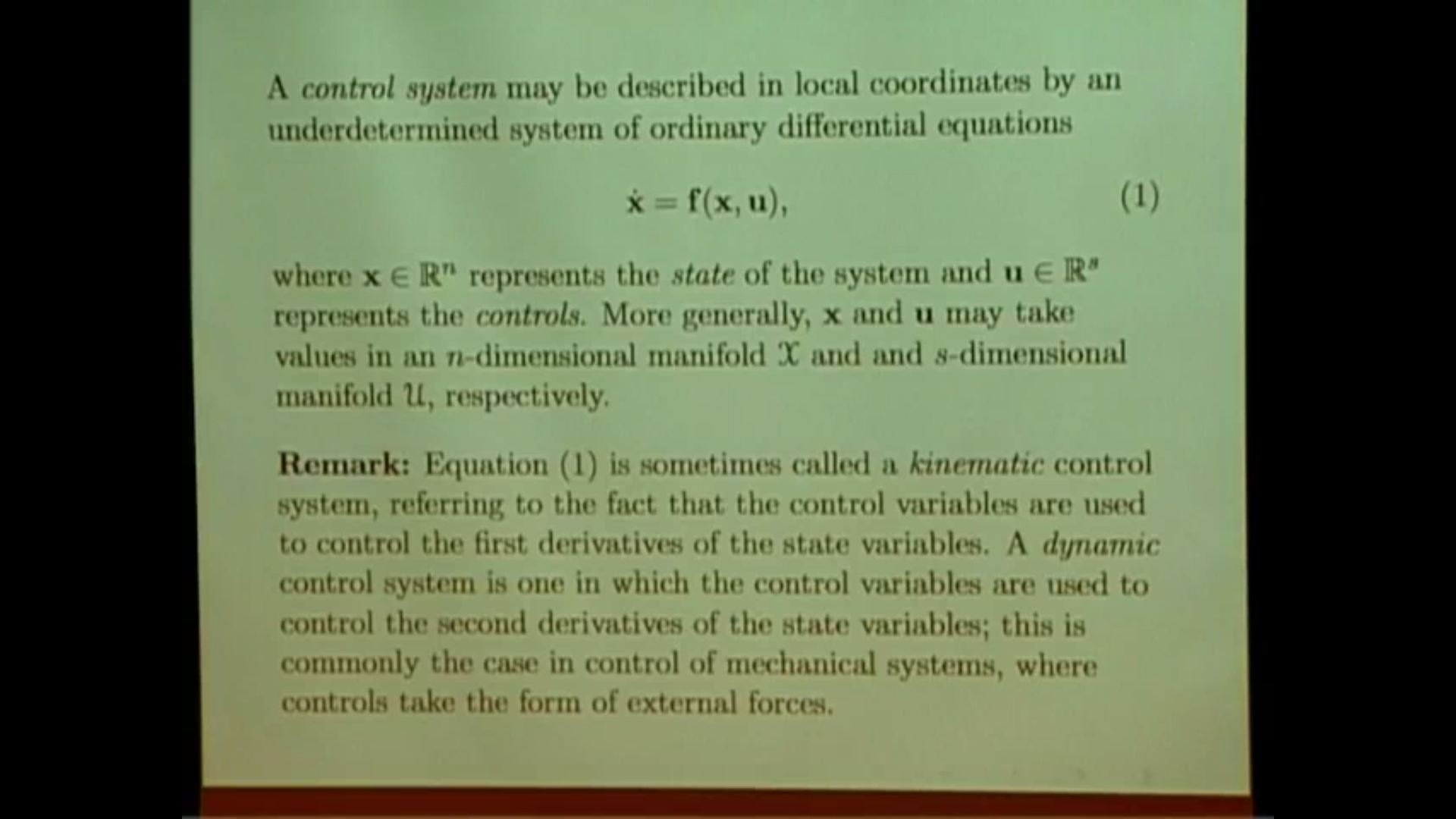After briefly demonstrating Cartan's method in the familiar context of Riemannian geometry, we will describe applications of the method of equivalence to some geometric structures related to control theory: 1) Sub-Riemannian geometry (Hughen, Moseley) and sub-Finsler geometry (C----, Moseley, and Wilkens), both of which involve metrics which are defined only on a distribution on a manifold (i.e., on a subbundle of the tangent bundle of the manifold). Such metrics appear naturally in the context of optimal control of "driftless" kinematic systems. 2) Affine distributions (C----, Moseley, and Wilkens), which appear naturally in the contexts of kinematic control systems with drift and dynamic control systems. 3) Dynamic equivalence of control systems (Stackpole). Dynamic equivalence generalizes the more thoroughly understood notion of static equivalence for control systems, which is addressed by the methods mentioned above. In his just-completed Ph.D. thesis, Stackpole shows how the method of equivalence may be used to address this more general notion of equivalence---which is defined in terms of submersions rather than diffeomorphisms---by extending to infinite prolongations.