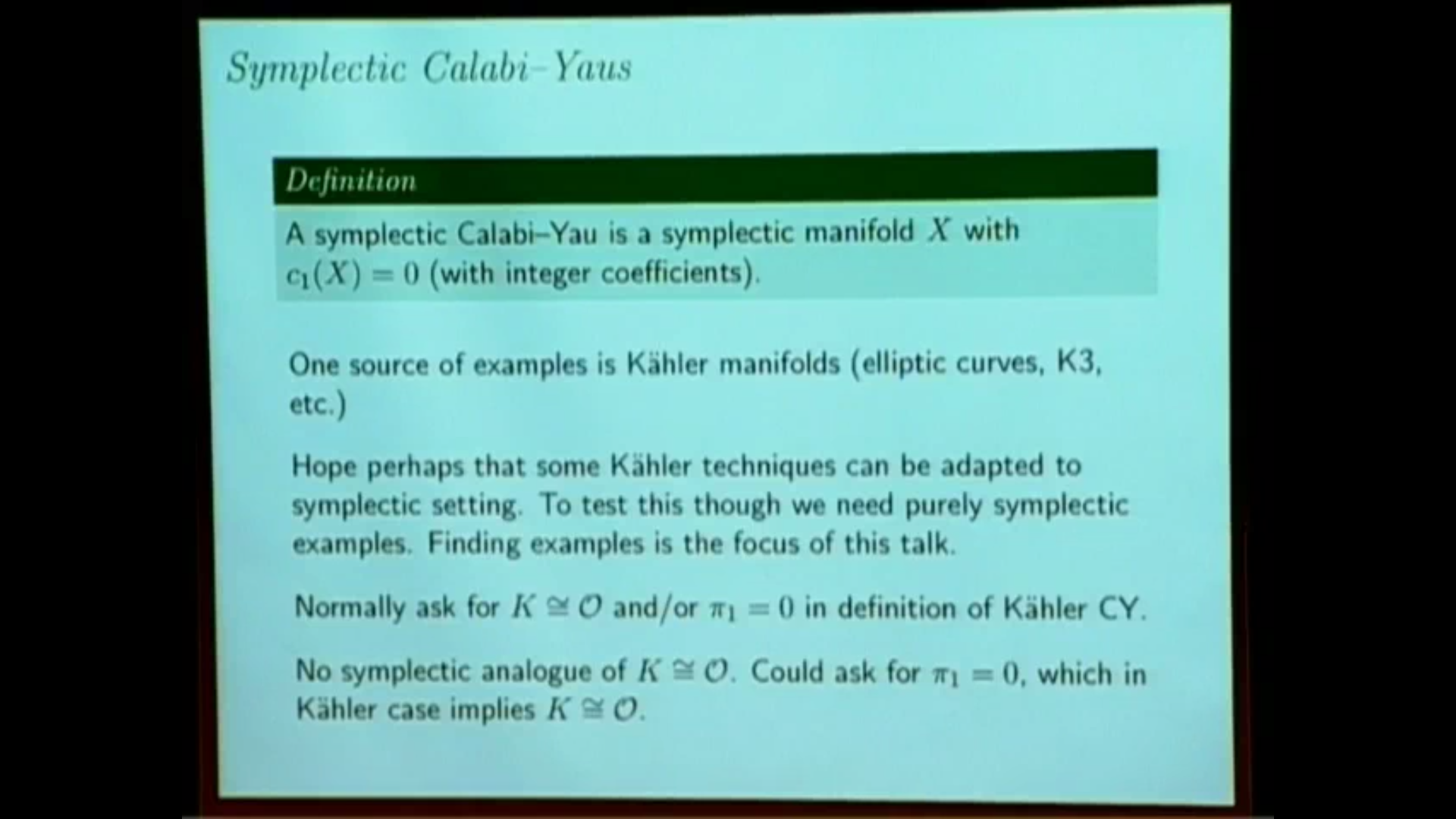
I will describe joint work with Dmitri Panov, in which we prove the following result. Given a finitely presented group G and an integer b there is a compact symplectic 6- manifold with c_1=0, b_2>b and fundamental group G. This is in sharp contrast to the situation for symplectic 4-manifolds, where the condition c_1=0 places strong restrictions on the topology, by a theorem of Tian-Jun Li. The proof is based on the fact that every hyperbolic 4-orbifold carries a fibration whose total space is a symplectic 6-orbifold with c_1=0. A construction of Panov and Petrunin provides a large collection of hyperbolic 4- orbifolds, with given fundamental group G. They are all built from a single simple piece, the right-angled hyperbolic 120 cell. The theorem is proved by making a crepant resolution of the singularities in the corresponding symplectic orbifolds.