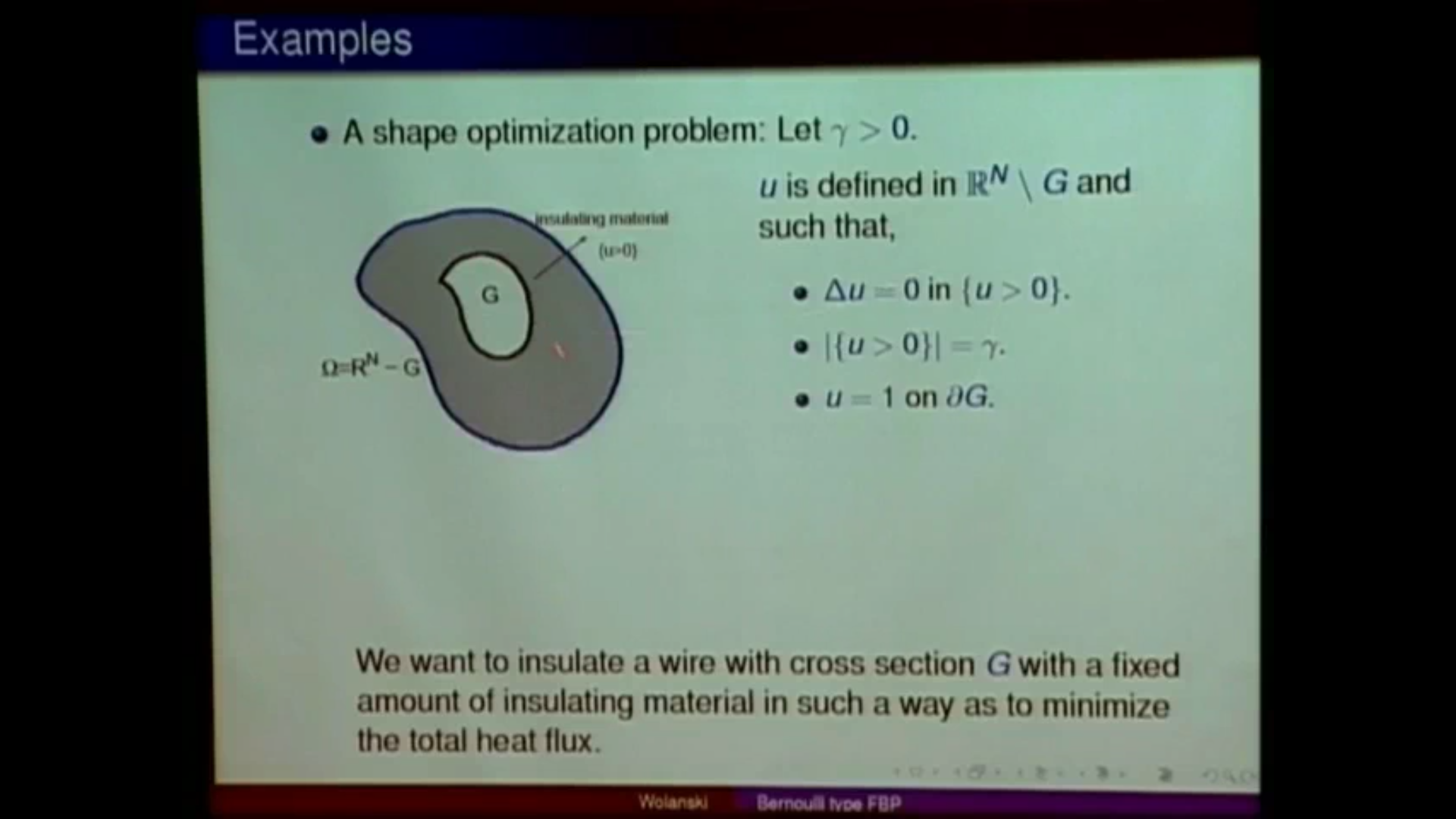
This is a survey talk in which we will describe what we understand by a Bernoulli type free boundary problem, we will present several optimization problems that lead to these kind of free boundary problems, and will describe the lines of proof of the regularity of the solution and of the free boundary. By a Bernoulli type free boundary problem we mean: given an open set Ω ⊂ R^N, an elliptic operator L defined on Ω and a nonnegative function phi defined on ∂Ω, find a subset Ω' ⊂ Ω and a function u ∈ C(Ω' closure) such that Lu=0 in Ω', u=phi on that part of the boundary of Ω' that lies on the boundary of Ω, u=0 on that part of the boundary of Ω' that lies inside Ω (called the free boundary) and such that, in addition, |∇u|=c on the free boundary with c a given constant (this is what is called the free boundary condition). We will survey on results where L is either uniformly or degenerate elliptic, linear or quasi-linear and with standard or nonstandard growth. This story started with the seminal paper of Alt and Caffarelli of 1981 for the Laplacian and ranges all the way up to the present days.